At a certain locatioa in Africa, compass point 12∘ west of geographic north. The north tip of magnetic needle of a dip circle placed in the plane of magnetic meridian points 60∘ above the horizontal. The horizontal component of the earth's field is measured to be 0.16(G). The magnitude of the earth's field (in gauss) at the location is
To find the magnitude of the Earth's magnetic field at the given location, we can use the relationship between the horizontal component of the magnetic field, the total magnetic field, and the angle of dip. Here’s a step-by-step solution:
Step 1: Understand the Components of the Magnetic Field
The Earth's magnetic field can be resolved into two components:
- The horizontal component (Bh)
- The vertical component (Bv)
The total magnetic field (B) can be calculated using the formula:
B=√B2h+B2v
Step 2: Identify the Given Values
From the problem, we have:
- Horizontal component, Bh=0.16G
- Angle of dip, θ=60∘
Step 3: Calculate the Vertical Component
The vertical component can be calculated using the angle of dip:
Bv=Bsin(θ)
We also know that:
Bh=Bcos(θ)
Step 4: Express B in Terms of Bh and θ
From the equation for the horizontal component:
Bh=Bcos(60∘)
Given that cos(60∘)=12, we can rewrite the equation as:
Bh=B2
Thus, we can express B as:
B=2Bh
Step 5: Substitute the Value of Bh
Substituting Bh=0.16G:
B=2×0.16G=0.32G
Step 6: Convert to Gauss
Since 1 Gauss is the unit we are using, our final answer remains:
B=0.32G
Step 7: Final Result
The magnitude of the Earth's magnetic field at the location is:
Magnitude of the Earth's magnetic field=0.32G
---
The Earth's magnetic field can be resolved into two components:
- The horizontal component (Bh)
- The vertical component (Bv)
B=√B2h+B2v
From the problem, we have:
- Horizontal component, Bh=0.16G
- Angle of dip, θ=60∘
The vertical component can be calculated using the angle of dip:
Bv=Bsin(θ)
Bh=Bcos(θ)
From the equation for the horizontal component:
Bh=Bcos(60∘)
Given that cos(60∘)=12, we can rewrite the equation as:
Bh=B2
Thus, we can express B as:
B=2Bh
Substituting Bh=0.16G:
B=2×0.16G=0.32G
Since 1 Gauss is the unit we are using, our final answer remains:
B=0.32G
The magnitude of the Earth's magnetic field at the location is:
Magnitude of the Earth's magnetic field=0.32G
Topper's Solved these Questions
Similar Questions
At a certain location in Africa, compass point 12∘ west of geographic north. The north tip of magnetic needle of a dip circle placed in the plane of magnetic meridian points 60∘ above the horizontal. The horizontal component of the earth's field is measured to be 0.16(G). The magnitude of the earth's field at the location is
View SolutionAt a certain location in Africa, a compass points 12∘ west of the geographic north. The north tip of the magnetic needle of a dip circle placed in the plane of magnetic meridian points 60∘ above the horizontal. The horizontal component of the earth's field is measured to be 0.16 G. Specify the direction and magnitude of the earth's field at the location.
View SolutionAt a certain location in Africa, compass points 12∘ west of geographic north, figure. The north tip of magnetic needle of a dip circle placed in the plane of magnetic meridian points 60∘ above the horizontal. The horizontal component of earth's field is measured to be 0.16gauss. Specify the direction and magnitude of the earth's field at the location.
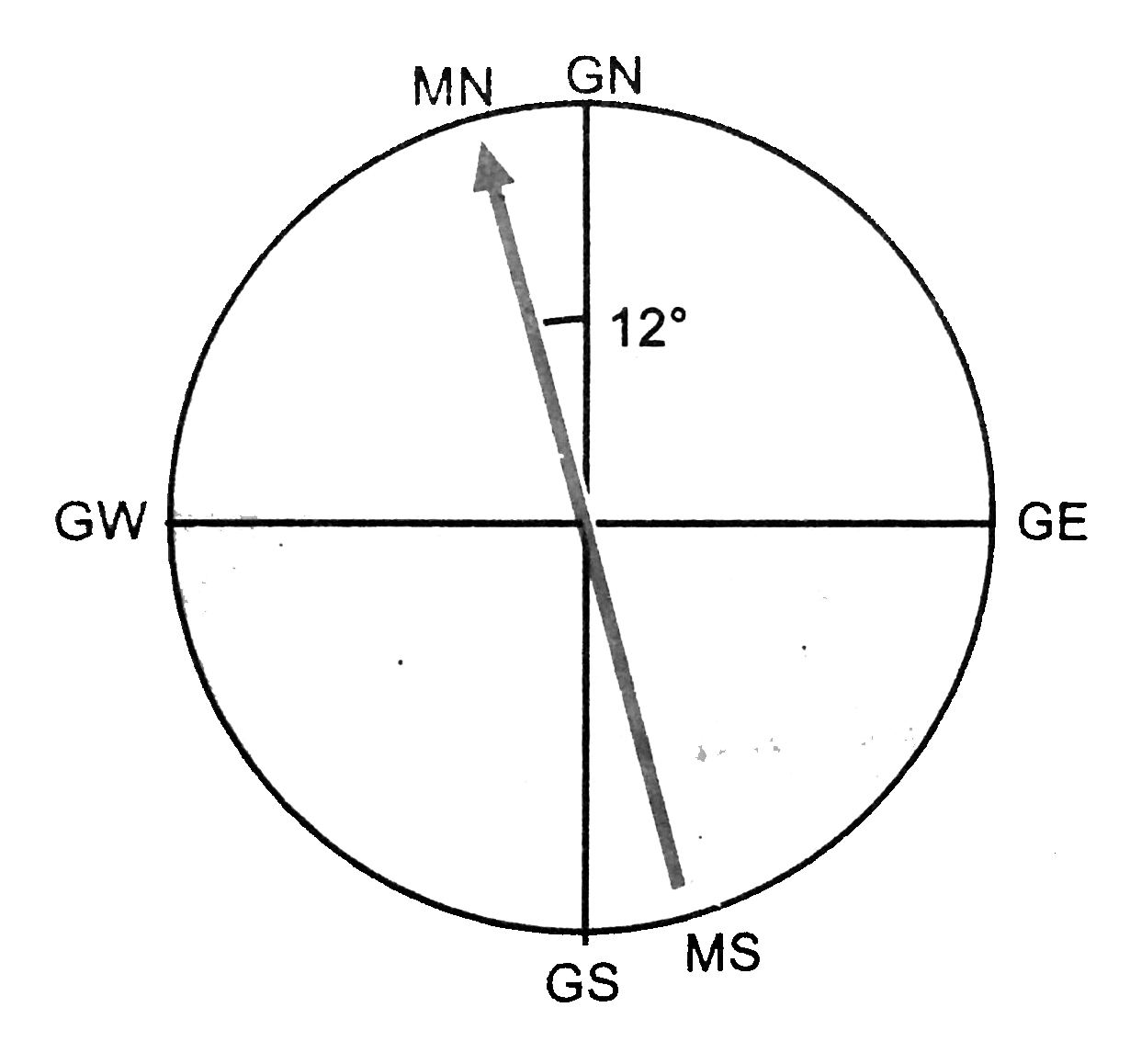
View SolutionA magnetic needle free to rotate in a vertical plane parallel to the magnetic meridian has its north tip down at 60∘ with the horizontal. The horizontal component of the earth's magnetic field at the place is known to be 0.4 G. Determine the magnitude of the earth's magnetic field at the place.
View SolutionA magnetic needle free to rotate in a vertical plane parallel to the magnetic meridian has its north tip down at 60∘ with the horizontal.The horizontal component of the earth's magnetic field at the place is known to be 0.4G.Determine the magnitude of the earth's magnetic field at the place.
View SolutionA magnetic needle free to rotate in a vertical plane parallel to magnetic meridian has its north tip down at 60∘ with the horizontal. The horizontal component of earth's magnetic field at that place is 0⋅4G. Determine the magnitude of earth's magnetic field at the place.
View SolutionThe horizontal component of the earth's magnetic field is 3.6×10−5T where the dip is 60∘. Find the magnitude of the earth's magnetic field.
View SolutionA magnetic needle free to rotate about the vertical direction (compass ) point 3.5 west of the geographic north . Another magnetic needle free to rotate in a vertical plane parallel to the magnetic meridian has its north tip pointing down at 18∘ with the horizontal . The magnitude of the horizontal component of the earth ' magnetic field at the place is known to be 0.40G . what is the direction and magnitude of the earth's magnetic field at the place ?
View SolutionA magnetic needle free to rotate in a vertical plane parallel to the magnetic meridian has its north tip pointing down at 22∘ with the horizontal. The horizontal component of the earth's magnetic field at the place is known to be 0.35 G. Determine the magnitude of the earth's magnetic field at the place.
View SolutionA magnetic needle free to rotate in a vertical plane parallel to the magnetic meridian has its north tip pointing down at 22∘ with the horizontal . The horizontal componemt of the earth's magnetic field at the place is know to be 0.35 G . Determine the magnitude of the earth's magnetic field at the place .
View Solution
