(c) Derive the expression for the affective capacitance of a series combination of n capacitors.
To derive the expression for the effective capacitance of a series combination of n capacitors, we can follow these steps:
1. Identify the Capacitors:
Let’s consider n capacitors connected in series, with capacitances C1,C2,C3,…,Cn.
2. Connect to a Voltage Source:
Connect these capacitors to a voltage source V. The total voltage V across the series combination will be distributed among the individual capacitors.
3. Charge on Capacitors:
In a series combination, the charge Q on each capacitor is the same. Therefore, we can denote the charge on each capacitor as Q.
4. Voltage Across Each Capacitor:
The voltage across each capacitor can be expressed using the formula V=QC:
- Voltage across C1: V1=QC1
- Voltage across C2: V2=QC2
- Voltage across C3: V3=QC3
- ...
- Voltage across Cn: Vn=QCn
5. Total Voltage:
The total voltage V across the series combination is the sum of the voltages across each capacitor:
V=V1+V2+V3+…+Vn
Substituting the expressions for Vi:
V=QC1+QC2+QC3+…+QCn
6. Factor Out Q:
We can factor out Q from the right-hand side:
V=Q(1C1+1C2+1C3+…+1Cn)
7. Expressing in Terms of Equivalent Capacitance:
The equivalent capacitance Ceq of the series combination can be defined as:
V=QCeq
Equating the two expressions for V:
QCeq=Q(1C1+1C2+1C3+…+1Cn)
8. Cancel Q:
Since Q is the same for all capacitors and is non-zero, we can cancel Q from both sides:
1Ceq=1C1+1C2+1C3+…+1Cn
9. Final Expression:
Thus, the effective capacitance Ceq for n capacitors in series is given by:
1Ceq=n∑i=11Ci
Let’s consider n capacitors connected in series, with capacitances C1,C2,C3,…,Cn.
Connect these capacitors to a voltage source V. The total voltage V across the series combination will be distributed among the individual capacitors.
In a series combination, the charge Q on each capacitor is the same. Therefore, we can denote the charge on each capacitor as Q.
The voltage across each capacitor can be expressed using the formula V=QC:
- Voltage across C1: V1=QC1
- Voltage across C2: V2=QC2
- Voltage across C3: V3=QC3
- ...
- Voltage across Cn: Vn=QCn
The total voltage V across the series combination is the sum of the voltages across each capacitor:
V=V1+V2+V3+…+Vn
Substituting the expressions for Vi:
V=QC1+QC2+QC3+…+QCn
We can factor out Q from the right-hand side:
V=Q(1C1+1C2+1C3+…+1Cn)
The equivalent capacitance Ceq of the series combination can be defined as:
V=QCeq
Equating the two expressions for V:
QCeq=Q(1C1+1C2+1C3+…+1Cn)
Since Q is the same for all capacitors and is non-zero, we can cancel Q from both sides:
1Ceq=1C1+1C2+1C3+…+1Cn
Thus, the effective capacitance Ceq for n capacitors in series is given by:
1Ceq=n∑i=11Ci
Topper's Solved these Questions
- XII BOARDSBook:XII BOARD PREVIOUS YEAR PAPER ENGLISHChapter:XII BOARDSExercise:SECTIONS-D
Explore 4 Videos
- XII BOARDSBook:XII BOARD PREVIOUS YEAR PAPER ENGLISHChapter:XII BOARDSExercise:C.B.S.E.CLASS-XII PHYSICS (THEORY) [SET-I]
Explore 3 Videos
- XII BOARDSBook:XII BOARD PREVIOUS YEAR PAPER ENGLISHChapter:XII BOARDSExercise:SECTIONS-C
Explore 8 Videos
- SAMPLE PAPER 2019Book:XII BOARD PREVIOUS YEAR PAPER ENGLISHChapter:SAMPLE PAPER 2019Exercise:SECTION D
Explore 6 Videos
Similar Questions
(i) Define the SI unit of capacitance.
(ii) Obtain expression for the capacitance of a parallel plate capacitor.
(iii) Derive the expression for the effective capacitance of a parallel combination of 2 capacitors.
View SolutionObtain an expression for effective capacitance of two capacitors in series.
View Solution(b) Obtain the expression for the capacitance of a parallel plate capacitor.
View SolutionBriefly explain the principle of a capacitor. Derive an expression for the capacitance of a parallel plate capacitor, whose plates are separated by a dielectric medium.
View SolutionWrite the expression for equivalent spring constant of
(i) parallel combination of n−springs
(ii) series combination of n−springs
View SolutionThe minimum value of effective capacitance that can be obtained by combining 3 capacitors of capacitances 1 pF, 2pF and 4pF is
View SolutionAnswer the following:
(a) Describe briefly the process of transferring charge between the two plates of a parallel plate capacitor when connected to a battery. Derive an expression for the energy stored in a capacitor.
(b) A parallel plate capacitor is connected to a battery of potential difference V. It is disconnected from battery and then connected to another uncharged capacitor of the same capacitance. Calculate the ratio of the energy stored in the combination to the initial energy on the single capacitor.
View SolutionThree capacitor of capacitance C(μF) are connected in parallel to which a capacitor of capacitance C is connected in series. Effective capacitance is 3.75. then capacity off each capacitor is
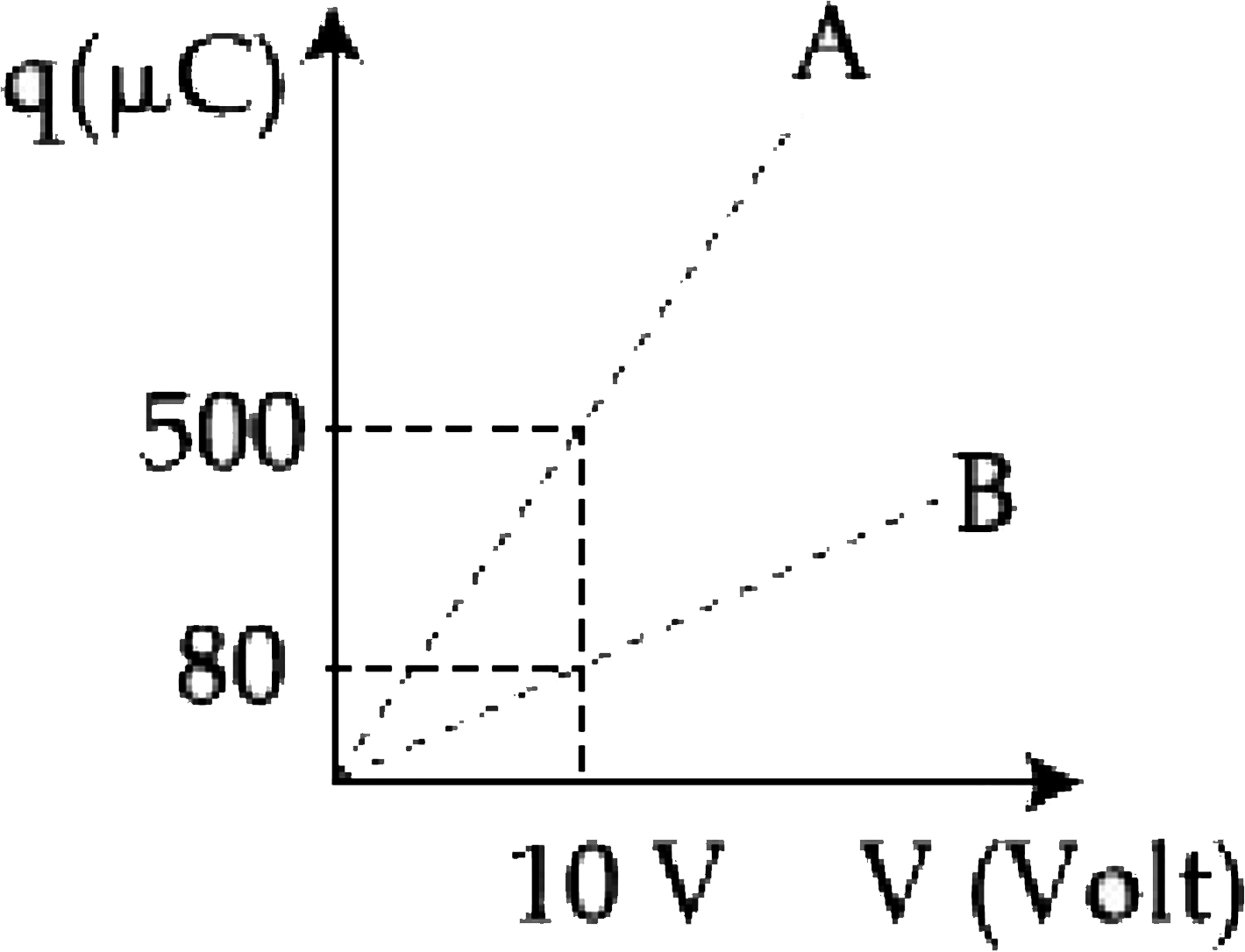
View SolutionFigure shows charge (q) versus voltage (V) graph for series and parallel combination of two given capacitors.The capacitances are :
View SolutionA 200Ω resistor is connected in series with a 5(μ)F capacitor. The voltage across the resistor is VR=(1.20V)cos(2500rads−1)t.
(a) Derive an expression for the circuit current.
(b) Determine the capacitive reactance of the capacitor.
View Solution