Consider a particle of mass m having linear momentum →p at position →r relative to the origin O. Let →L be the angular momentum of the particle with respect to the origin. Which of the following equations correctly relate(s) →r, →p and →L?
d→Ldt+→r×d→pdt=0
d→Ldt+d→rdt×→p=0
d→Ldt−d→rdt×→p=0
d→Ldt−→r×d→pdt=0
The correct Answer is:D
To solve the problem, we need to establish the relationship between the position vector →r, linear momentum vector →p, and angular momentum vector →L of a particle of mass m.
1. Understanding Angular Momentum:
The angular momentum →L of a particle with respect to a point (in this case, the origin O) is defined as:
→L=→r×→p
where →r is the position vector of the particle relative to the origin O, and →p is the linear momentum of the particle.
2. Differentiating Angular Momentum:
To find a relationship involving time, we differentiate both sides of the angular momentum equation with respect to time t:
d→Ldt=ddt(→r×→p)
3. Applying the Product Rule:
Using the product rule for differentiation of the cross product, we have:
d→Ldt=d→rdt×→p+→r×d→pdt
4. Substituting Linear Momentum:
Since the linear momentum →p is defined as →p=m→v (where →v=d→rdt), we can express d→pdt as:
d→pdt=md→vdt=m→a
where →a is the acceleration of the particle.
5. Analyzing the Terms:
The first term d→rdt×→p becomes →v×→p. However, since →p=m→v, we find that:
→v×→p=→v×(m→v)=0
because the cross product of any vector with itself is zero.
6. Final Equation:
Thus, we can simplify our earlier equation:
d→Ldt=0+→r×d→pdt
or,
d→Ldt=→r×d→pdt
7. Setting Up the Final Relation:
Rearranging gives us:
d→Ldt−→r×d→pdt=0
Conclusion:
The correct relationship that relates →r, →p, and →L is:
d→Ldt−→r×d→pdt=0
Thus, the answer is option D.
The angular momentum →L of a particle with respect to a point (in this case, the origin O) is defined as:
→L=→r×→p
where →r is the position vector of the particle relative to the origin O, and →p is the linear momentum of the particle.
To find a relationship involving time, we differentiate both sides of the angular momentum equation with respect to time t:
d→Ldt=ddt(→r×→p)
Using the product rule for differentiation of the cross product, we have:
d→Ldt=d→rdt×→p+→r×d→pdt
Since the linear momentum →p is defined as →p=m→v (where →v=d→rdt), we can express d→pdt as:
d→pdt=md→vdt=m→a
where →a is the acceleration of the particle.
The first term d→rdt×→p becomes →v×→p. However, since →p=m→v, we find that:
→v×→p=→v×(m→v)=0
because the cross product of any vector with itself is zero.
Thus, we can simplify our earlier equation:
d→Ldt=0+→r×d→pdt
or,
d→Ldt=→r×d→pdt
Rearranging gives us:
d→Ldt−→r×d→pdt=0
The correct relationship that relates →r, →p, and →L is:
d→Ldt−→r×d→pdt=0
Thus, the answer is option D.
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONSBOOK - NCERT FINGERTIPSCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONSEXERCISE - Equilibrium Of A Rigid Body
13 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONSBOOK - NCERT FINGERTIPSCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONSEXERCISE - Moment Of Inertia
7 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONSBOOK - NCERT FINGERTIPSCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONSEXERCISE - Motion Of Centre Of Mass
10 Videos
- PRACTICE PAPERSBOOK - NCERT FINGERTIPSCHAPTER - PRACTICE PAPERSEXERCISE - Practice Paper 3
50 Videos
- THERMAL PROPERTIES OF MATTERBOOK - NCERT FINGERTIPSCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Assertion And Reason
10 Videos
Similar Questions
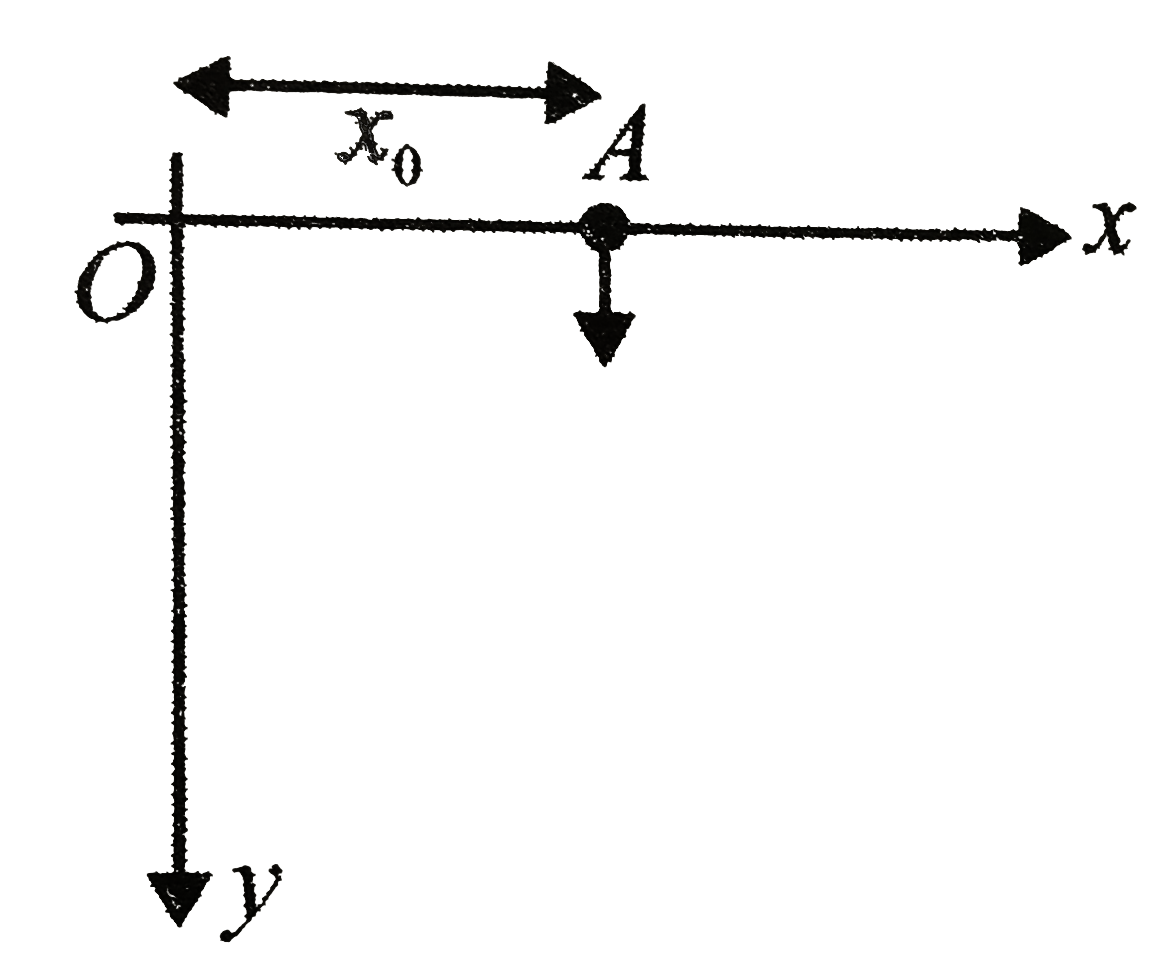
A particle of mass m is released from rest at point A in Fig., falling parallel to the (vertical) y-axis. Find the angular momentum of the particle at any time t with respect to the same origin O.
View SolutionA particle moves with a constant velocity pralel to the X-axis. Its angular momentum with respect to the origin
View Solution
Knowledge Check
If a moving particle have linear momentum →P and position vector →r then choose the correct relation between →→p and angular momentum →L of particle about the origin.
A→r.→L=0B∣∣→r.→L∣∣=0C→L.→P≠0D∣∣→L.→P∣∣=0A particle of mass m moves in the XY plane with a velocity v along the straight line AB. If the angular momentum of the particle with respect to origin O is LA when it is at A and LB when it is at B, then
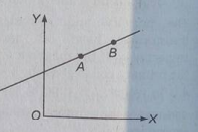 ALA>LBBLA=LBCthe relationship between LA and LBdepends upon the slope of the line ABDLA<LB
ALA>LBBLA=LBCthe relationship between LA and LBdepends upon the slope of the line ABDLA<LBA particle of mass m moves in the XY plane with a velocity v along the straight line AB. If the angular momentum of the particle with respect to origin O is LA when it is at A and LB when it is at B, then
 ALA>LBBLA=LBCthe relationship between LA and LBdepends upon the slope of the line ABDLA<LB
ALA>LBBLA=LBCthe relationship between LA and LBdepends upon the slope of the line ABDLA<LB
A particle moves with a constant velocity parallel to the X-axis. Its angular momentum with respect to the origin
View SolutionA particle of mass m=5kg is moving with a uniform speed v=3√2 in the XOY plane along the line Y=X+4. The magnitude of the angular momentum of the particle about the origin is
View SolutionA particle has a linear momentum p and position vector r. the angular momentum of this particle about the origin will not be zero under the conditions
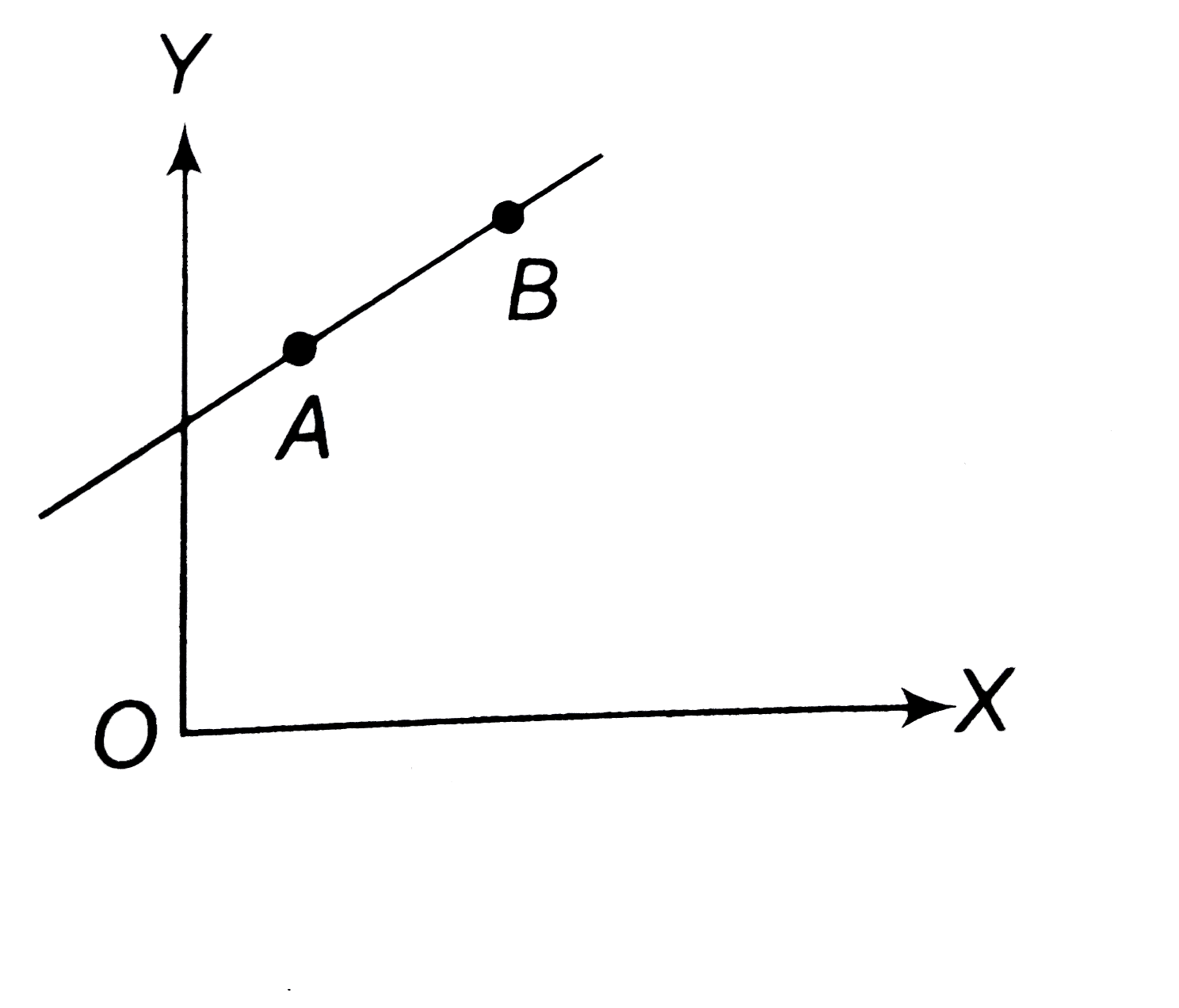
View SolutionA particle of mass m moves in the XY plane with a velocity v along the straight line AB. If the angular momentum of the particle with respect to origin O is LA when it is at A and LB when it is at B, then
View SolutionA particle of mass m=5 unit is moving with a uniform speed v=3√2 unit in the XOY plane along the line y=x+4 the magnitude of the angular momentum of the particle about the origin is
View Solution