Consider a radioactive disintegration according to the equation A→B→C. Decay constant of A and B is same and equal to λ. Number of nuclei of A, B and C are N0 , 0, 0 respectively at t=0. Find
(a) number of nuclei of B as function of time t.
(b) time t at which the activity of B is maximum and the value of maximum activity of B.
The correct Answer is:A, B, D
To solve the problem step-by-step, we will analyze the radioactive disintegration process and derive the required expressions.
Step 1: Write the Rate Equations
For the disintegration process A→B→C, we can write the rate equations for the number of nuclei of A, B, and C.
1. For nucleus A:
dNAdt=−λNA
2. For nucleus B:
dNBdt=λNA−λNB
3. For nucleus C:
dNCdt=λNB
Step 2: Solve for NA(t)
We start with the equation for NA:
dNAdt=−λNA
Integrating this from t=0 to t:
NA(t)=N0e−λt
Step 3: Substitute NA(t) into the Equation for NB
Now we substitute NA(t) into the equation for NB:
dNBdt=λNA−λNB
Substituting NA(t):
dNBdt=λN0e−λt−λNB
Step 4: Rearranging the Equation for NB
Rearranging gives:
dNBdt+λNB=λN0e−λt
This is a first-order linear differential equation.
Step 5: Solve the Differential Equation for NB
To solve it, we can use an integrating factor:
The integrating factor is eλt. Multiplying through by this factor:
eλtdNBdt+λeλtNB=λN0
The left-hand side can be rewritten as:
ddt(NBeλt)=λN0
Integrating both sides:
NBeλt=λN0t+C
At t=0, NB(0)=0, so C=0:
NBeλt=λN0t
Thus,
NB(t)=λN0te−λt
Step 6: Find the Maximum Activity of B
The activity RB of B is given by:
RB=λNB=λ2N0te−λt
Step 7: Maximize the Activity
To find the time t at which RB is maximum, we differentiate:
dRBdt=λ2N0e−λt(1−λt)=0
Setting the derivative to zero gives:
1−λt=0⟹t=1λ
Step 8: Calculate Maximum Activity
Substituting t=1λ into the expression for RB:
RB(1λ)=λ2N0(1λ)e−λ⋅1λ=λN0e−1
Final Answers
(a) The number of nuclei of B as a function of time t:
NB(t)=λN0te−λt
(b) The time t at which the activity of B is maximum is:
t=1λ
And the value of maximum activity of B is:
RB(1λ)=λN0e
For the disintegration process A→B→C, we can write the rate equations for the number of nuclei of A, B, and C.
dNAdt=−λNA
2. For nucleus B:
dNBdt=λNA−λNB
dNCdt=λNB
We start with the equation for NA:
dNAdt=−λNA
Integrating this from t=0 to t:
NA(t)=N0e−λt
Now we substitute NA(t) into the equation for NB:
dNBdt=λNA−λNB
Substituting NA(t):
dNBdt=λN0e−λt−λNB
Rearranging gives:
dNBdt+λNB=λN0e−λt
This is a first-order linear differential equation.
To solve it, we can use an integrating factor:
The integrating factor is eλt. Multiplying through by this factor:
eλtdNBdt+λeλtNB=λN0
The left-hand side can be rewritten as:
ddt(NBeλt)=λN0
Integrating both sides:
NBeλt=λN0t+C
At t=0, NB(0)=0, so C=0:
NBeλt=λN0t
Thus,
NB(t)=λN0te−λt
The activity RB of B is given by:
RB=λNB=λ2N0te−λt
To find the time t at which RB is maximum, we differentiate:
dRBdt=λ2N0e−λt(1−λt)=0
Setting the derivative to zero gives:
1−λt=0⟹t=1λ
Substituting t=1λ into the expression for RB:
RB(1λ)=λ2N0(1λ)e−λ⋅1λ=λN0e−1
(a) The number of nuclei of B as a function of time t:
NB(t)=λN0te−λt
t=1λ
And the value of maximum activity of B is:
RB(1λ)=λN0e
Topper's Solved these Questions
- MODERN PHYSICS - 2BOOK - DC PANDEY ENGLISHCHAPTER - MODERN PHYSICS - 2EXERCISE - Level 2 Comprehension Based
3 Videos
- MODERN PHYSICS - 1BOOK - DC PANDEY ENGLISHCHAPTER - MODERN PHYSICS - 1EXERCISE - Level 2 Subjective
23 Videos
- NUCLEIBOOK - DC PANDEY ENGLISHCHAPTER - NUCLEIEXERCISE - C MADICAL ENTRANCES GALLERY
46 Videos
Similar Questions
Two radioactive materials A & B have decay constant 3λ and 2λ respectively. At t=0 the numbers of nuclei of A and B are 4N0 and 2N0 respectively then,
View SolutionNumber of nuclei of a radioactive substance are 1000 and 900 at times t=0 and time t=2s. Then, number of nuclei at time t=4s will be
View Solution
Knowledge Check
The decay constant of a radioactive isotope is λ. If A1 and A2 are its activites at time t1 and t2 respectively, then the number of nuclei which have decayed the time (t1−t2)
AA1t1−A2t2BA1−A2C(A1−A2)/λDλ(A1−A2)
At time t=0 , number of nuclei of a radioactive substance are 100. At t=1 s these numbers become 90. Find the number of nuclei at t=2s.
View SolutionAt time t=0 , number of nuclei of a radioactive substance are 100. At t=1 s these numbers become 90. Find the number of nuclei at t=2s.
View SolutionNucleus A decays into B with a decay constant λ1 and B further decays into C with a decay constant λ2 . Initially, at t = 0, the number of nuclei of A and B were 3N0 and N0 respectively. If at t = t0 number of nuclei of B becomes constant and equal to 2N0, then
View SolutionA radio nuclide X is produced at constant rate α. At time t=0, number of nuclei of X are zero. Find
(a) the maximum number of nuclei of X.
the number of nuclei at time t.
Decay constant of X is λ.
View SolutionTwo radioactive samples of different elements (half-lives t1 and t2 respectively) have same number of nuclei at t=0. The time after which their activities are same is
View SolutionNucleus A decays to B with decay constant λ1 and B decays to C with decay constant λ2. Initially at t=0 number of nuclei of A and B are 2N0 and N0 respectively. At t=to, no. of nuclei of B is 3N02 and nuclei of B stop changing. Find t0?
View SolutionConsider radioactive decay of A to B with which further decays either to X or Y, λ1,λ2 and λ3 are decay constant for A to B decay, B to X decay and Bto Y decay respectively. At t=0, the number of nuclei of A,B,X and Y are N0,N0 zero and zero respectively.
N1,N2,N3 and N4 are the number of nuclei of A,B,X and Y at any instant t.
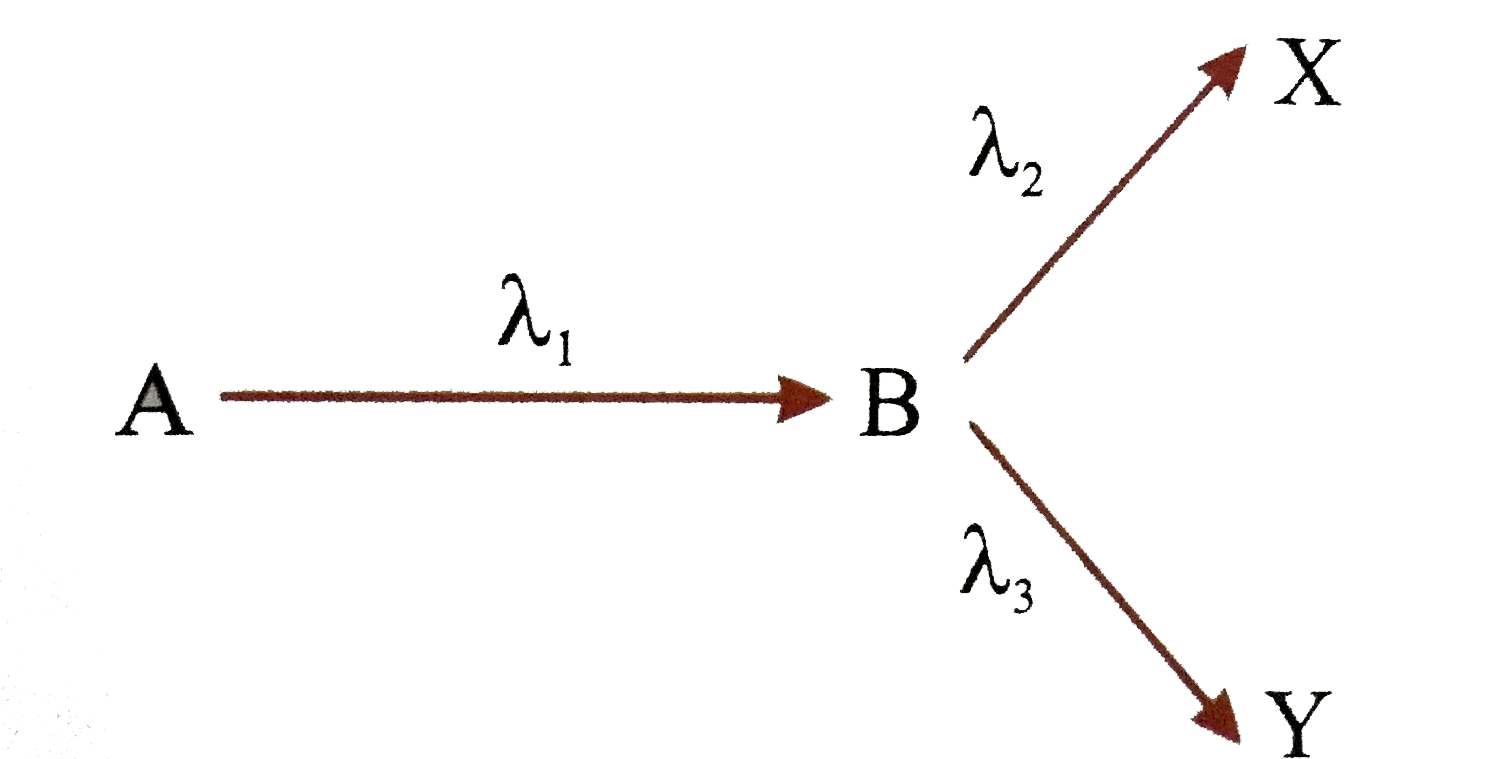
The number of nuclei of B will first increase and then after a maximum value, it decreases for
View Solution
