Nucleus A decays into B with a decay constant λ1 and B further decays into C with a decay constant λ2 . Initially, at t = 0, the number of nuclei of A and B were 3N0 and N0 respectively. If at t = t0 number of nuclei of B becomes constant and equal to 2N0, then
t0=1λ1ln[3λ12λ2]
t0=1λ1lm[λ1λ2]
t0=1λ1ln[2λ13λ2]
t0=1λ2ln[3λ12λ2]
The correct Answer is:A
To solve the problem step by step, we need to analyze the decay process of nuclei A and B, and how they relate to each other through their decay constants.
1. Understand the Initial Conditions:
- At time t=0:
- Number of nuclei of A, NA(0)=3N0
- Number of nuclei of B, NB(0)=N0
2. Write the Decay Equations:
- The decay of A into B can be expressed as:
dNAdt=−λ1NA
- The decay of B into C can be expressed as:
dNBdt=λ1NA−λ2NB
3. Solve for NA(t):
- The solution to the decay equation for A is:
NA(t)=NA(0)e−λ1t=3N0e−λ1t
4. Substitute NA(t) into the equation for NB(t):
- Now substituting NA(t) into the equation for NB:
dNBdt=λ1(3N0e−λ1t)−λ2NB
5. At t=t0, we know that NB becomes constant and equals 2N0:
- This means dNBdt=0 at t=t0:
0=λ1(3N0e−λ1t0)−λ2(2N0)
6. Rearranging the equation:
- Rearranging gives:
λ1(3N0e−λ1t0)=2λ2N0
- Dividing by N0:
3λ1e−λ1t0=2λ2
7. Solving for e−λ1t0:
- Rearranging gives:
e−λ1t0=2λ23λ1
8. Taking the natural logarithm:
- Taking the natural logarithm of both sides:
−λ1t0=ln(2λ23λ1)
- Thus:
t0=−1λ1ln(2λ23λ1)
9. Final Expression:
- The expression for t0 can be simplified as:
t0=1λ1ln(3λ12λ2)
- At time t=0:
- Number of nuclei of A, NA(0)=3N0
- Number of nuclei of B, NB(0)=N0
- The decay of A into B can be expressed as:
dNAdt=−λ1NA
- The decay of B into C can be expressed as:
dNBdt=λ1NA−λ2NB
- The solution to the decay equation for A is:
NA(t)=NA(0)e−λ1t=3N0e−λ1t
- Now substituting NA(t) into the equation for NB:
dNBdt=λ1(3N0e−λ1t)−λ2NB
- This means dNBdt=0 at t=t0:
0=λ1(3N0e−λ1t0)−λ2(2N0)
- Rearranging gives:
λ1(3N0e−λ1t0)=2λ2N0
- Dividing by N0:
3λ1e−λ1t0=2λ2
- Rearranging gives:
e−λ1t0=2λ23λ1
- Taking the natural logarithm of both sides:
−λ1t0=ln(2λ23λ1)
- Thus:
t0=−1λ1ln(2λ23λ1)
- The expression for t0 can be simplified as:
t0=1λ1ln(3λ12λ2)
Similar Questions
Nucleus A decays to B with decay constant λ1 and B decays to C with decay constant λ2. Initially at t=0 number of nuclei of A and B are 2N0 and N0 respectively. At t=to, no. of nuclei of B is 3N02 and nuclei of B stop changing. Find t0?
View SolutionTwo radioactive materials A & B have decay constant 3λ and 2λ respectively. At t=0 the numbers of nuclei of A and B are 4N0 and 2N0 respectively then,
View SolutionNucleus A decays into B with a decay constant λ1 and B further decays into C with a decay constant λ2 . Initially, at t = 0, the number of nuclei of A and B were 3N0 and N0 respectively. If at t = t0 number of nuclei of B becomes constant and equal to 2N0, then
View SolutionA radioactive substance A having N0 active nuclei at t=0, decays to another radioactive substance B with decay constant λ1. B further decays to a stable substance C with decay constant λ2. (a) Find the number of nuclei of A,B and C time t. (b) What should be the answer of part (a) if λ1>>λ2 and λ1<<λ2
View SolutionA radioactive nucleus X decays to a nucleus Y with a decay constant λX=0.1s−1, Y further decays to a stable nucleus Z with a decay constant
λY=1/30s−1. Initially, there are only X nuclei and their number is N0=1020. Set up the rate equations for the populations of X, Y and Z. The population of Y nucleus as a function of time is given by NY(t)={N0λX/(λX−λY)}[exp(−λYt)−exp(−λXt)].
Find the time at which NY is maximum and determine the population X and Z at that instant.
View SolutionConsider radioactive decay of A to B with which further decays either to X or Y, λ1,λ2 and λ3 are decay constant for A to B decay, B to X decay and Bto Y decay respectively. At t=0, the number of nuclei of A,B,X and Y are N0,N0 zero and zero respectively.
N1,N2,N3 and N4 are the number of nuclei of A,B,X and Y at any instant t.
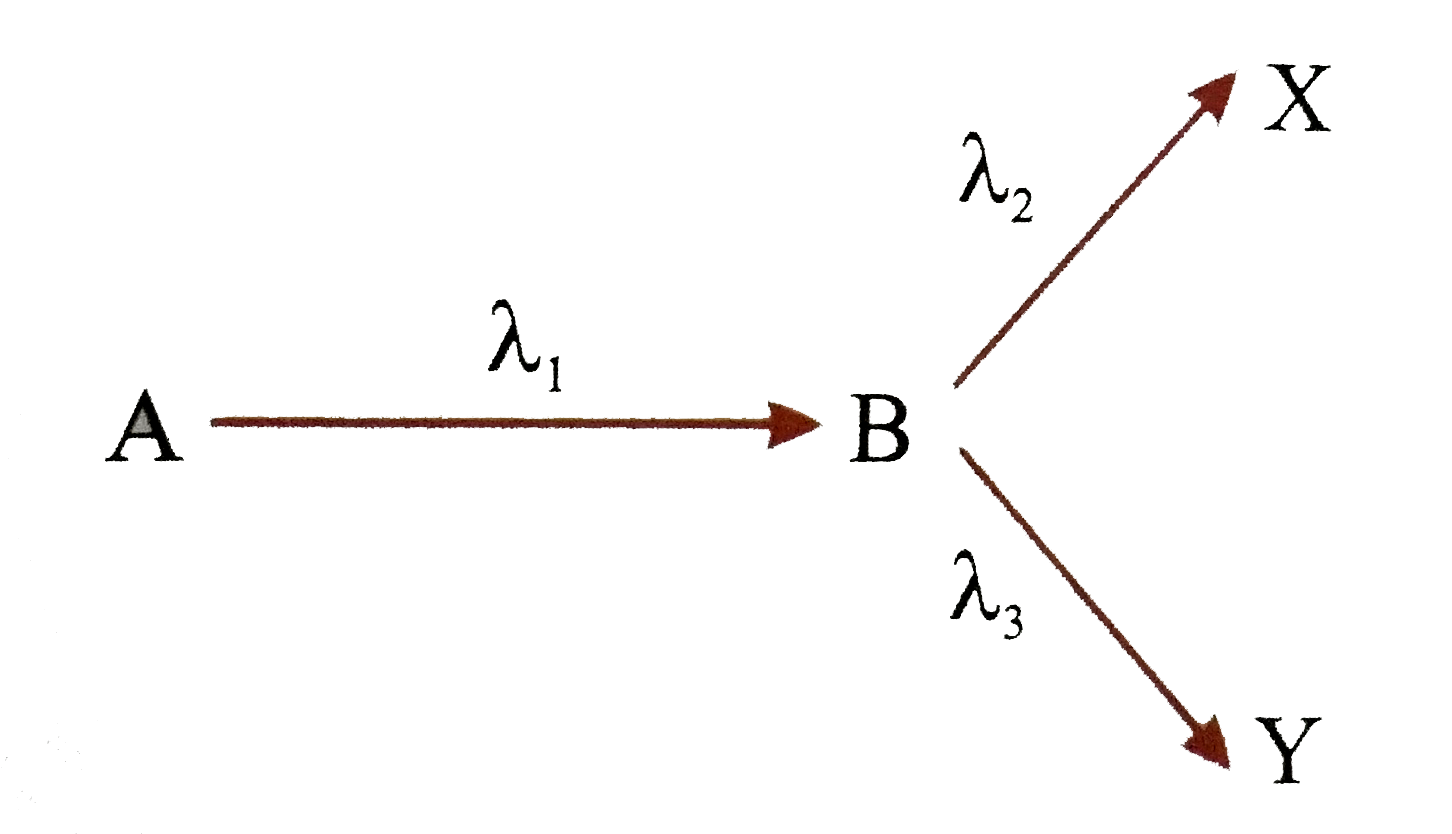
The net rate of accumulation of B at any instant is
View SolutionConsider radioactive decay of A to B with which further decays either to X or Y, λ1,λ2 and λ3 are decay constant for A to B decay, B to X decay and Bto Y decay respectively. At t=0, the number of nuclei of A,B,X and Y are N0,N0 zero and zero respectively.
N1,N2,N3 and N4 are the number of nuclei of A,B,X and Y at any instant t.
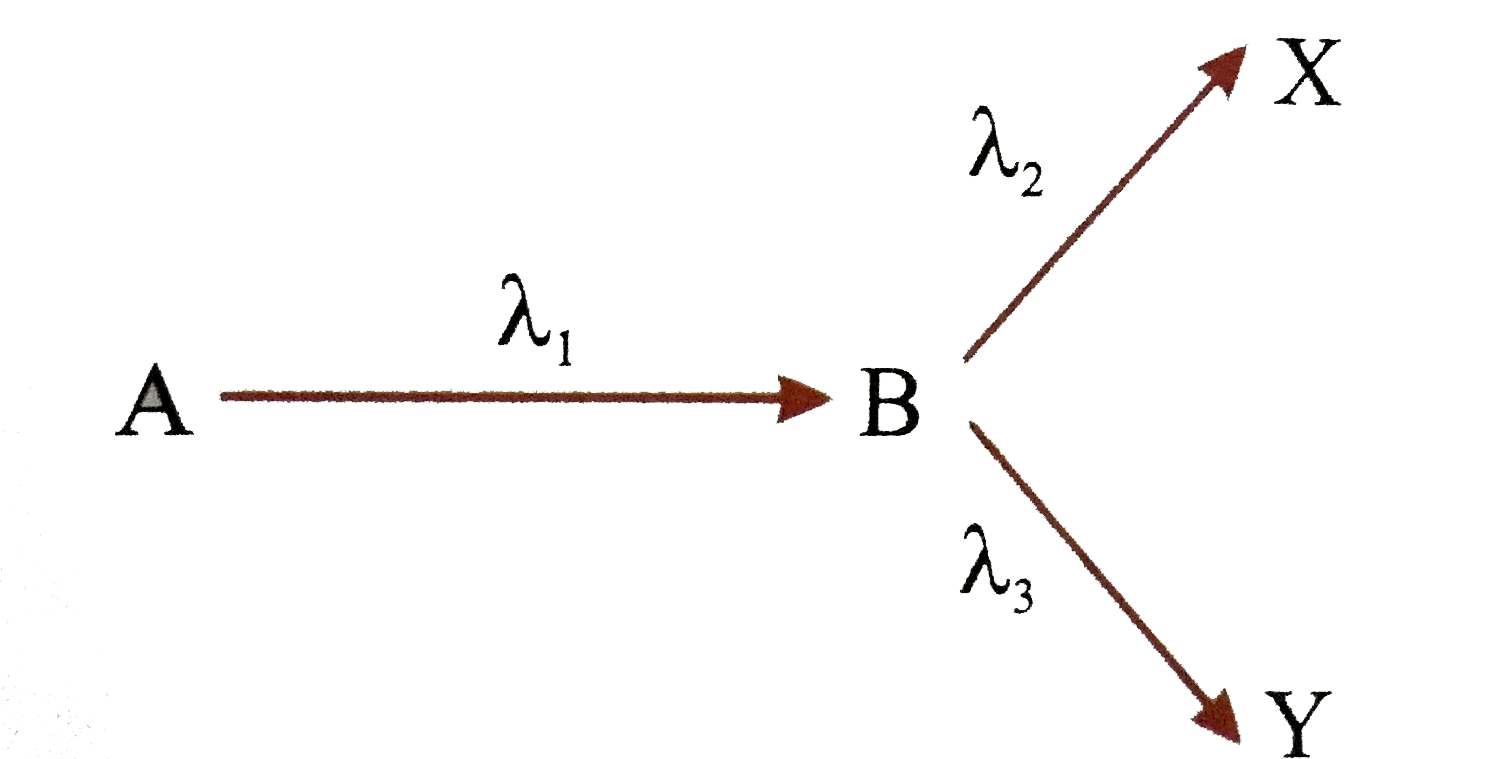
The number of nuclei of B will first increase and then after a maximum value, it decreases for
View SolutionA parent radioactive nucleus A (decay constant λA) converts into a radio-active nucleus B of decay constant λb, initially, number of atoms of B is zero At any time Na,Nb are number of atoms of nuclei A and B respectively then maximum velue of Nb.
View SolutionAt time t=0N1 nuclei of decay constant λ1&N2 nuclei of decay constant λ2 are mixed. The decay rate of the mixture at time 't' is:
View SolutionRadioactive material 'A' has decay constant '8λ' and material 'B' has decay constant 'lamda'. Initial they have same number of nuclei. After what time, the ratio of number of nuclei of material 'A' to that 'B' will be '(1/ e) ?
View Solution
