Consider a uniform square plate of side 'a' and mass 'm' The moment of inertia of heis plate about an axis perpendiucalar to its plane and passing through one of its corners is
(5)(6)ma2
12ma2
712ma2
23ma2
The correct Answer is:D
To find the moment of inertia of a uniform square plate of side 'a' and mass 'm' about an axis perpendicular to its plane and passing through one of its corners, we can follow these steps:
Step 1: Moment of Inertia about the Center
First, we need to calculate the moment of inertia of the square plate about an axis passing through its center. The formula for the moment of inertia ICM of a square plate about an axis perpendicular to its plane and passing through its center is given by:
ICM=16ma2
Step 2: Finding the Distance to the Corner
Next, we need to find the distance from the center of the plate to the corner where the axis is located. The distance from the center of the square to a corner can be calculated as follows:
The diagonal of the square is given by:
d=a√2
Since we need the distance from the center to the corner, we take half of the diagonal:
Distance=d2=a√22
Step 3: Applying the Parallel Axis Theorem
Now we can apply the Parallel Axis Theorem, which states that:
I=ICM+md2
where d is the distance from the center of mass to the new axis. Substituting the values we have:
I=16ma2+m(a√22)2
Calculating (a√22)2:
(a√22)2=2a24=a22
Now substituting this back into the equation:
I=16ma2+m⋅a22
Step 4: Simplifying the Expression
Now we combine the terms:
I=16ma2+36ma2=46ma2=23ma2
Final Result
Thus, the moment of inertia of the square plate about the axis passing through one of its corners is:
I=23ma2
First, we need to calculate the moment of inertia of the square plate about an axis passing through its center. The formula for the moment of inertia ICM of a square plate about an axis perpendicular to its plane and passing through its center is given by:
Next, we need to find the distance from the center of the plate to the corner where the axis is located. The distance from the center of the square to a corner can be calculated as follows:
Now we can apply the Parallel Axis Theorem, which states that:
Now we combine the terms:
Thus, the moment of inertia of the square plate about the axis passing through one of its corners is:
Topper's Solved these Questions
- BOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - ROTATIONAL MOTIONEXERCISE - MCQs with one correct answer1videos
ROTATIONAL MOTION
- BOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - MOTIONEXERCISE - JEE Main And Advanced63videos
MOTION
- BOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - JEE Main And Advanced69videos
SIMPLE HARMONIC MOTION
Similar Questions
Calculate the moment of inertia of a rod of mass M, and length l about an axis perpendicular to it passing through one of its ends.
View SolutionFind the moment of inertia of a uniform half-disc about an axis perpendicular to the plane and passing through its centre of mass. Mass of this disc is M and radius is R.
View Solution
Knowledge Check
- Question 1 - Select One
Consider a uniform square plate of side 'a' and mass 'm'. The moment of inertia of this plate about an axis perpendicular to its plane and passing through one of its corners is
A112ma2B712ma2C23ma2D56ma2 - Question 2 - Select One
Consider a uniform square plate of of side and mass m. The moment of inertia of this plate about an axis perpendicular to its plane and passing through one of its corners is -
A56ma2B112ma2C712ma2D23ma2 - Question 3 - Select One
If I moment of inertia of a thin circular plate about an axis passing through tangent of plate in its plane. The moment of inertia of same circular plate about an axis perpendicular to its plane and passing through its centre is
A4I5B2I5C4I3D2I3
Moment of inertia of a uniform circular disc about a diameter is I. Its moment of inertia about an axis perpendicular to its plane and passing through a point on its rim will be.
View SolutionFour particles each of mass m are placed at the corners of a square of side length l. The radius of gyration of the system about an axis perpendicular to the plane of square and passing through its centre is
View SolutionA square is made by joining four rods each of mass M and length L. Its moment of inertia about an axis PQ, in its plane and passing through one of its corner is
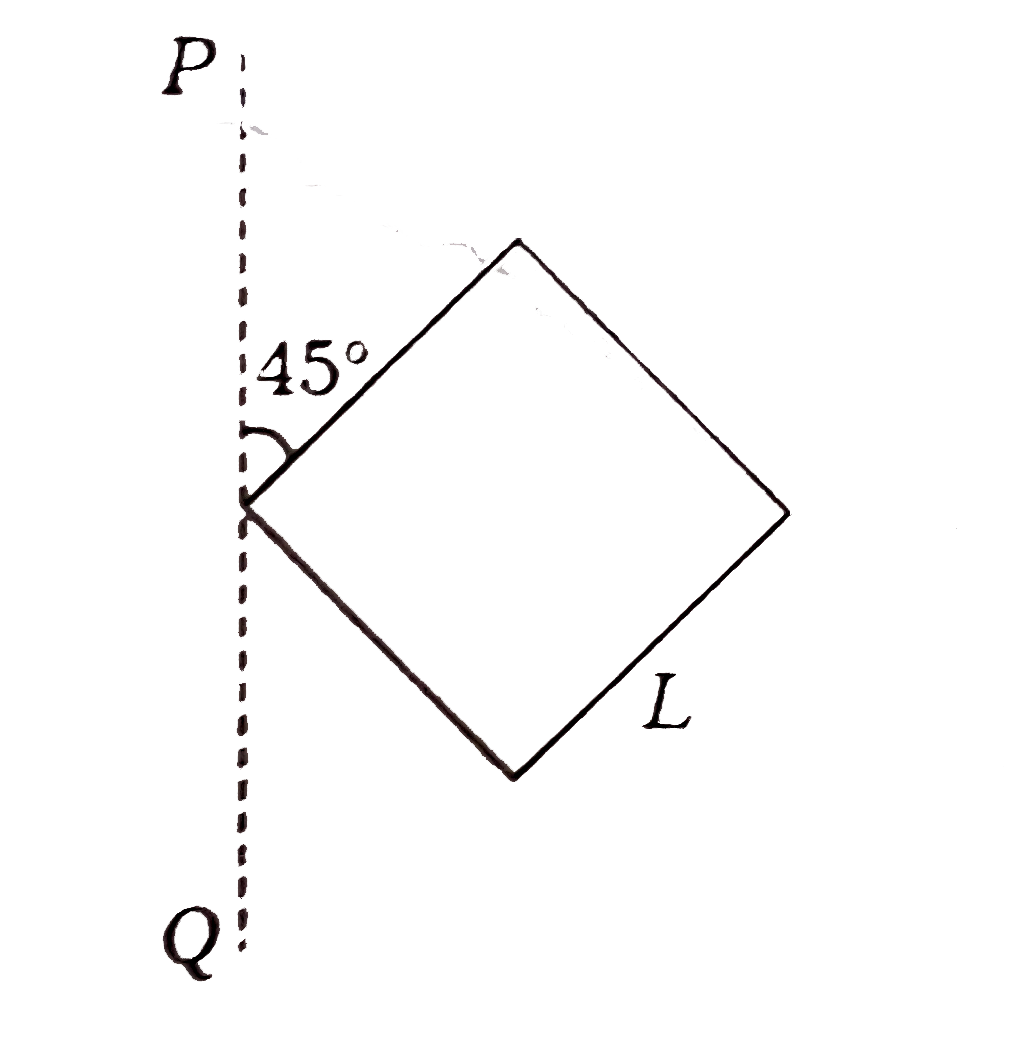 View Solution
View SolutionI is moment of inertia of a thin square plate about an axis passing through opposite corners of plate. The moment of inertia of same plate about an axis perpendicular to the plane of plate and passing through its centre is
View SolutionA square plate of mass M and edge L is shown in the figure. The moment of inertia of the plate about the axis in the plane of plate and passing through one of its vertex making an angle 15∘ horizontal is
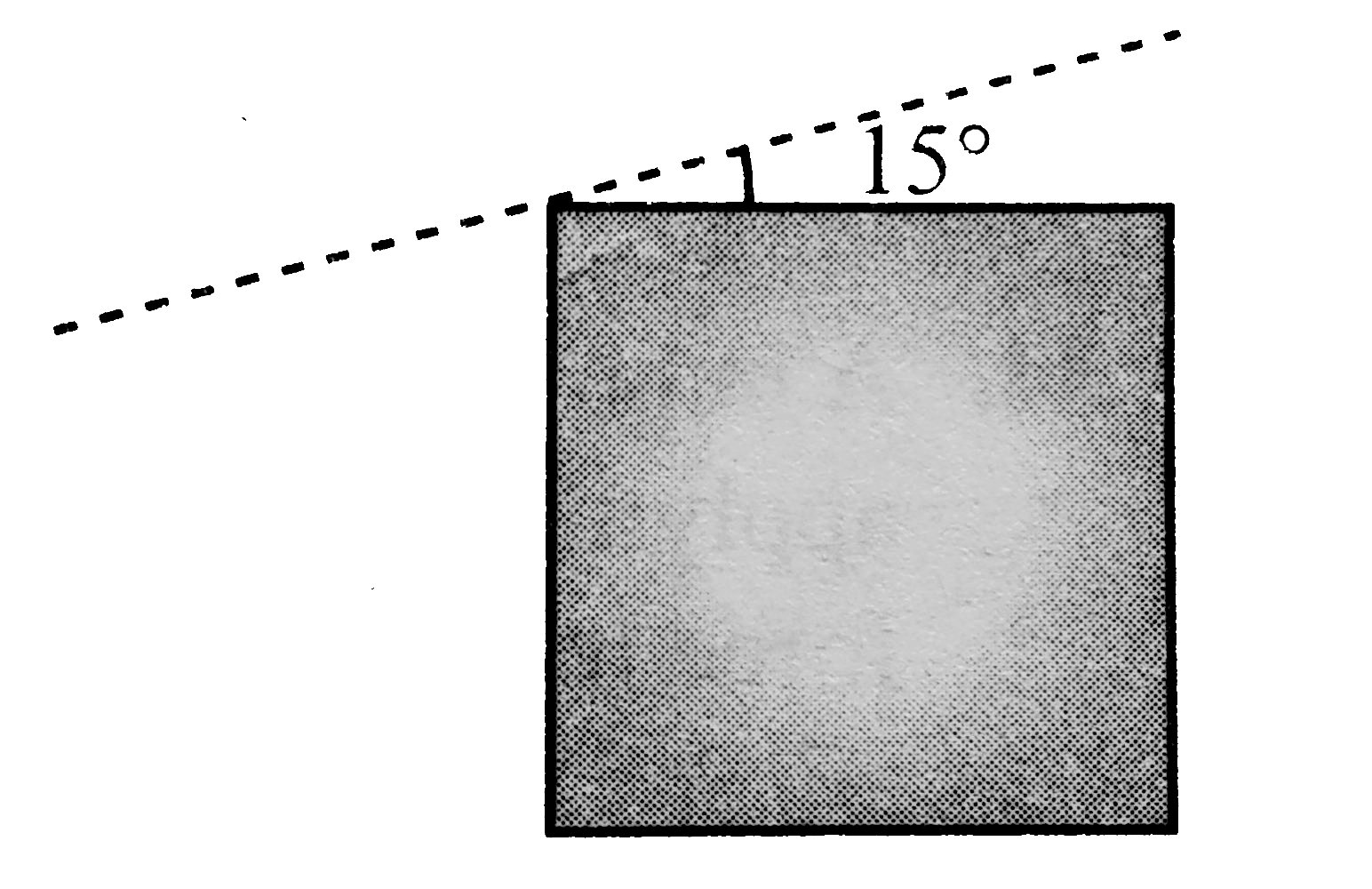 View Solution
View Solution

