Demonstare that a closed system of charged non-relativistic particles

For a non relativistic $(v < < c)$ charged particle, specific charge $\frac{q}{m}$ :
A $\frac{q}{m}$ = con stant
B $\frac{q}{m} \neq$ constant
C $\frac{q}{m} = C^{2}$
D $\frac{q}{m} = μ_{0} \in_{0}$
Value of e/m (specific charge) of the cathode ray particles
Adepends on potential difference of cathode and anode
Bdepends on nature of gas in the discharge tube
Cis independet of material of cathod eor gas in tube
Ddepends on nature of metal used for cathode
A charged particle of specific charge s passes undeviated through region 1 as shown in Fig.

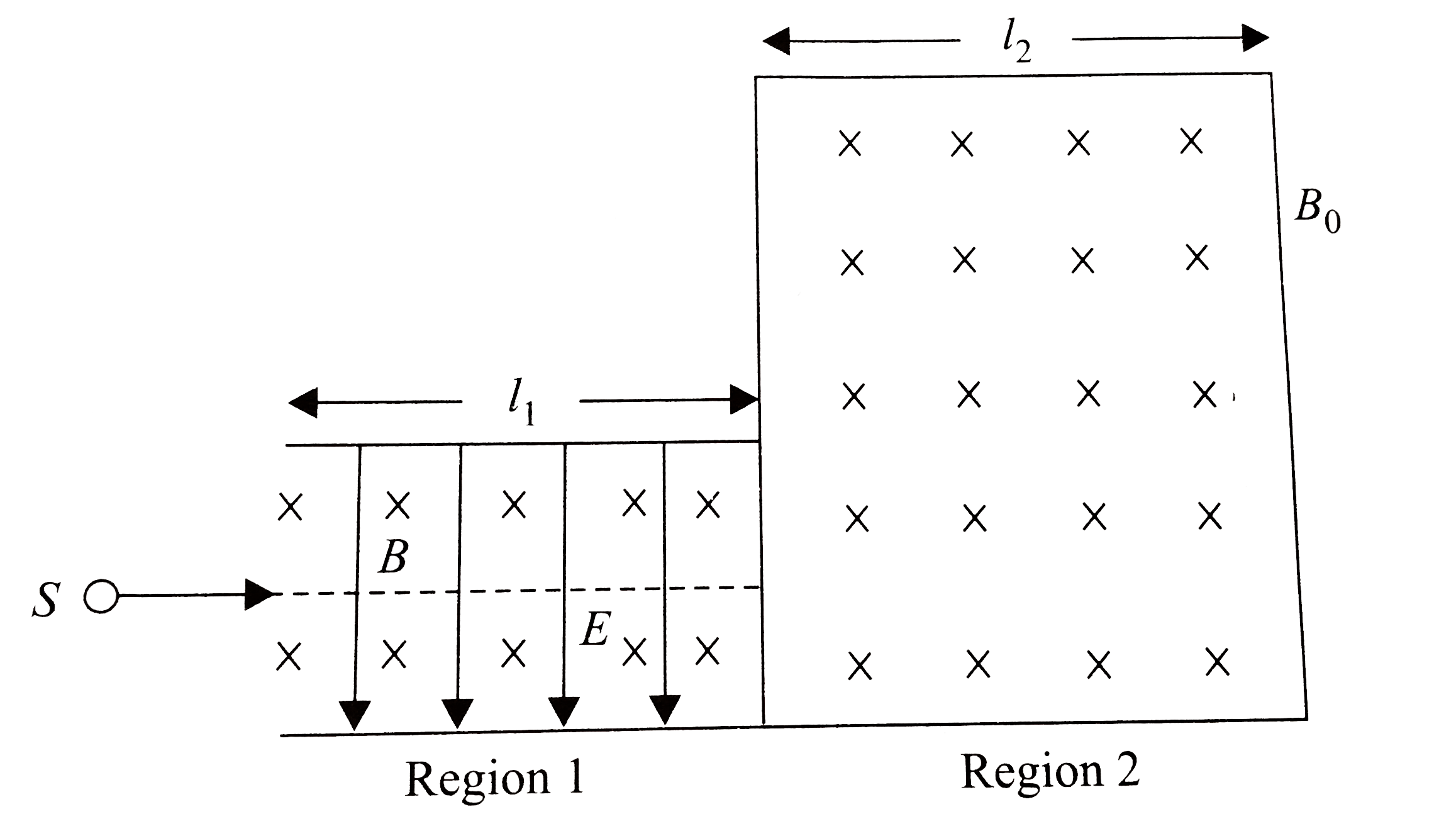AVelocity of paticle in rigion 1 is $v = \frac{E}{B}$
BWork done to move the charged particle in region 1 and region 2 is zero.
CThe radius of the trajectory of the charged particle in Region 2 is $(E / s B B_{0})$
DThe particle emerges from region 2 with a velocity $\to v' w h e r e$ vec(v') $= - \to v$ for $l_{2} > \frac{E}{B B_{0}}$

Demonstare that a closed system of charged non-relativistic particles with identical specific charges emits no dipole radiation.