Derive an expression for phase angle between the applied voltage and current in a series RLC circuit.
Let us consider a circuit containing a resistor of resistance R, a inductor of inductance L and a capacitor capacitance C connected across an alternating voltage source.
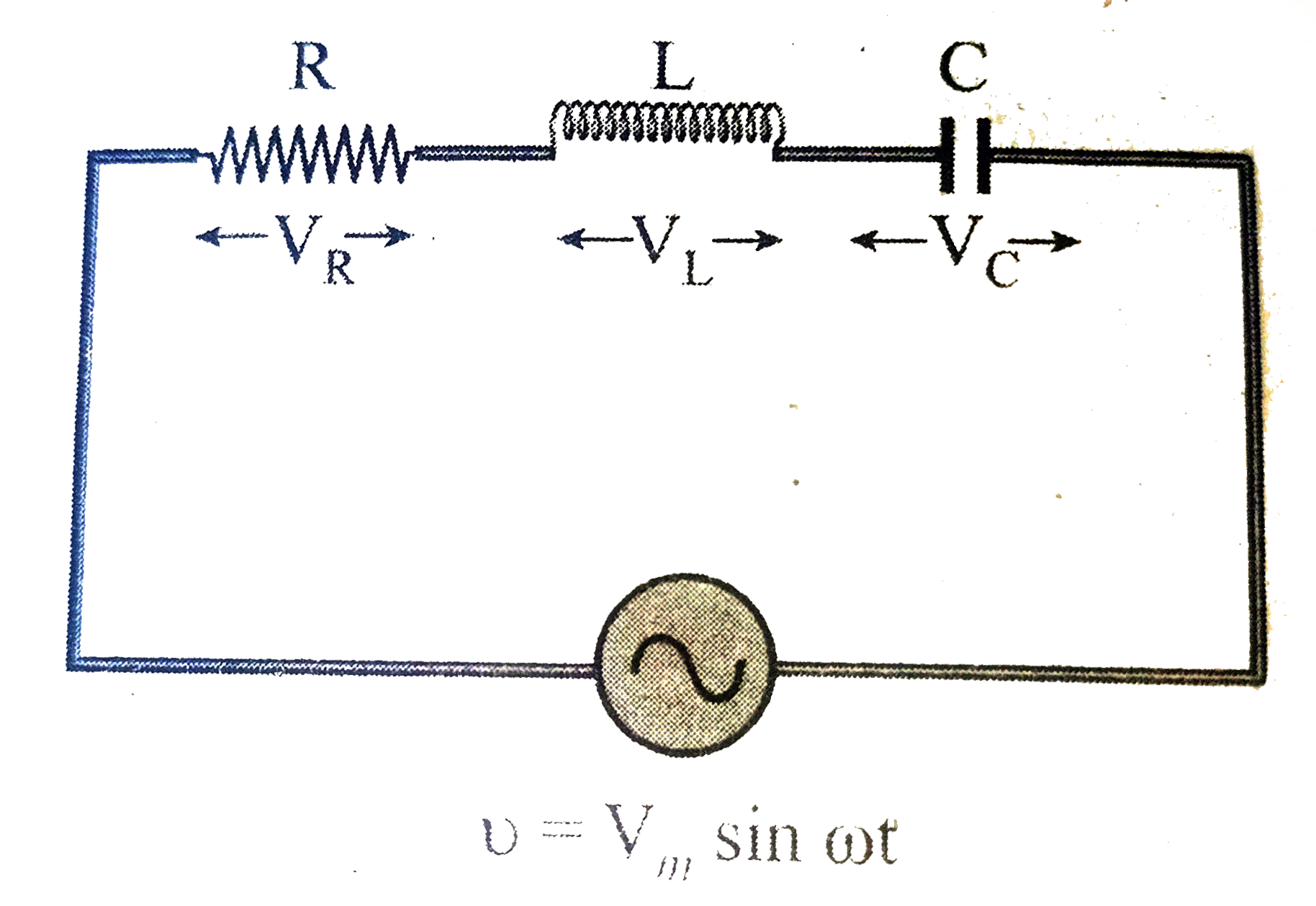
The applied alternating voltage is given by the equation.
v=Vmsinωt ...(1)
Let i be the resulting circuit current in the circuit at that instant. As a result, the voltage is devloped across R,L and C.
We know that voltage across R (VR) is in phase with i, voltage across L(VL) leads i by π/2 and voltage across C(VC) lags i by π/2.
The phasor diagram is drawn with current as the reference phasor. The current is represented by the phasor.−→OI,VRby−−→OA, VLby−−→OBandVCby−−→OC.
The lenght of these phasors are OI=Im,
OA=ImR,OB=ImXL,OC=ImXC
The circuit is eighter effectively inductive or capacitive or resistive that depends on the value of VL or VC. Let us assume that VL>VC so that net voltage drop across L-C combination is VL−VC which is represented by a phasor −−→AD.
By parallelogram law, the diagonal −−→OE gives the resultant voltage v of VR and (VL−VC) and its lenght OE is equal to Vm. Therefor,
Vm2=VR2+(VL−VC)
=√(ImR)2+(ImXL−ImXC)2
=Im√R2+(XL−XC)2
or Im=Vm√R2+(XL−XC)2 ...(2)
or Im=VmZ
where Z=√R2+(XL−XC)2 (3)
Z is called impedance of the circuit which refers to the effective opposition to the circuit currenty by the series RLC circuit. The voltage triangle and impedance triangle.
From phasor diagram, the phase angle between v and i is found out from the following relation
tanθ=VL−VCVR=XL−XCR
Special Cases:
(i) If XL>XC,(XL−XC)
is positive and phase angle ϕ is also positive. It means that the applied voltage leads the current by ϕ (or current lags behind voltage by ϕ ). The circuit is inductive.
∴v=Vmsinωt,i=Imsin(ωt−ϕ)
(ii) If XL<XC,(XL−XC) is negative and ϕ is also negative. Therefore current leads voltage by ϕ and the circuit is capacitive .
∴v=Vmsinωt,i=Imsin(ωt+ϕ)
(iii) If XL=XC,ϕ is zero. Therefore current and voltage are in the same phase and the circuit is resistive.
∴v=Vmsinωt,i=Imsinωt
Similar Questions
The phase difference between voltage and current in series L-C circuit is
View SolutionIn a series LCR circuit, the voltages across an inductor, a capacitor and a resistor are 30 V, 30 V, 60 V respectively. What is the phase difference between the applied voltage and the current in the circuit ?
View Solution
Knowledge Check
The phase difference between voltage and current in series L-C circuit is
A0∘BπCπ2Dπ3The phase difference between voltage and current in an LCR series circuit is
Azero alwaysBπ/4 alwaysCπDbetween 0 and π/2In series LCR circuit, the phase angle between supply voltage and current is
Atanϕ=XL−XCRBtanϕ=RXL−XCCtanϕ=RXL+XCDtanϕ=XL+XCR
In a series LCR circuit, the voltage across an inductor, a capacitor and a resistor are 20 V, 20 V and 40 V, respectively. What is the phase difference between the applied voltage and the current in the circuit ?
View SolutionIn a series LCR circuit, the voltages across an inductor, a capacitor and a resistor are 30 V, 30 V, 60 V respectively. What is the phase difference between the applied voltage and the current in the circuit ?
View SolutionIn a series LCR circuit, the voltages across an inductor, a capacitor and a resistor are 30 V, 30 V, 60 V respectively. What is the phase difference between the applied voltage and the current in the circuit ?
View SolutionIn series LCR circuit, the phase angle between supply voltage and current is
View SolutionAn L-C-R circuit is connected to a source of A.C. current. At resonance, the phase difference between the applied voltage and the current in the circuit, is
View Solution
