Eleven forces each equal to 5N act on a particle simultaneously. If each force makes an angle 30° with the next one, the resultant of all forces is
15N
55 N
5 N
zero
The correct Answer is:C
To find the resultant of the eleven forces each equal to 5 N acting at an angle of 30° with respect to each other, we can follow these steps:
Step 1: Understand the Configuration of Forces
We have 11 forces, each with a magnitude of 5 N. Each force is separated by an angle of 30° from the next force.
Step 2: Calculate the Total Angle
Since there are 11 forces, the total angle they would cover is:
Total angle=(11−1)×30°=300°
This means that the forces are not completing a full circle (360°), which is important for understanding how they combine.
Step 3: Determine the Minimum Forces for Zero Resultant
To achieve a zero resultant, we need to consider how many forces would be required to cancel each other out. The minimum number of forces required to achieve a zero resultant is determined by:
Minimum forces for zero resultant=360°30°=12
Since we only have 11 forces, we cannot achieve a zero resultant.
Step 4: Analyze the Remaining Force
Since we have 11 forces and need 12 for a zero resultant, we conclude that 10 of these forces will effectively cancel each other out, leaving us with one unopposed force.
Step 5: Calculate the Resultant Force
The only remaining force that does not get canceled out is one of the 5 N forces. Therefore, the resultant of all the forces acting on the particle is:
Resultant=5N
Conclusion
Thus, the resultant of all 11 forces acting on the particle is 5 N.
We have 11 forces, each with a magnitude of 5 N. Each force is separated by an angle of 30° from the next force.
Since there are 11 forces, the total angle they would cover is:
Total angle=(11−1)×30°=300°
This means that the forces are not completing a full circle (360°), which is important for understanding how they combine.
To achieve a zero resultant, we need to consider how many forces would be required to cancel each other out. The minimum number of forces required to achieve a zero resultant is determined by:
Minimum forces for zero resultant=360°30°=12
Since we only have 11 forces, we cannot achieve a zero resultant.
Since we have 11 forces and need 12 for a zero resultant, we conclude that 10 of these forces will effectively cancel each other out, leaving us with one unopposed force.
The only remaining force that does not get canceled out is one of the 5 N forces. Therefore, the resultant of all the forces acting on the particle is:
Resultant=5N
Thus, the resultant of all 11 forces acting on the particle is 5 N.
Similar Questions
Twelve forces each of 5 N act on a body simultaneously. If each force makes an angle of 30° with other their resultant is
View Solution100 coplanar forces each equal to 10 N act on a body. Each force makes angle π50 with the preceding force. What is the resultant of the forces
View SolutionFive equal forces each of 20N are acting at a point in the same plane. If the angles between them are same, the resultant of these forces is
View SolutionTwo equal forces (P each) act at a point inclined to each other at an angle of 120∘. The magnitude of their resultant is
View SolutionA force of 10.5 N acts on a particle along a direction making an angle of 370 with the vertical. Find the component of the force in the vertical direction.
View SolutionA force of 5N acts on a particle along a direction making an angle of 60∘ with verticle. Its verticel components is
View SolutionTwo forces of magentude 7 newton and 5 newton act on a particle at an angle θ can have any value . The minimum magnitude of the resultant forces is :
View SolutionOne of the two rectangular components of a force is 10 N and it makes an angle 60° with the force. The magnitude of the force is :
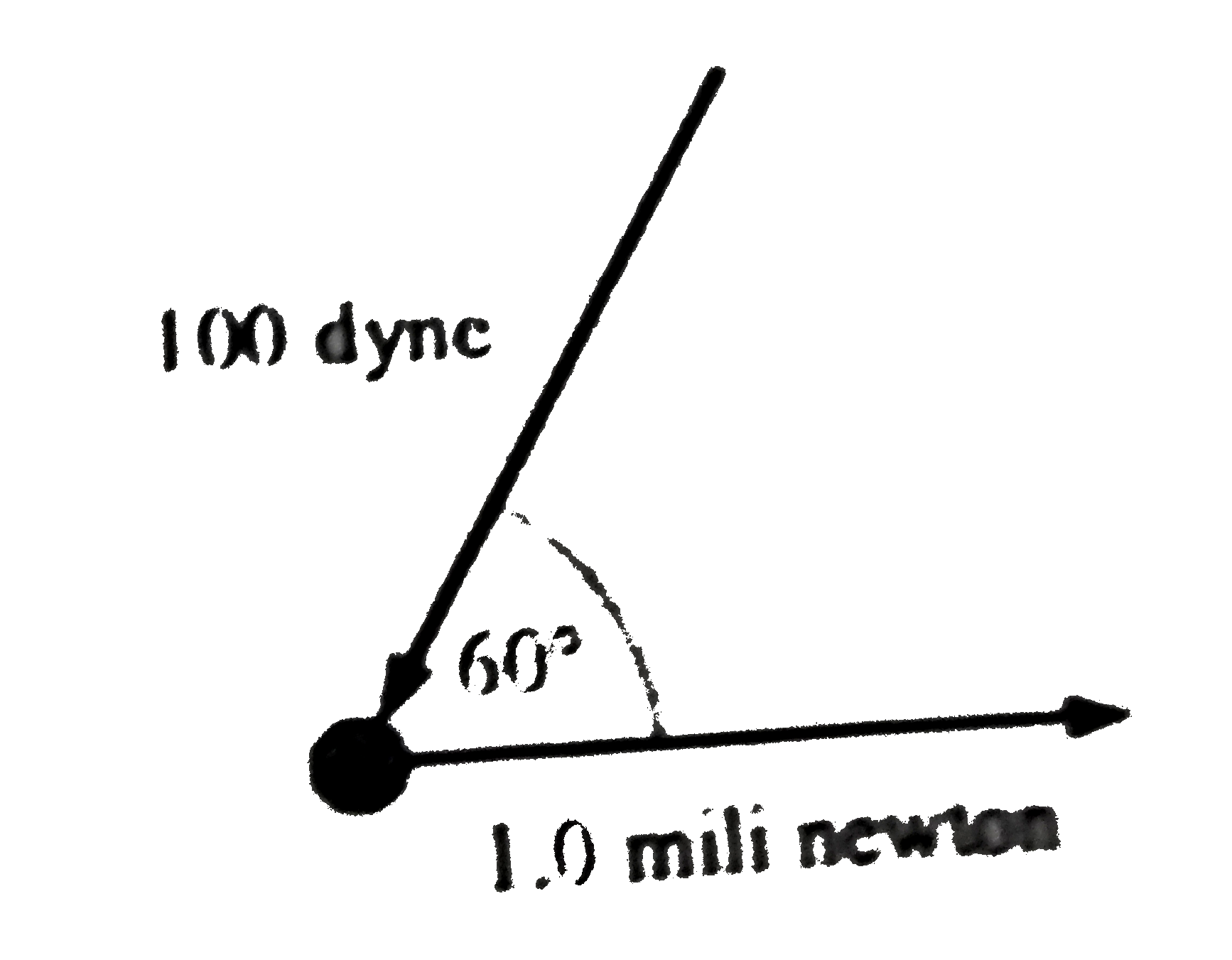
View SolutionTwo forces act on a particle simultaneously as shown in the figure. Find net force in milli newton on the particle. [Dyne is the CGS unit of forces ]
View SolutionFive equal forces of 10N each are applied at one point and all are lying one plane. If the angles between them are equal, the resultant force will be
View Solution
