Estimate the number of mean lives elapsed, when the number of atoms in a radioactive sample decrease to 5% of the original value.
Let the required number of mean lives elapsed be .m., then
t=m<τ≥mλ
N=5% of N0=120N0
But, N=N0e−λt
∴N020=N0e−m or , em=20
or , m=ln 20 = ln 2+ ln 10 = 0.693 + 2.303 ≈ 3
∴ At the end of about three average lives, the number of atoms (undecayed) would fall to 5%.
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - NUCLEIEXERCISE - Exercise-I79videos
NUCLEI
- BOOK - AAKASH SERIESCHAPTER - NUCLEIEXERCISE - Exercise-II40videos
NUCLEI
- BOOK - AAKASH SERIESCHAPTER - NUCLEAR PHYSICSEXERCISE - ADDITIONAL PRACTICE PRACTICE SHEET (ADVANCED) Integer Type Questions3videos
NUCLEAR PHYSICS
- BOOK - AAKASH SERIESCHAPTER - OPTICAL INSTRUMENTS EXERCISE - PRACTICE SHEET (EXERCISE -I) (OPTICAL INSTRUMENTS) (LEVEL-I (MAIN)) (SUBJECTIVE OBJECTIVE TYPE QUESTIONS)21videos
OPTICAL INSTRUMENTS
Similar Questions
Calculate the number of half lives elapsed, the end of which, the activity of a radioactive sample decrease by 90%.
View SolutionFind the number of half lives elapsed, before which, 93.75% of a radioactive sample has decayed.
View SolutionThe sample with largest number of atoms is
View SolutionHow many atoms decay in one mean life time of a radioactive sample-
View SolutionThe rate at which radioactive substances decay is known to be proportional to the number of such nuclei that are present in a given sample. In a certain sample 10% of the original number of radioactive nuclei have undergone disintegration in a period of 100 years. Find what percentage of the original radioactive nuclei will remain after 1000 years.
View SolutionThe decay constant of a radioactive substance is0.173year−1. Therefore,
View SolutionAfter three half lives, the percentage of fraction of amount a).35 b)12.5 c)50 d)75
View SolutionA sample of radioactive material A, that has an activity of 10mCi(1Ci=3.7×1010decays/s), has twice the number of nuclei as another sample of a different radioactive material B which has an activity of 20 mCi. The correct choices for half-lives of A and B would then be respectively :
View Solutionuse shortcut method to find the mean of monthly wages of a certain number of workers:
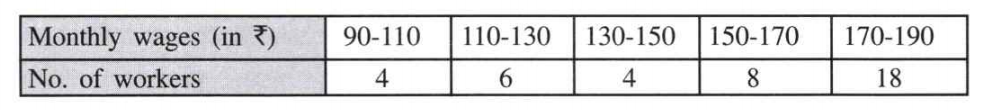
Find the new mean, when:
the number of workers in each category is doubled and the monthly wage in each category is decreased by 20%.View SolutionWhich of the following samples contains the largest number of atoms ? .
View Solution

