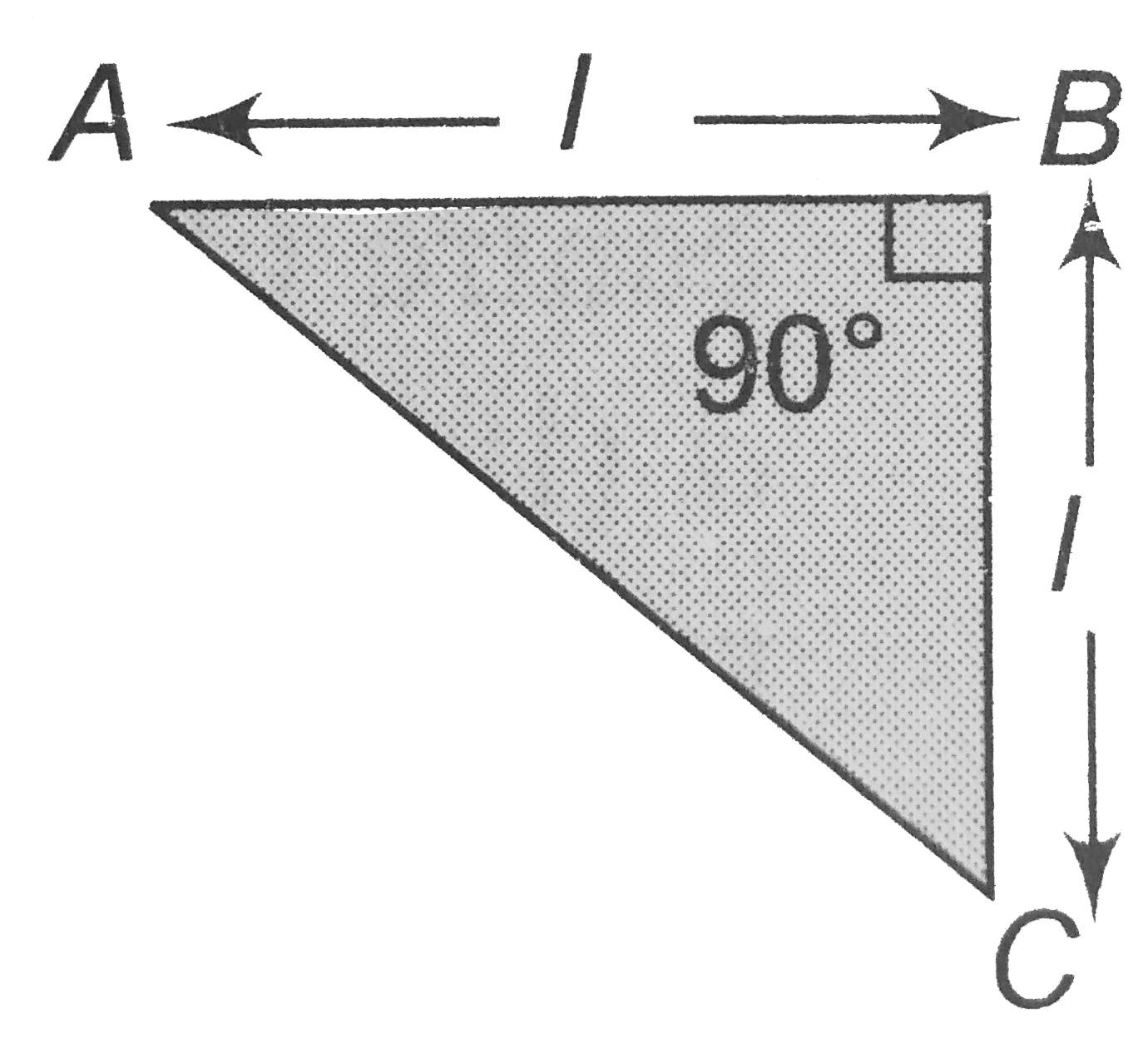
Figure shows a thin metallic triangular sheet ABC. The mass of the sheet is M. The moment of inertia of the sheet about side AC is :
 .
.
Ml218
Ml212
Ml26
Ml24
The correct Answer is:B
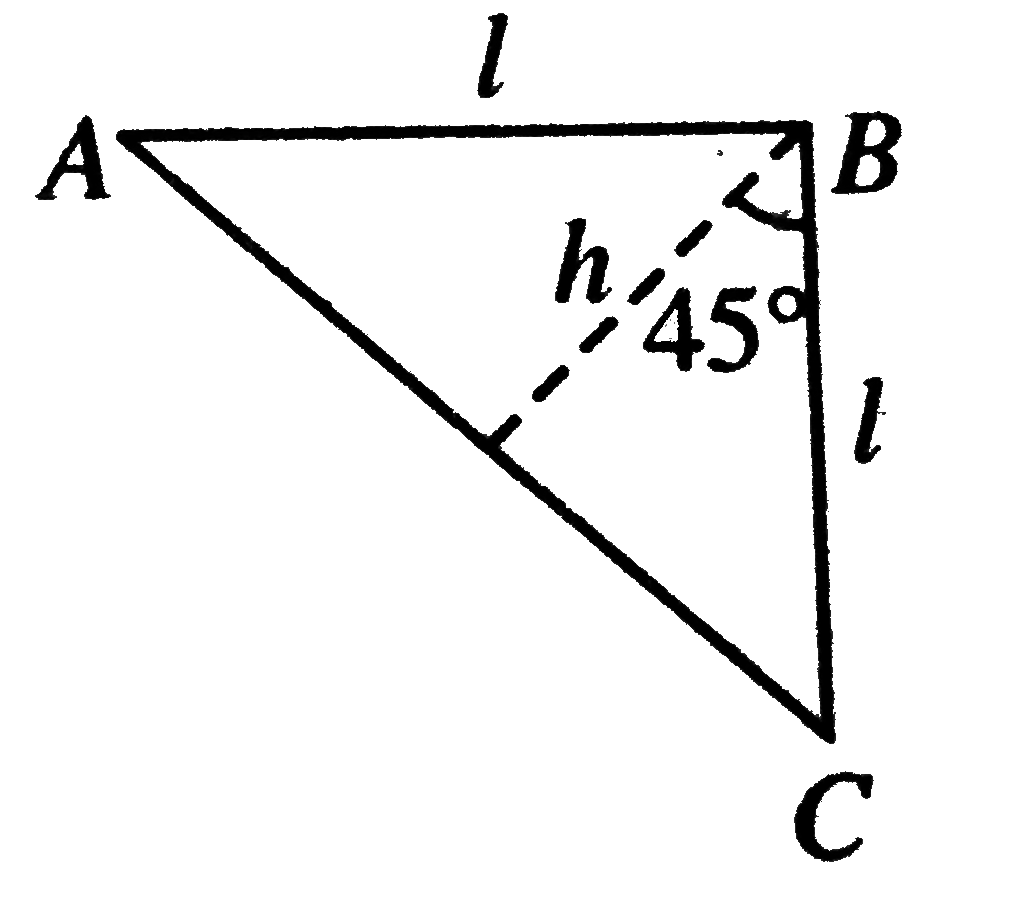
h=cos45∘=l√2
IAC=16Mh2=16M(l√2)2=Ml22
Topper's Solved these Questions
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Multiple Correct
26 Videos
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Linked Comprehension
71 Videos
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Subjective
19 Videos
- RIGID BODY DYNAMICS 1BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer
11 Videos
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICSCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer
16 Videos
Similar Questions
A thin uniform metallic triangular sheet of mass M has sides AB=BC=L. What is its moment of inertia about axis AC lying in plane of sheet ?

View SolutionThree particles, each of mass m are situated at the vertices of an equilateral triangle ABC of side l (as shown in the figure).
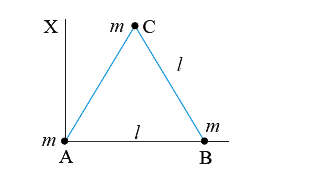
The moment of inertia of the system about a line AX perpendicular to AB and in the plane of ABC, will be:
View Solution
Knowledge Check
In a metallic triangular sheet ABC, AB=BC=l. If M is mass of sheet, what is the moment of inertia about AC?
 AMl24BMl212CMl26DMl218
AMl24BMl212CMl26DMl218In a metallic triangular sheet ABC, AB=BC=l. If M is mass of sheet, what is the moment of inertia about AC?
 AMl24BMl212CMl26DMl218
AMl24BMl212CMl26DMl218The circumference of a circular sheet is 154 m. The area of the sheet is equal to
A1886.5m2B1806.5m2C1886.5m2D1688.5m2
Three particles, each of mass m are situated at the vertices of an equilateral triangle ABC of side L figure. Find thee moment of inertia of the system about the line AX perpendicular to AB in the plane of ABC

View SolutionThe moment of inertia of a triangular lamina of mass M about edge AB is equal to?
View SolutionAn equilateral triangle ABC is cut from a thin solid sheet of wood .(see figure ) D,E and F are the mid-points of its sides as shown and G is the centre of the triangle.The moment of inertia of the triangle about an axis passing through G and perpendicular to the plane to the triangle is I0 If the smaller triangle DEF is removed from ABC , the moment of inertia of the remaining figure about the same axis is I. Then :

View SolutionThe figure shows an isosceles triangular plate of mass M and base L. The angle at the apex is 90∘ . The apex lies at the origin and the base is parallel to X - axis.
The moment of inertia of the plate about its base parallel to the x-axis is

View SolutionA uniform plane sheet of metal in the form of a triangle ABC has BC>AB>AC. Its moment of inertia will be smallest
View Solution
