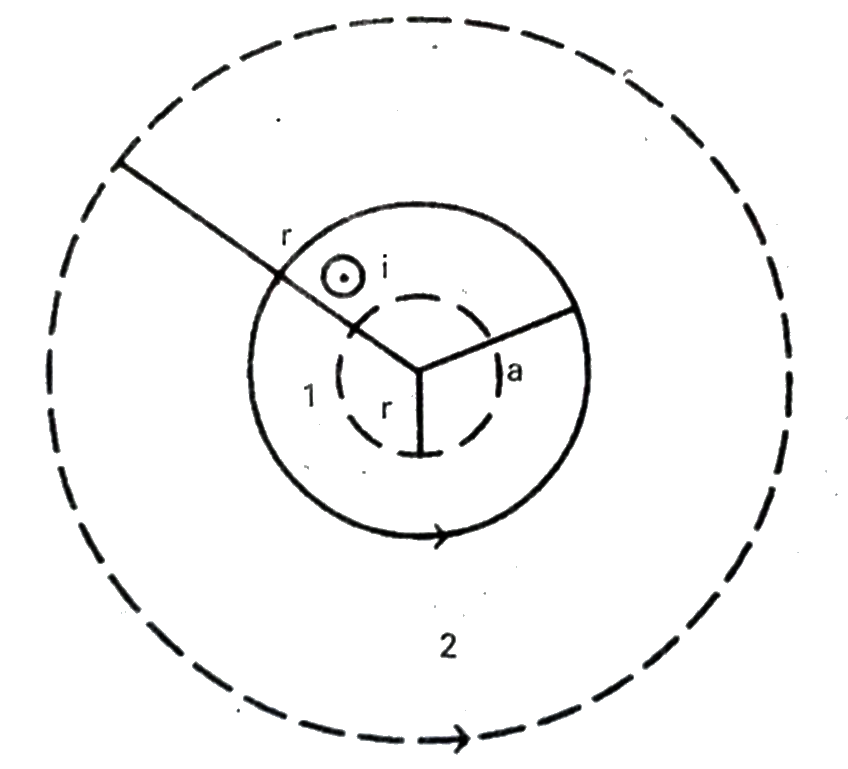
Figure shows the circular cross-section of a long straight wire of radius a carrying steady current I. The current I is uniformly distributed across this cross-section. Calculate the magnetic field in the region r<a (dashed inner circle) and r>a (dashed outer circle).
 Solution in Telugu
Solution in Telugu
a) Consider the case r>a. The Amperian loop, labelled 2, is a circle concentric with the cross-section. For this loop,
L=2πr
Ie= Current enclosed by the loop = I
The result is the familiar expression for a long straight wire B(2πr)=μ0I
B=μ0I2πr
b) Consider the case r<a. The Amperian loop is a circle labelled 1. For this loop, taking the radius of the circle to be r,
L=2πr
Now the current enclosed Ie is not I (because r<a), but is less than this value. Since the current distribution is uniform, the current enclosed is,
Ie=I(πr2πa2)=Ir2a2
Using Ampere's law, B(2πr)=μ0Ir2a2
B=(μ0I2πa2)r −(2)
B∝r(r<a)
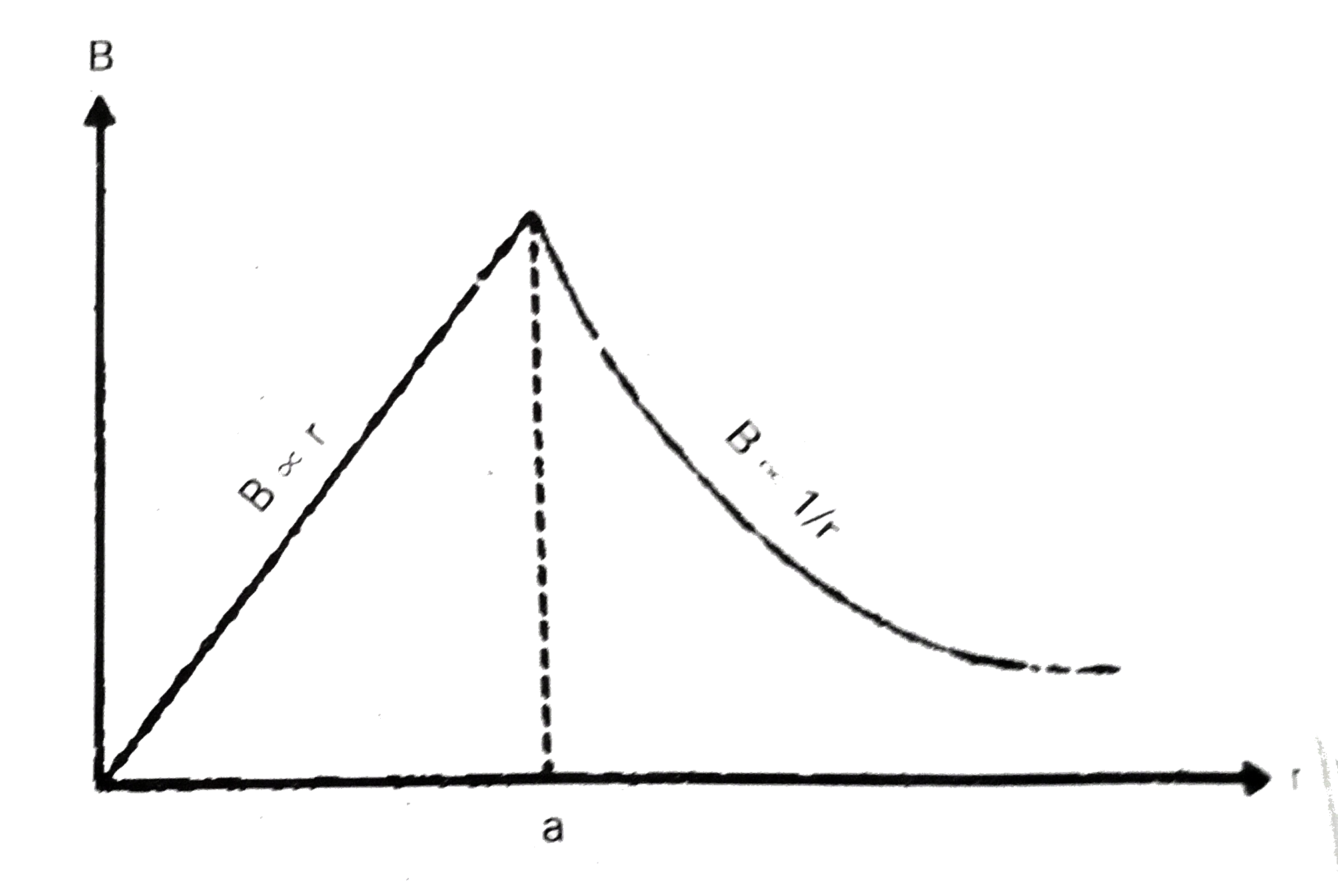
Figure shows a plot of the magnitude of B with distance r from the centre (axis) of the wire. The direction of the field is tangential to the respective circular loop (1 or 2) and given by the right-hand rule dsecribed earlier in this section.
This example possesses the required symmetry so that Ampere's law can be applied readily.
Topper's Solved these Questions
- MOVING CHARGES AND MAGNETISHMBOOK - VIKRAM PUBLICATION ( ANDHRA PUBLICATION)CHAPTER - MOVING CHARGES AND MAGNETISHMEXERCISE - VERY SHORT ANSWER QUESTIONS
15 Videos
- MOVING CHARGES AND MAGNETISHMBOOK - VIKRAM PUBLICATION ( ANDHRA PUBLICATION)CHAPTER - MOVING CHARGES AND MAGNETISHMEXERCISE - SHORT ANSWER QUESTIONS
9 Videos
- MOVING CHARGES AND MAGNETISHMBOOK - VIKRAM PUBLICATION ( ANDHRA PUBLICATION)CHAPTER - MOVING CHARGES AND MAGNETISHMEXERCISE - ADDITIONAL EXERCISES
26 Videos
- MAGNETISM AND MATTERBOOK - VIKRAM PUBLICATION ( ANDHRA PUBLICATION)CHAPTER - MAGNETISM AND MATTEREXERCISE - Additional Exercises
19 Videos
- NUCLEIBOOK - VIKRAM PUBLICATION ( ANDHRA PUBLICATION)CHAPTER - NUCLEIEXERCISE - DAM SURE (VSAQ)
1 Video
Similar Questions
What is the importance of Oersted's experiment ?
View SolutionState and explain Biot-Savart law.
View SolutionTwo concentric circular coils X and Y of radii 16 cm and 10 cm, respectively, lie in the same vertical plane containing the north to south direction. Coil X has 20 turns and carries a current of 16 A, coil Y has 25 turns and carries a current of 18 A. The sense of the current in X is anticlockwise, and clockwise in Y, for an observer looking at the coils facing west. Give the magnitude and direction of the net magnetic field due to the coils at their centre.
View SolutionWhy does a paramagnetic sample display greater magnetisation (for the same magnetising field) when cooled?
View SolutionThe half life of .58Co is 72 days. Calculate its average life.
View SolutionFigure shows a long straight wire of circular crosssection (radius a) carrying steady current I. The current I is uniformly distributed across this crosssection. Calculate the magnetic field in the region r<a and r>a.

View SolutionFigure shows a long straight wire of a circular cross-section (radius a) carrying steady current l. The current l is uniformly distributed across this cross-section. Calculate the magnetic field in the region r<aandr>a

View SolutionFigure shows a long straight wire of circular crosssection (radius a) carrying steady current I. The current I is uniformly distributed across this crosssection. Calculate the magnetic field in the region r<a and r>a.

View SolutionFigure shows a long straight wire of a circular cross - section (radius a) carrying steady current I. The current I is uniformly distributed across this cross - section . Calculate the magnetic field in the region r lt a and r gt a
View SolutionThe adjoining figure shows a long straight wire of a circular cross section (radius a) carrying steady current I. The current I is uniformly distributed across its cross section. Calculate the magnetic field at a distance from its axis for (i) r lt a , (ii) r gt a .
View SolutionFigure shows a long straight wire of a circular cross-section (radius a) carrying steady current I. The current I is uniformly distributed across this cross-section. Calculate the magnetic field in the region r a.
View SolutionA long straight wire of a circular cross-section (radius r) carries a steady current I. The current is distributed uniformly across the cross-section. Calculare the magnetic field at a point (a) outside the wire , (b) inside the wire. Draw a graph showing variation of magnetic field.
View SolutionFigure shows a long straight wire of circular crosssection (radius a) carrying steady current I. The current I is uniformly distributed across this crosssection. Calculate the magnetic field in the region r<a and r>a.

View SolutionA long straight wire of a curcular cross-section of radius 'a' carries a steady current 'l'. The current is uniformly distributed the across- section . Apply Amphere's circuital law to calculate the magnetic field at a point 'r' in the region for (i) r lt a and (ii) r gt a .
View SolutionA long straight wire of radius 'a' carries a steady current i. The current is uniformly distributed across its cross section. The ratio of the magnetic fields at a/2 and 2a is
View Solution