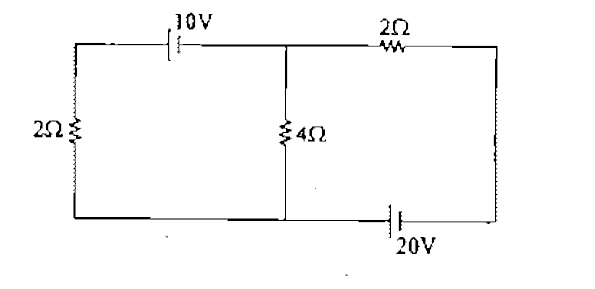
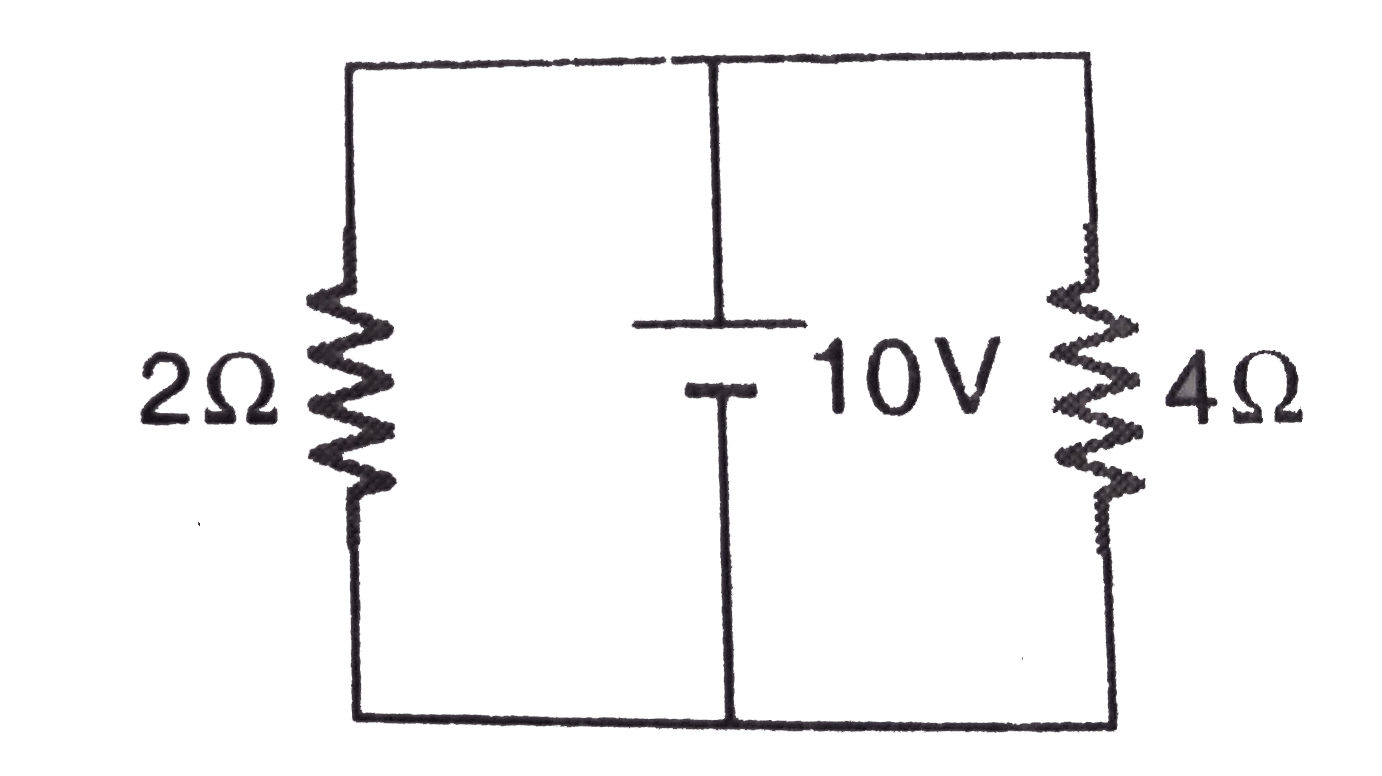
Find the current passing through 2Ω
and 4Ω resistance in the circuit shown in figure.
The correct Answer is:A
To find the current passing through the 2 Ω and 4 Ω resistors in the given circuit, we will analyze the circuit step by step.
Step 1: Analyze the Circuit
In the circuit, we have a 10V battery connected to two diodes (D1 and D2) and two resistors (2 Ω and 4 Ω).
Step 2: Determine the State of Diodes
- Diode D1 is connected in the forward direction (positive terminal of the battery to the anode of D1), which means it will conduct current.
- Diode D2 is connected in the reverse direction (negative terminal of the battery to the anode of D2), which means it will not conduct current.
Step 3: Current Flow Analysis
Since D2 is reverse-biased, it will not allow any current to flow through it. Therefore, the only path for the current is through D1 and the 2 Ω resistor.
Step 4: Calculate the Current Through the 2 Ω Resistor
Using Ohm's Law (V = IR), we can find the current flowing through the 2 Ω resistor. The voltage across the 2 Ω resistor is equal to the voltage of the battery since D2 does not conduct.
- Voltage (V) = 10V
- Resistance (R) = 2 Ω
Using Ohm's Law:
I=VR=10V2Ω=5A
Step 5: Current Through the 4 Ω Resistor
Since the current can only flow through the 2 Ω resistor (due to D2 being reverse-biased), the current through the 4 Ω resistor is 0 A.
Summary of Results
- Current through the 2 Ω resistor: 5 A
- Current through the 4 Ω resistor: 0 A
In the circuit, we have a 10V battery connected to two diodes (D1 and D2) and two resistors (2 Ω and 4 Ω).
- Diode D1 is connected in the forward direction (positive terminal of the battery to the anode of D1), which means it will conduct current.
- Diode D2 is connected in the reverse direction (negative terminal of the battery to the anode of D2), which means it will not conduct current.
Since D2 is reverse-biased, it will not allow any current to flow through it. Therefore, the only path for the current is through D1 and the 2 Ω resistor.
Using Ohm's Law (V = IR), we can find the current flowing through the 2 Ω resistor. The voltage across the 2 Ω resistor is equal to the voltage of the battery since D2 does not conduct.
- Resistance (R) = 2 Ω
I=VR=10V2Ω=5A
Since the current can only flow through the 2 Ω resistor (due to D2 being reverse-biased), the current through the 4 Ω resistor is 0 A.
- Current through the 2 Ω resistor: 5 A
- Current through the 4 Ω resistor: 0 A
Topper's Solved these Questions
- SEMICONDUCTORSBOOK - DC PANDEYCHAPTER - SEMICONDUCTORSEXERCISE - Solved Example
14 Videos
- SEMICONDUCTORSBOOK - DC PANDEYCHAPTER - SEMICONDUCTORSEXERCISE - Exercise35.1
1 Videos
- REFRACTION OF LIGHTBOOK - DC PANDEYCHAPTER - REFRACTION OF LIGHTEXERCISE - Level 2 Subjective
21 Videos
- SOLVED PAPER 2017BOOK - DC PANDEYCHAPTER - SOLVED PAPER 2017EXERCISE - Solved papers 2017(JIPMER)
32 Videos
Similar Questions
Knowledge Check
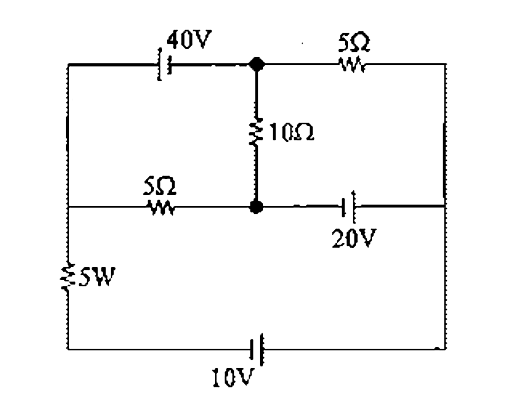
The current passing through 20Ω resistance in the circuit arrangement shown here will be
 A0.1AB0.2AC0.5AD0
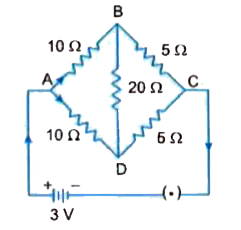
A0.1AB0.2AC0.5AD0Current passing through 3Ω resistance is
 A143AB3AC2AD125A
A143AB3AC2AD125AFind out the current flowing through 2Ω resistance in the given circuit.
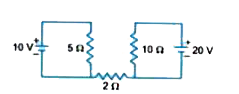 A5AB2AC0D4A
A5AB2AC0D4A
Find the current through 2Ω and 4Ω resistance.
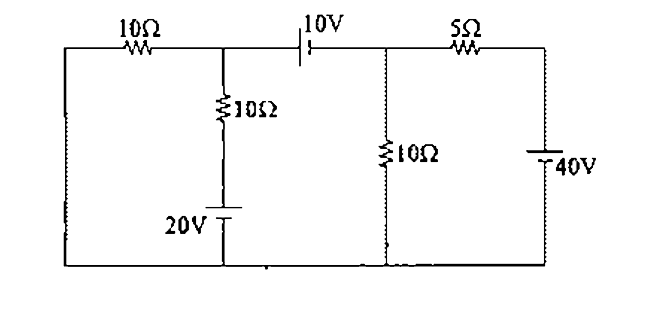
View SolutionFind the current in 5Ω resistance incircuit shown in figure-3.110
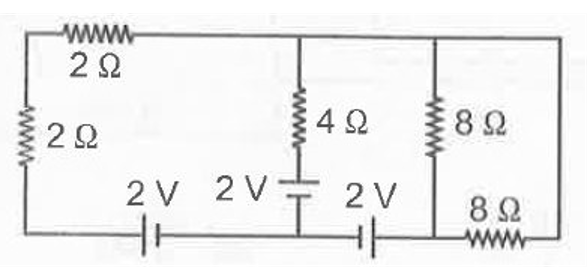
View SolutionFind the current through 4Ω resistor in the circuit shown in figure.
View SolutionFind the equivalent resistance and current in 6Ω resistance in the circuit shown in figure 3.55.
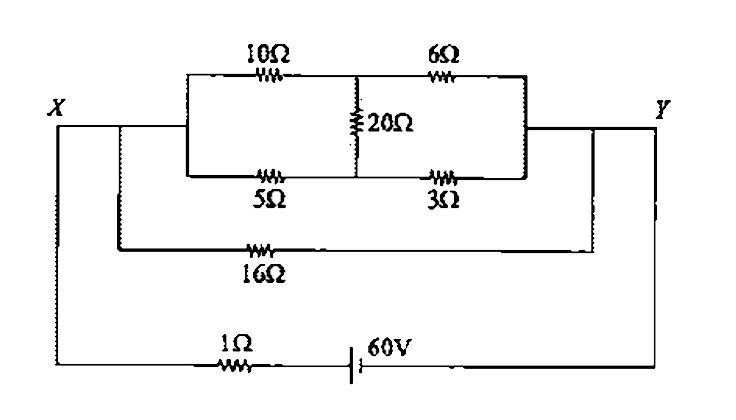
View SolutionFind the current l through then 10Ω resistance in the network shown in figure.
View Solution