Find the equivalent cappacitance between A and B.
(a) Three conducting concentic shells of radii r,2r and
(b) An isoltated ball-shaped conductor of radius r surrounded by an adjuacent layer of dielectric (K) and outer radius 2r.
Two conducting concentic spherical shells having radii r1 and r2(r2>r1), calculate capacity of the system if
(i) The inner shell is given a charge and outer is connected to the earth.
(ii) The outer shell is given a charge and inner is connected to the earth.
(d) Find capacitance of the system
(i) Two shells of radii r1 and r2 having charges are connected by a metallic wire, the separation between shells is large.
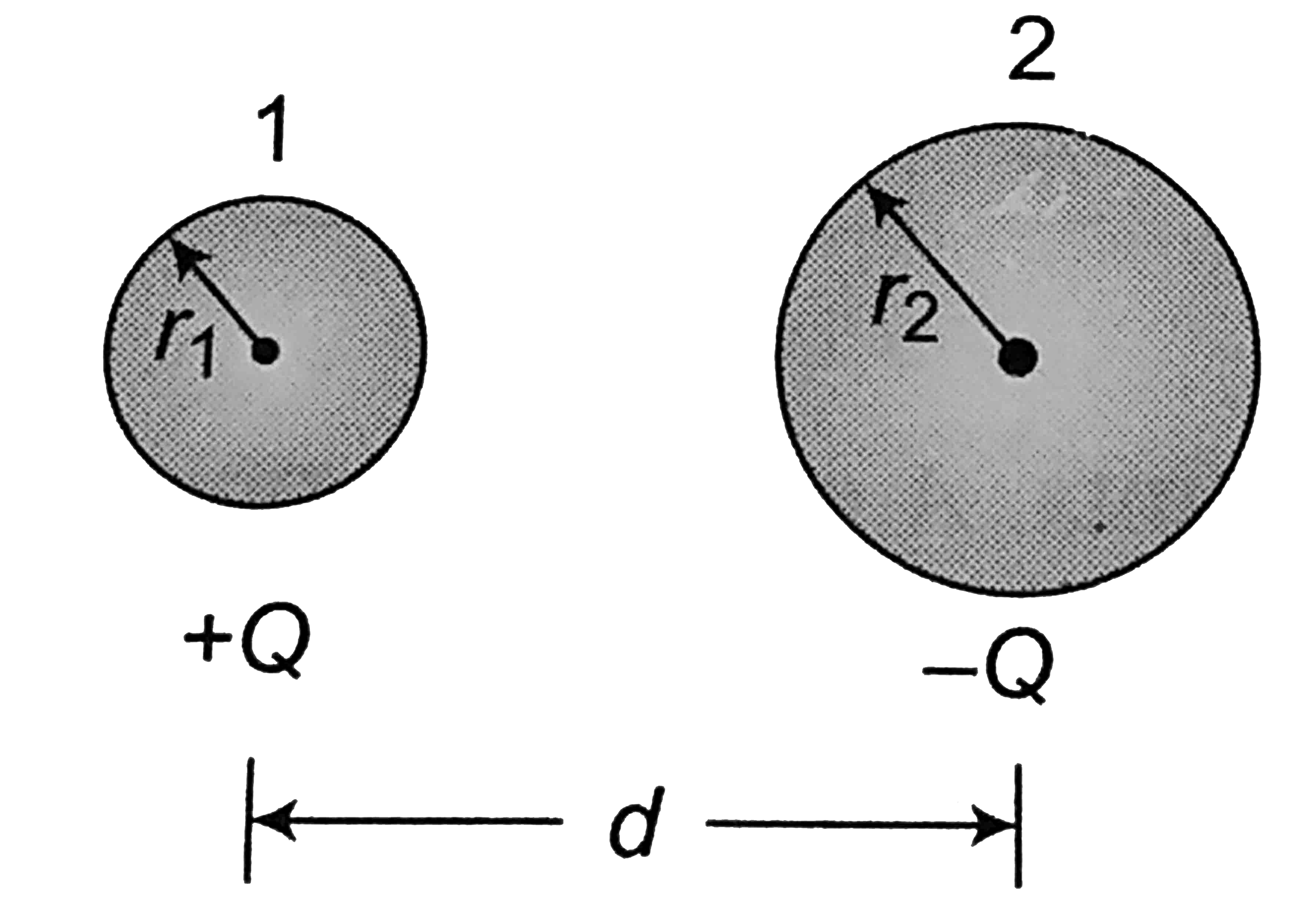
(ii) Two shells of radii r1 and r2 carry equal and opposite charges and are separated by a distance d.
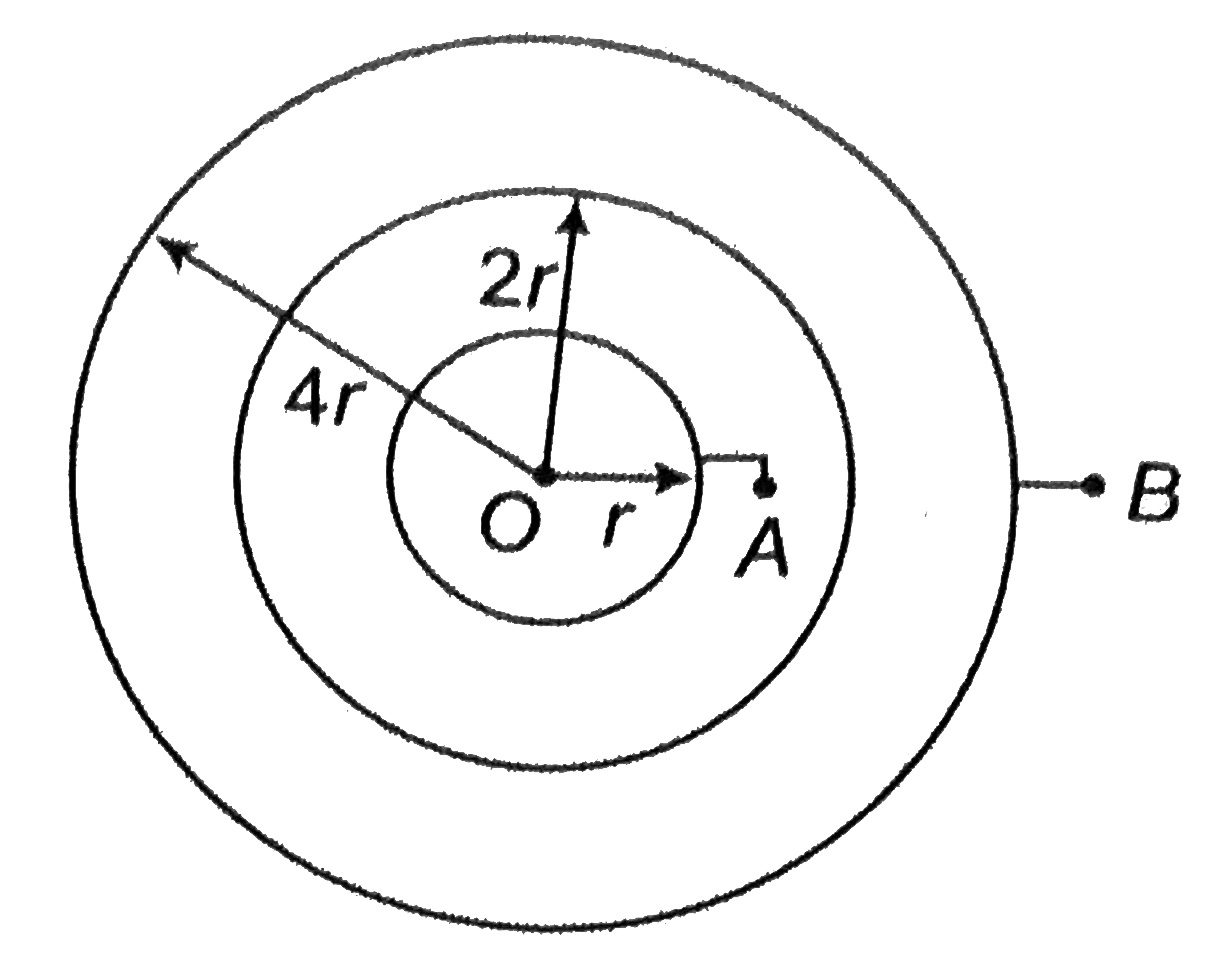
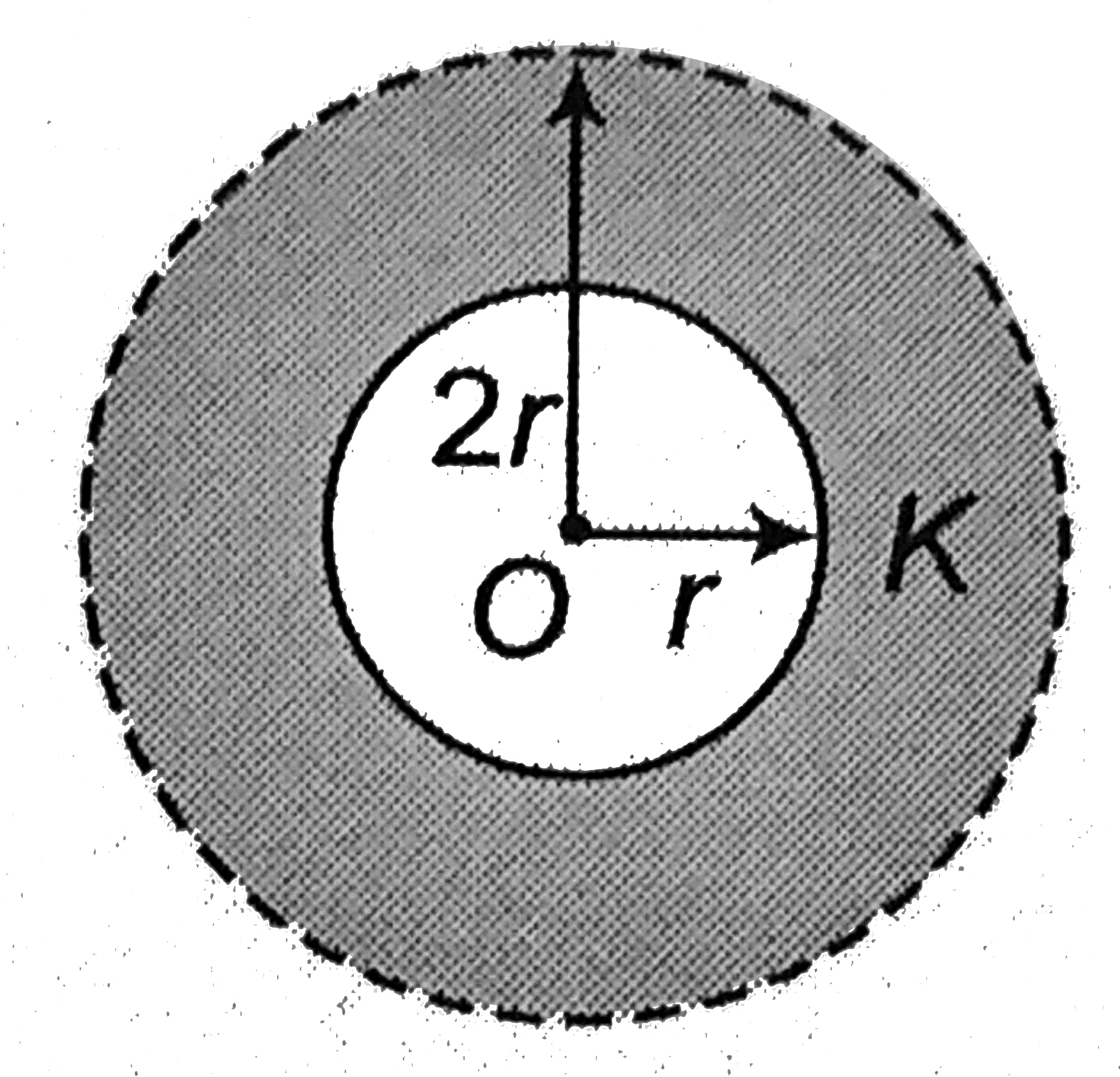

(a) Two capacitors,
Capacitor 1,C1=4π∈0r.2r(2r−r)=8π∈0r
Capacitor 2,C2=4π∈02r.3r(3r−2r)=24π∈0r
connected a battery between A and B, and check polarity, +,−,+,−,.
C=C1C2C1+C2=+π∈0r
(b) capacitor 1:
C1=4π∈0K.r.2r2r−r=8π∈0Kr
Capacitor 2: Isolated sphere of radius 2r and outer radius infinite.
C2=4π∈02r=8π∈0r
C1 and C2 in series, as shown in (a)
C−(eq)=C1C2C1+C2=8π∈0KrK+1
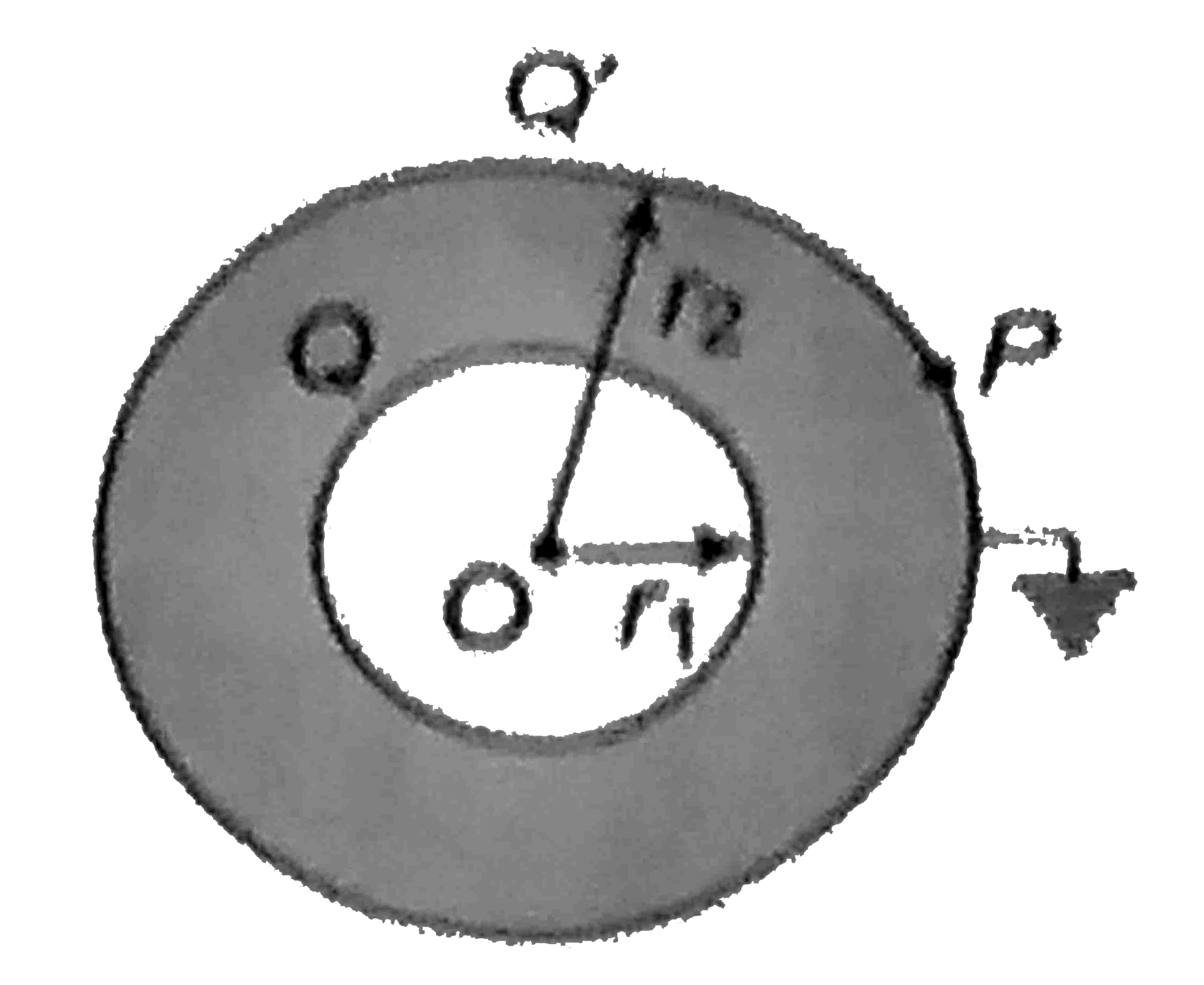
(c) (i) Let total charge on outer shell is Q'
Vp=14π∈0(Qr2+Q'r2)=0⇒Q'=−Q
C=4π∈0r1r2r20−r−(1)
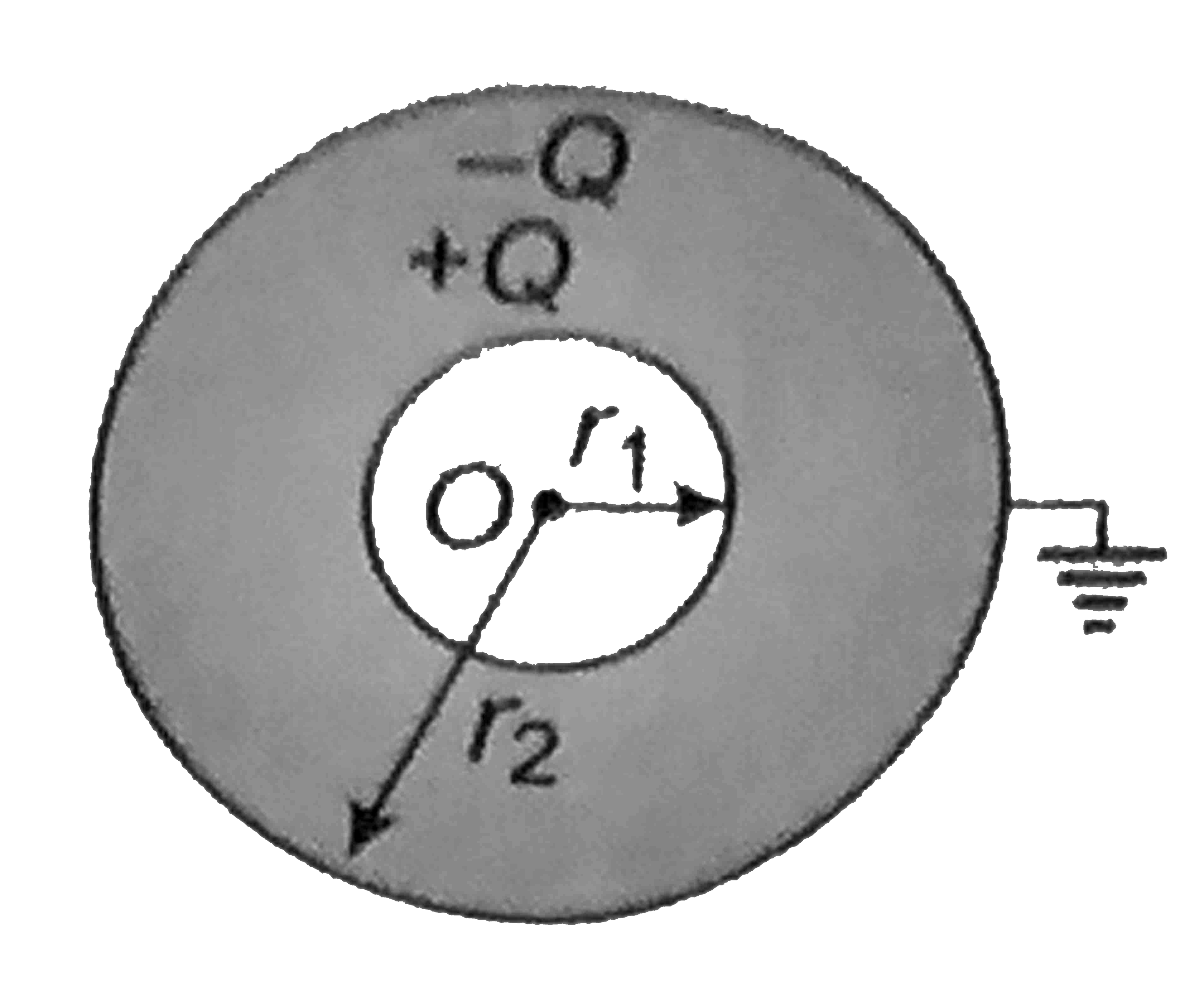
(ii) Let charge on inner shell be Q'.
Vp=14π∈0(Q'r1+Qr2)=0
Q'=−Qr1r2
This capacitor is combination of two capacitors
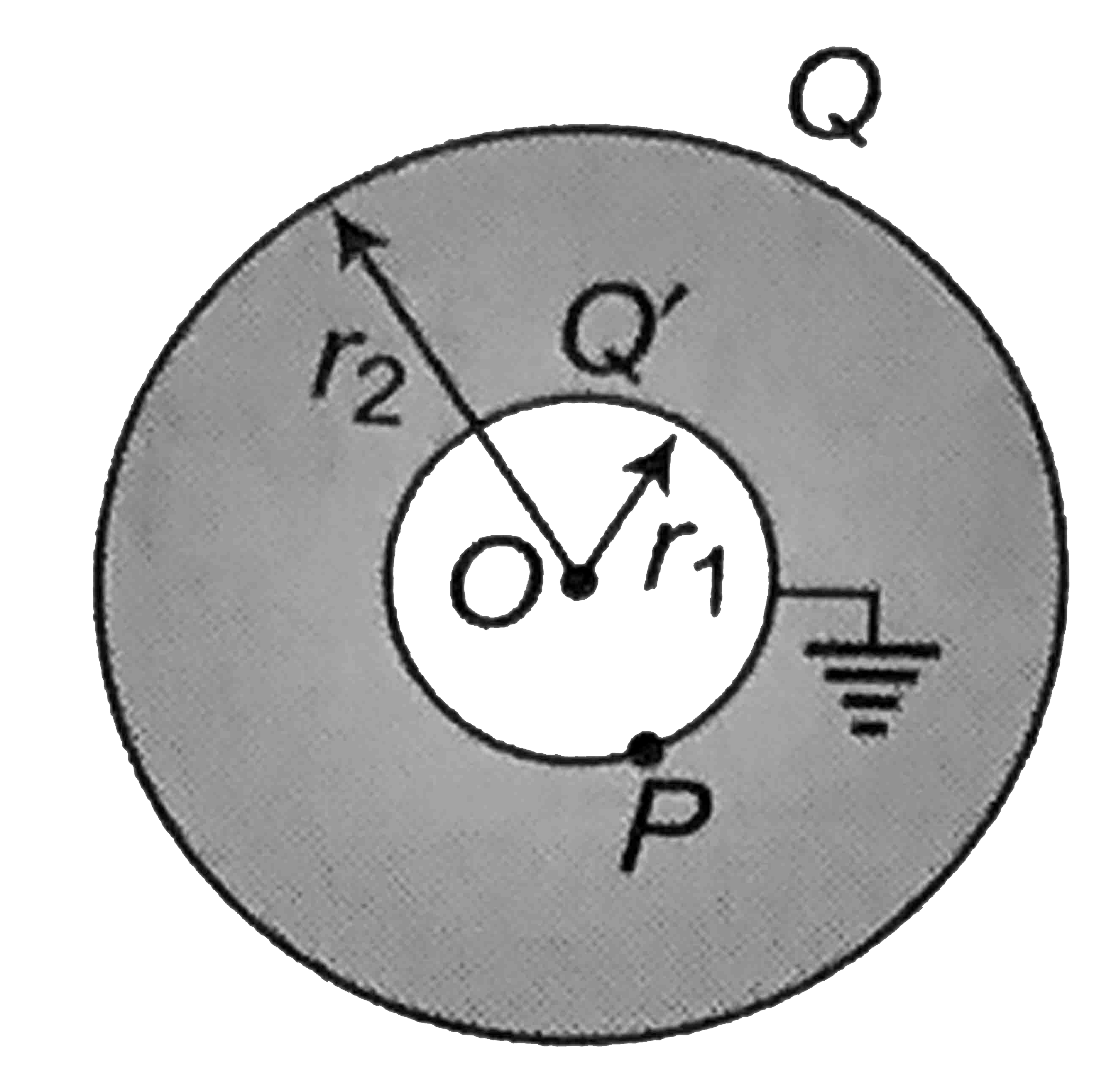
(i) capacitor 1,C1=4π∈0r1r2r2−r1
(ii) Capacitor 2, C2=4π∈0r2 (isolated spherical capacitor)
These capacitor are in parallel. the positive plates of capacitors are at one place. the −ve plate of capacitor 1 is connected to ground i.e., its potential is zero. the −ve plate of capacitor 2 is at infine i.e., its potential is zero. hence, positive plates at one point and negative plates at another point and hence C1 and C2 are in parallel.
Ceq=C1+C2=4π∈0r2(r1r2−r1+1)=4π∈0r22(r2−r1)
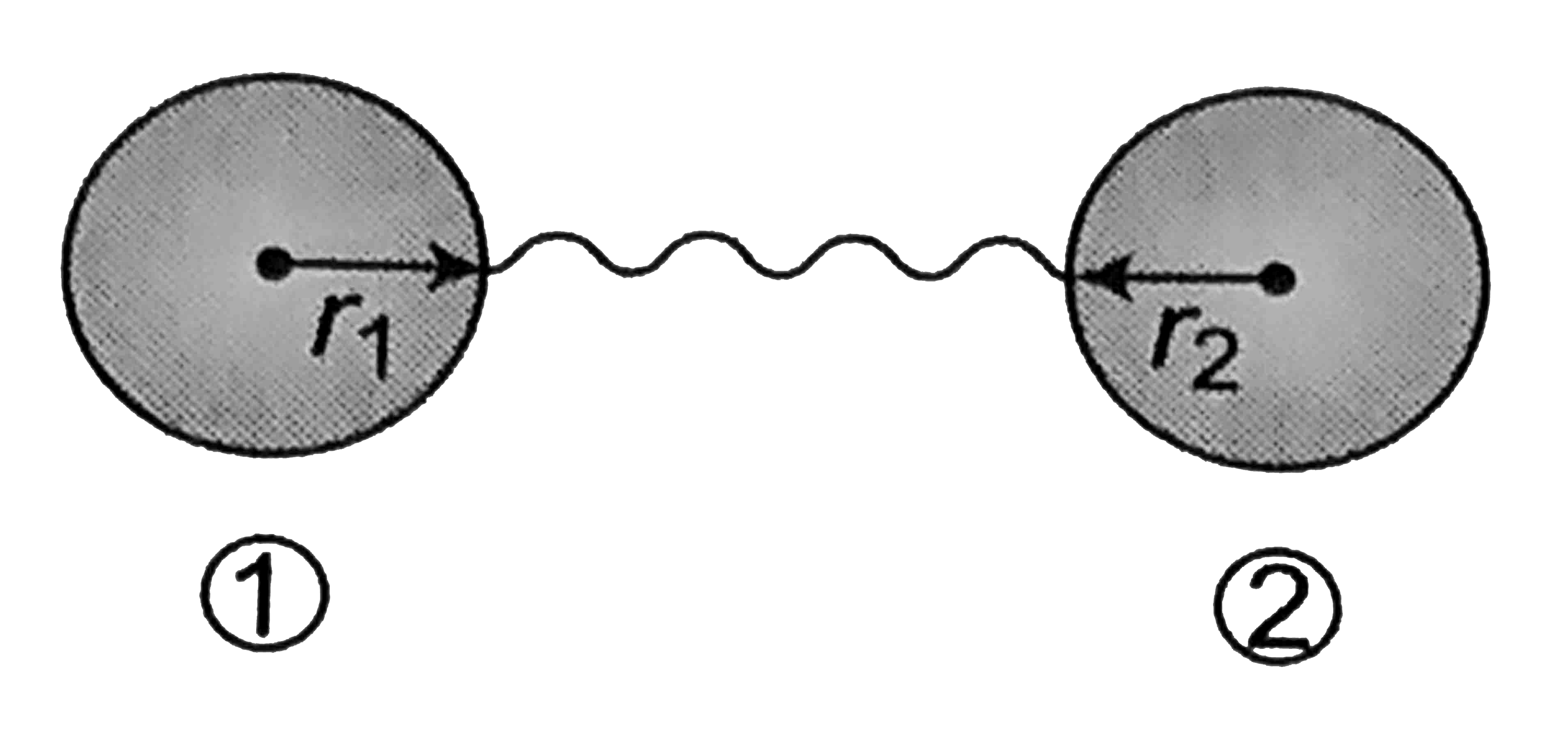
(d) (i) C1=4π∈0r1
C2=4π∈0r2
C1 and C2 are in parallel. their positive plates are connected as shown and negative plates are at infine.
Ceq=C1+C2=4π∈0(r1+r2)
(ii) Here, we will find capacitance with the help of energy. energy stored in a conductor,
U=Q22C
Energy of two point charges sepearated by r,
U'=14π∈0Q1Q2r so potential energy of system
U=U1+U2+U12
=Q22C1+Q22C2+14π∈0(Q)(−Q)d
=Q22×4π∈0r1+Q22×4π∈0r2−Q2(4π∈0d)
=Q28π∈0(1r1−1r2−2d) .....(i)
For a system, U=Q22Ceq
Equating (i) and (ii), we get
1Ceq=14π∈0(1r1+1r2−2d)
Ceq=4π∈0(1r1+1r2−2d)
If d→∞,Ceq=4π∈01r1+1r2
1Ceq=14π∈0r1+14π∈0r2=1C1+1C2
i.e., the given system is equivalent to two capacitors C1=4π∈0r1 and C2=4π∈0r2 in series.
 .
.
 .
.
 .
.

 .
.
Topper's Solved these Questions
Similar Questions
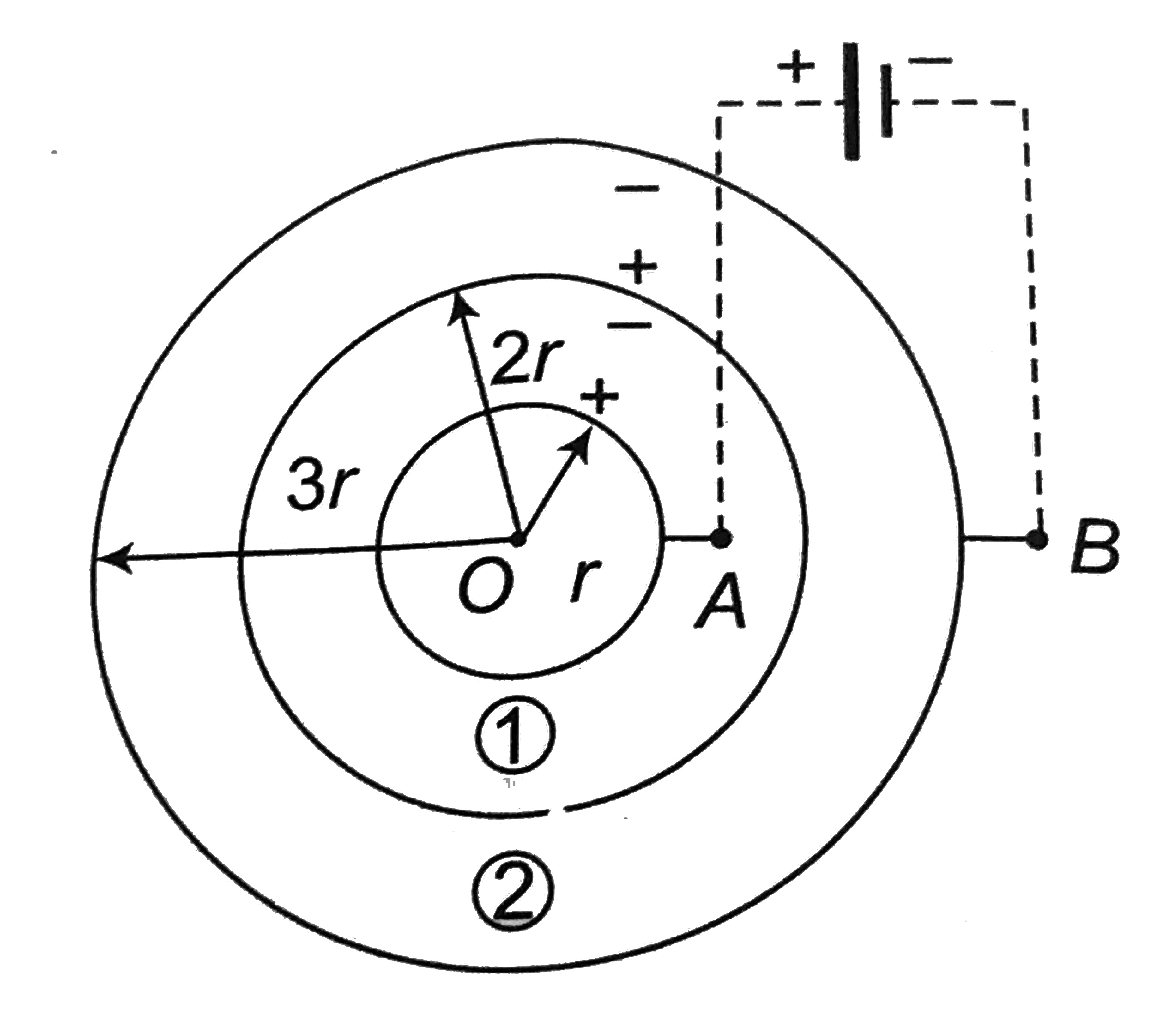
Find the equivalent capacitance between A and B
(i)
Three conducting concentric shells of radii r,2r,4r
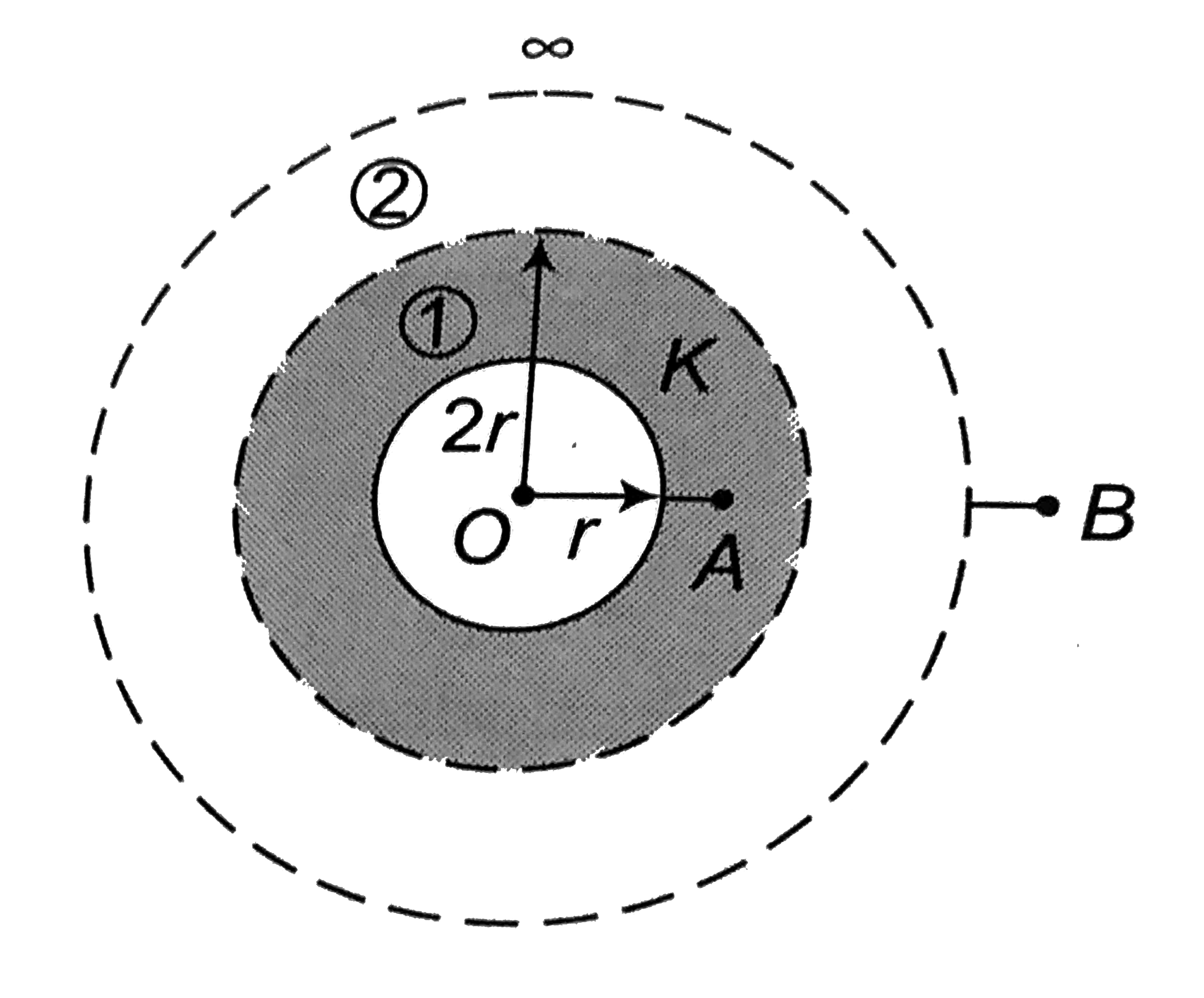
(ii)
An isolated ball-shaped conductor of radius r surrounded by an adjacent concentrc layer of dielectric (K) and outer radius 2r.
View SolutionConsider two concentric spherical metal shells of radii r1 and r2(r2>r1). Find the charge on the inner shell and charge distribution.
View Solution
Knowledge Check
Consider two concentric spherical metal shells of radii r1 and r2(r2>r1). If the outer shell has a charge q and the inner one is grounded, then the charge on the inner shell is
A−r2r1qBzeroC−r1r2qD−qThe radii of two concentric spherical conducting shells are r1andr2(>r1). The change on the oute shell is q. The charge on the inner shell which is connected to the earth is
Aq(r2r1)Bq2(r1r2)C−q(r1/r2)Dq2(r2r1)There are two concentric spherical shells with inner shell having positive charge q and outer shell having a charge -q. Now outer shell is supplied a charge -2g and inner shell is earthed, Calculate the ratio of initial and final capacities of the system. (If a = 3R, b= 5 R)
 A3/5B3C1D5/3
A3/5B3C1D5/3
Find the potential of an isolated ball-shaped conductor with a charge q of radius R1 surrounded by an adjacent concentric layer of dielectric with dielectric constant k and outer radius R2
View SolutionConsider two concentric spherical metal shells of radii r1 and r2(r2>r1). If the outer shell has a charge q and the inner one is grounded, then the charge on the inner shell is
View SolutionThe radii of two concentric spherical conducting shells are r1andr2(>r1). The change on the oute shell is q. The charge on the inner shell which is connected to the earth is
View SolutionCharge Q is given to a spherical conductor of radius R. It is surrounded by a concentric conducting shell of inner radius a and outer radius b. Corresponding electric field diagram will be:
View SolutionTwo concentric conducting shells of radii R and 2R are having charges Q and -2Q respectively.In a region r
View Solution
