Find the final temperature of a cooper rod. Whose area of cross section changes from 10m2 to 11m2 due to heating. The copper rod is initially kept at 90K. (Coefficient of superficial expansion is 0.0021/K)Solution in Tamil
Given: Area of copper rod A0=A1=10m2
Changes of area cross section ΔA=A2−A1
=11−10=1m2
nitial temperature t1=90K
ΔT=t2−t1
ΔT=t2=90
Coefficient of superficial expansion αA=0.0021/K
Then t2=?
αA=ΔAA0Δt
0.0021=110×(t2−90)⇒t2=90=10.0021×10
t2−90=10.021
t2=10.021+90
t2=137.6K
Topper's Solved these Questions
- BOOK - FULL MARKSCHAPTER - THERMAL PHYSICS EXERCISE - TEXTUAL EVALUATION -VIII (Answer in detail)2videos
THERMAL PHYSICS
- BOOK - FULL MARKSCHAPTER - THERMAL PHYSICS EXERCISE - TEXTUAL EVALUATION - XI (HOT question)1videos
THERMAL PHYSICS
- BOOK - FULL MARKSCHAPTER - THERMAL PHYSICS EXERCISE - TEXTUAL EVALUATION -VI (Answer in brieifly)8videos
THERMAL PHYSICS
- BOOK - FULL MARKSCHAPTER - SAMPLE PAPER-9EXERCISE - PART-IV6videos
SAMPLE PAPER-9
Similar Questions
Derive the ideal gas equation by combining the empirical gas laws.
02:39View SolutionIf you keep lee at 0CC and water at 0∘C in either of your hands, in which hand you will feel more chillness? Why?
01:10View SolutionDefine one calorie.
04:23View SolutionDefine one roentgen.
03:42View SolutionA heat flux of 4000 J/s is to be passed through a copper rod of length 10 cm and area of cross section 100cm2. The thermal conductivity of copper is 400W/m/∘C The two ends of this rod must be kept at a temperature difference of
04:11View SolutionWhen a rod is heated, its linear expansions depends on (a) initial length (b) area of cross section (c) mass (d) temperature rise
03:16View Solutionचित्र 11.15 में दर्शाए गए निकाय की स्थाई अवस्था में स्टील - ताँबा संधि का ताप क्या है ? स्टील छड़ की लंबाई = 15.0 cm, ताँबे की छड़ की लंबाई = 10.0 cm, भट्ठी का ताप = 300∘C, दुसरे सिरे का ताप = 0∘C , स्टील की छड़ की अनुप्रस्थ काट के क्षेत्रफल ताँबे की छड़ की अनुप्रस्थ काट के क्षेत्रफल का दो गुना है | (स्टील की ऊष्मा चालकता = 50.2 J s−1m−1K−1, ताँबे की ऊष्मा चालकता = 385Js−1m−1K−1)
04:16View SolutionA uniform metal rod of 2mm^(2) area of cross section is heated from 0^(@)C to 20^(@)C . The coefficient of linear expansion of the rod is 12xx10^(-6)//""^(@)C . Its Young's modulus of elasticity is 10^(11)N//m^(2) , then the energy stored per unit volume of rod is,
05:07View SolutionA heat flux of 4000 J/s is to be passed through a copper rod of length 10 cm and area of cross-section 100cm2. The thermal conductivity of copper is 400 W/m/ °C. The two ends of this rod must be kept at a temperature difference of
07:19View SolutionFind the final temperature of a cooper rod. Whose area of cross section changes from 10m2 to 11m2 due to heating. The copper rod is initially kept at 90K. (Coefficient of superficial expansion is 0.0021/K)
05:48View Solution(a) Find the final temperature of a copper rod. Whose area of cross section changes from 10m2 to 11m2 due to heating. The copper rod is initially kept at 90K. (Coefficient of superficial expansion is 0.0021/K).
(b) The far position of a myopic person is 90 cm is front of the eye. what is the nature and power of the lens required to correct the problem?03:52View SolutionA copper rod and a steel rod maintain a difference in their lengths of 10 cm at all temperature . If their coefficients of expansion are 1.6×10−5K−1and1.2×10−5K−1 , then length of the copper rod is
03:16View Solutionचित्र में दर्शाये गये निकाय की स्थायी अवस्था में स्टील तांबा संधि का माप क्या है? स्टील छड़ की लम्बाई =15.0cm तांबे की छड़ की लम्बाई =10.0cm भट्टी का ताप =300∘C, दूसरे सिरे का ताप =0∘C, स्टील की छा़ की अनुप्रस्थ काट का क्षेत्रफल तांबे की छड़ की अनुप्रस्थ काट के क्षेत्रफल का दोगुना है। स्टील की ऊष्मा चालकता =50.2JS−1m−1K−1, तांबे की ऊष्मा चालकता =385JS−1m−1K−1
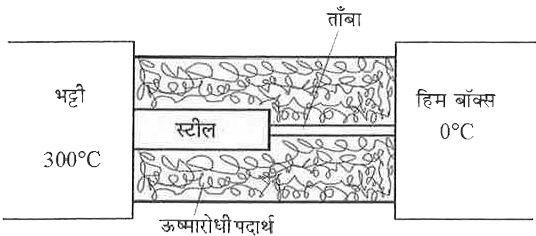 02:19View Solution
02:19View SolutionA Steel rod of length 25 cm has a cross - sectional area of 0.8cm2. The force required to stretch this rod by the same amount as the expansion produced by heating it through 10∘C is (coefficient of linear expansion of steel is 10−5/∘C and Young's modulus of steel is 2×1010N/m2).................... .
01:41View SolutionThere is a change in length when a 33000 N tensile force is applied on a steel rod of area of cross - section 10^(-3) . The change of temperature required to produce the same elongation, if the steel rod is heated, is (modulus of elasticity of steel =3xx10^(11)N//m^(2) , coefficient of linear expansion of steel =1.1xx10^(-5)//^(@)C ........................
03:18View Solution

