Find the molecular weight for a gas in which the velocity of sound is 1260ms at 0∘C and whose γ is 1.4.
To find the molecular weight of the gas, we will use the formula for the velocity of sound in a gas:
V=√γRTM
Where:
- V is the velocity of sound,
- γ is the adiabatic index (given as 1.4),
- R is the universal gas constant (approximately 8.31J/(mol K)),
- T is the absolute temperature in Kelvin,
- M is the molecular weight of the gas.
Step 1: Convert the temperature from Celsius to Kelvin
The temperature is given as 0∘C. To convert this to Kelvin, we use the formula:
T(K)=T(°C)+273.15
So,
T=0+273.15=273.15K≈273K(for simplicity)
Step 2: Rearrange the formula to solve for M
We can rearrange the formula for the velocity of sound to solve for the molecular weight M:
M=γRTV2
Step 3: Substitute the known values into the equation
Now we substitute the known values into the equation:
- γ=1.4
- R=8.31J/(mol K)
- T=273K
- V=1260m/s
Substituting these values gives:
M=1.4×8.31×273(1260)2
Step 4: Calculate the numerator and denominator
First, calculate the numerator:
1.4×8.31×273≈1.4×2270.83≈3189.162
Next, calculate the denominator:
(1260)2=1587600
Step 5: Calculate M
Now we can calculate M:
M=3189.1621587600≈0.002008kg
Step 6: Convert to grams
To convert kilograms to grams, we multiply by 1000:
M≈0.002008×1000≈2.008grams
Final Answer
The molecular weight of the gas is approximately 2grams.
---
- V is the velocity of sound,
- γ is the adiabatic index (given as 1.4),
- R is the universal gas constant (approximately 8.31J/(mol K)),
- T is the absolute temperature in Kelvin,
- M is the molecular weight of the gas.
The temperature is given as 0∘C. To convert this to Kelvin, we use the formula:
We can rearrange the formula for the velocity of sound to solve for the molecular weight M:
Now we substitute the known values into the equation:
- R=8.31J/(mol K)
- T=273K
- V=1260m/s
First, calculate the numerator:
Now we can calculate M:
To convert kilograms to grams, we multiply by 1000:
The molecular weight of the gas is approximately 2grams.
Topper's Solved these Questions
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Exercise 6.2
72 Videos
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Subjective
56 Videos
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Examples
56 Videos
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Interger
2 Videos
- SUPERPOSITION AND STANDING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Comprehension Type
5 Videos
Similar Questions
Find the temperature at which the velocity of sound in air will be 112 times of the velocity at 27∘C.
View SolutionFind the temperature at which the velocity of sound in air is double the velocity of sound in air at 0∘C.
[Hint : Use CC0=√TT0]
View SolutionIf cs is the velocity of sound in air and c is rms velocity , then
View SolutionCalculate the molecular weight of a gas X which diffuses four times as fast as another gas Y, which in turn diffuses twice as fast as another Z. Molecular weight of the gas Z is 128.
View SolutionCalculate the molecular weight of a gas which diffuses four times as fast as another gas Y, which in turn diffuses twice as fast as another gas Z [All under identical conditions]. Molecules weight of Z is 128.
View Solution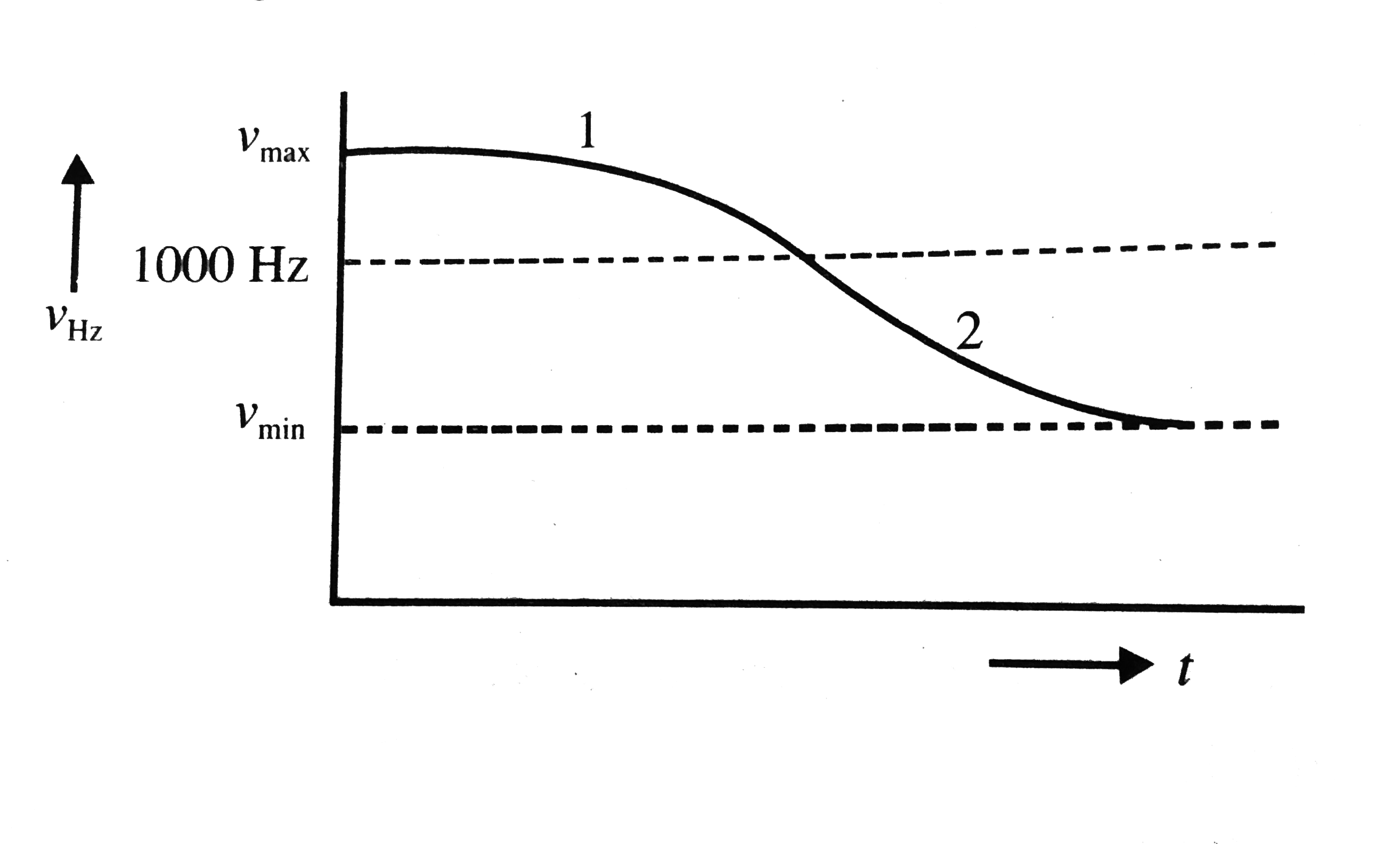
A stationary observer receives a sound from a sound of freqeuency v0 moving with a constant velocity vS=30ms The apparent frequency varies with time as shown in figure. Velocity of sound v=300ms. Then which of the following is incorrect?
View Solution
A stationary observer receives a sound from a sound of freqeuency v0 moving with a constant velocity vS=30ms The apparent frequency varies with time as shown in figure. Velocity of sound v=300ms. Then which of the following is incorrect?
View SolutionCalculate the increase in velocity of sound for 1∘C rise of temperature if the velocity of sound at 0∘C is 332m/s.
View SolutionFind the velocity of sound in O2 at NTP. Given that the velocity of sound in the H2 at NTP is 1880ms−1 the density of oxygen is 16 times the density of hydrogen γ=1.4 for both the gases.
View SolutionThe molecules of a given mass of gas have an rms velocity of 200ms−1 at 27∘C and pressure 1 atm. When the temperature is 127∘C and pressure is 2 atm, the rms velocity in ms−1 will be ?
View Solution
