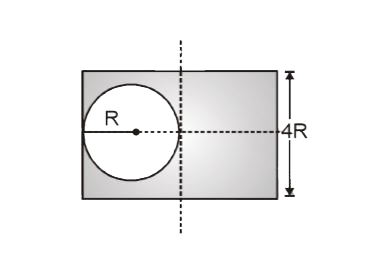
Find the position of centre of mass of the uniform lamina shown in figure.

Video Solution
Text Solution
Here A1 = area of complete circle πa2
A2= area of small circle =π(a2)2=πa24
(x1,y1)= coordinates of the centre of mass of the large circle
=(0,0)
(x2,y2)= coordinates of centre of mass of the small circle
=(a2,0)
Using xCM=(A1x1−A2x2)(A1−A2) we get
xCM=πa2×0−πa24×a2πa2−πa23=−a6
yCM=(as y1 and y2 both are zero)
Therefore coordinates of CM of the lamina shown in figure are (−a/6,0)
|
Topper's Solved these Questions
- CENTRE OF MASSBOOK - CENGAGE PHYSICSCHAPTER - CENTRE OF MASSEXERCISE - Solved Examples
13 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICSCHAPTER - CENTRE OF MASSEXERCISE - Exercise 1.1
20 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICSCHAPTER - CALORIMETRYEXERCISE - Solved Example
13 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICSCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
Similar Questions
Knowledge Check
Find the position of center of mass of the uniform lamina shown in figure, if small disc of radius a2 is cut from disc of radius a.
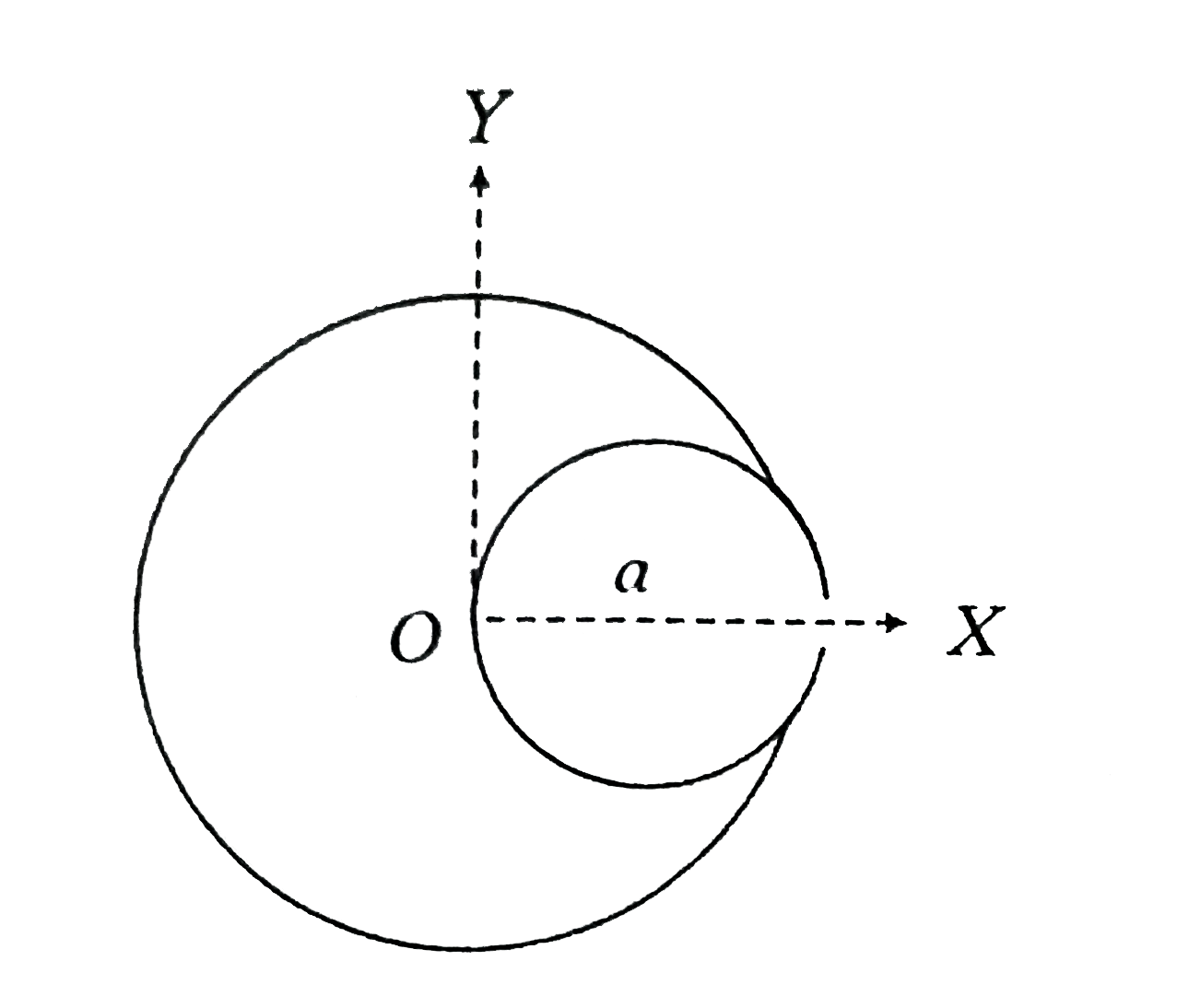 A(−a6,a)B(−a2,0)C(−a6,0)D(−a3,0)
A(−a6,a)B(−a2,0)C(−a6,0)D(−a3,0)Which of the following points is the likely position of the centre of mass of the system shown in figure?
 AA pencilBBCCDD
AA pencilBBCCDDFind the x coordinate of the centre of mass of the bricks shown in figure :
 A2425lB2524lC1516lD1615
A2425lB2524lC1516lD1615
Find the position of centre of mass of the uniform planner sheet shown in figure with respect to the origin (O) -
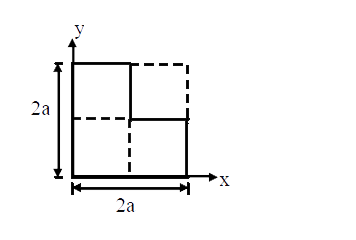
View SolutionFind the position of centre of mass of the section shown in figure

View SolutionFind the co-ordinates of centre of mass of the lamina shown in figure
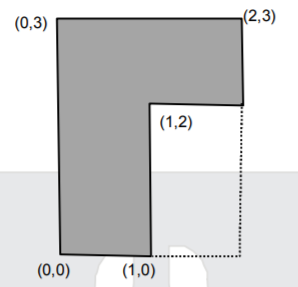
View SolutionFind out the position of centre of mass of the figure shown below.
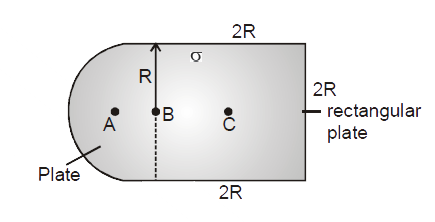
View SolutionLocate the position of centre of mass of a uniform plate of Mass m as shown in fig 4E 34

View Solution