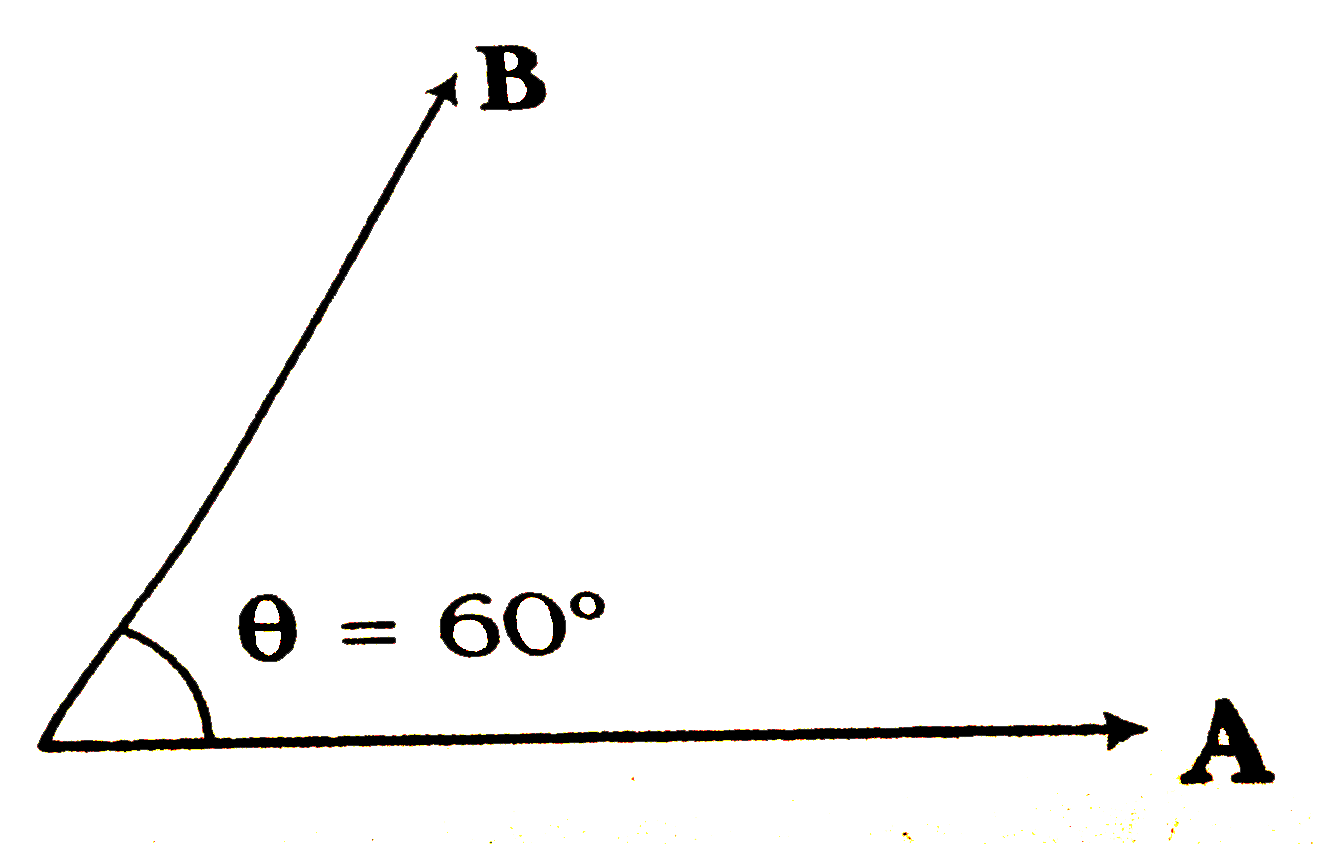
Find the sum ofvecctors A and B as shown in the figure.Also find the direction of sum vector. Given, A = 4 unit and B= 3 unit. K
A
√37unit,25.3∘
B
√2unit,36.8∘
C
5unit,70∘
D
√30unit,50.2∘
Text Solution
(a) According to the question, we draw the following figure.

Resultant of the vectors A and B,
R=√A2+B2+2ABcosθ
=√16+9+2×4×3cos60∘√37 unit
∴ Direction of the sum vectors A and B
tanα=BsinθA+Bcosθ=3sin60∘4+3cos60∘=0.472
∴ α=tan1(0.472)=25.3∘
Thua, resultant of |A| and |B|is √37 unit at angle 25.3∘ form A in the directionshownin figure above.
|
Topper's Solved these Questions
- SCALARS AND VECTORS BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - SCALARS AND VECTORS EXERCISE - Exercise 1
37 Videos
- SCALARS AND VECTORS BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - SCALARS AND VECTORS EXERCISE - Exercise 2 (Miscellaneous Problems)
20 Videos
- ROTATIONAL MOTION'BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - ROTATIONAL MOTION'EXERCISE - MHT CET Corner
35 Videos
- SEMICONDUCTORSBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - SEMICONDUCTORSEXERCISE - MHT CET Corner
25 Videos
Similar Questions
Knowledge Check
Find the sum of vector A and B as shown in the figure ,also find the direction of sum vector. Given A=4unit and B=3unit .
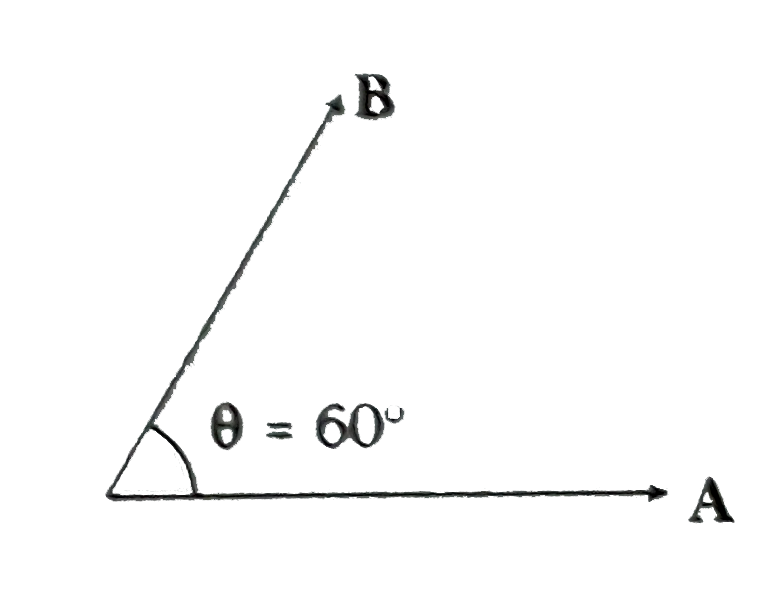 A√35unit , α=tan−1(0.472)=25.3∘B√25unit , α=tan−1(0.472)=25.3∘C√37unit , α=tan−1(0.472)=25.3∘D√37unit , α=tan−1(1)=45∘
A√35unit , α=tan−1(0.472)=25.3∘B√25unit , α=tan−1(0.472)=25.3∘C√37unit , α=tan−1(0.472)=25.3∘D√37unit , α=tan−1(1)=45∘Find the subraction of vector A and B as shown in the figure , also find the direction of subtraction vector ,Given A=4 unit and b=3 unit .
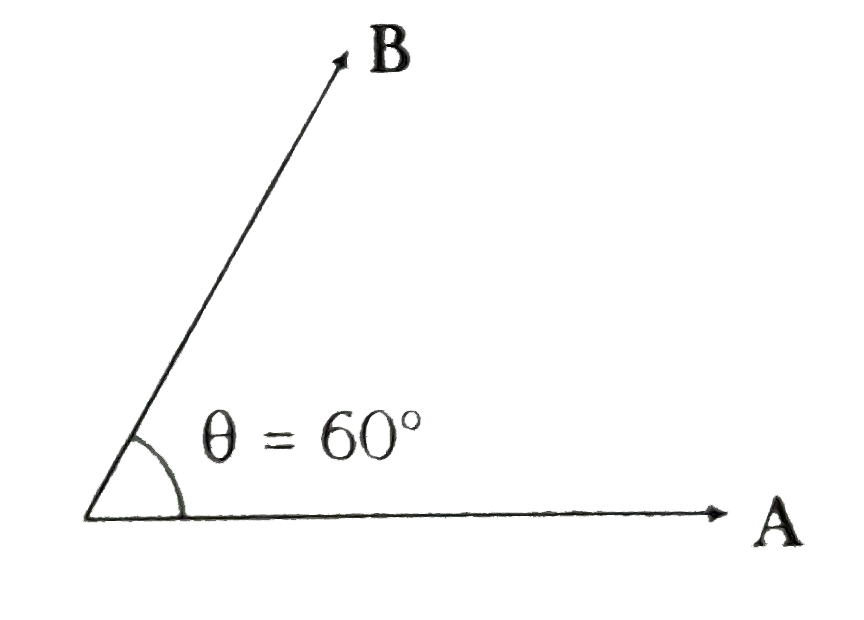 A=√19unit 60B=√24unit 45∘C=√17unit 46.1∘D=√13unit 46.1∘
A=√19unit 60B=√24unit 45∘C=√17unit 46.1∘D=√13unit 46.1∘Find A+B and A-B in the diagram shown in figure. Given A=4 units and B=3 units.
 .A√ 30,√ 22 unitsB√ 40,√ 28C√ 37,√ 15 unitsD√ 37,√ 13
.A√ 30,√ 22 unitsB√ 40,√ 28C√ 37,√ 15 unitsD√ 37,√ 13
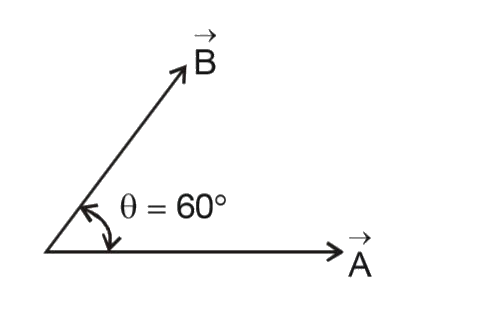
Find A+B and A-B in the diagram shown in figure. Given A=4 units and B=3 units.
 .
.
View SolutionFind →A+→Band→A−→B in the diagram shown in figure. Given A = 4 units and B = 3 units.
View SolutionFind the unit vector in the direction of sum of vectors →a=ˆ2i−ˆj+ˆk and →b=2ˆj+ˆk.
View SolutionFind the unit vector in the direction of sum of vectors →a=ˆ2i−ˆj+ˆk and →b=2ˆj+ˆk.
View SolutionIf the sum of two unit vectors is a unit vector,then find the magnitude of their differences.
View Solution
