Five identical charges, q1 each, are placed at the vertices of a regular pentagon having side length l1. The net electrostatic force on any of the charges due to other four is F1. Find the electrostatic force F2 on any one of the five identical charges, q2 each, placed at the vertices of a regular pentagon having side length l2.
The correct Answer is:F2=F1(q22l21q21l22)
Step by step video, text & image solution for Five identical charges, q_1 each, are placed at the vertices of a regular pentagon having side length l_1. The net electrostatic force on any of the charges due to other four is F_1. Find the electrostatic force F_2 on any one of the five identical charges, q_2 each, placed at the vertices of a regular pentagon having side length l_2. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- ELECTROSTATICSBOOK - ARIHANTCHAPTER - ELECTROSTATICSEXERCISE - Level 2
77 Videos
- ELECTROSTATICSBOOK - ARIHANTCHAPTER - ELECTROSTATICSEXERCISE - Level 3
59 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - ARIHANTCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Level 3
12 Videos
- GEOMETRICAL OPTICSBOOK - ARIHANTCHAPTER - GEOMETRICAL OPTICSEXERCISE - Level 3
9 Videos
Similar Questions
Five equal charges each of value q are placed at the corners of a regular pentagon of side a. The electric field at the centre of the pentagon is

View SolutionFive point charges, each +q are placed on five vertices of a regular hexagon of side' l '. Find the magnitude of the resultant force on a charge –q placed at the centre of the hexagon.
View Solution
Knowledge Check
Five point charge (+q each) are placed at the five vertices of a regular hexagon of side 2a.what is the magnitude of the net electric field at the centre of the hexazon?
A14πε0qa2Bq16πε0a2C√2q4πε0a2D5q16πε0a2Three point charges q are placed at three vertices of an equilateral triangle of side a. Find magnitude of electric force on any charge due to the other two.
A(√34πε0)(qa)2B(√24πε0)(qa)2C(√34πε0)(q2a)2D(√24πε0)(2qa)2Five identical point charges +Q are placed at the five corners of a regular hexagon of edge length a. One corner is empty. Electric field intensity at the centre of the hexagon is
AZeroBQ4πε0d2, towards the empty cornerCQ4πε0d2, away from the empty cornerD5Q4πε0a2 towards the empty corner
Six point masses of mass m each are at the vertices of a regular hexagon of side l. Calculate the force on any of the masses.
View SolutionFive point charge (+q each) are placed at the five vertices of a regular hexagon of side 2a.what is the magnitude of the net electric field at the centre of the hexazon?
View SolutionFive point charges each of charge + q are placed on five vertices of a regular of side h as shown in the figure. Then
View SolutionIf 8 identical charges of -g each are placed at the eight corners of a cube of side b, then electrostatic potential energy of a charge +q0 placed at the centre of the cube will be
View SolutionThree charge +q,−q, and +2q are placed at the vertices of a right angled triangle (isosceles triangle)as shown. The net electrostatic energy of the configuration is:
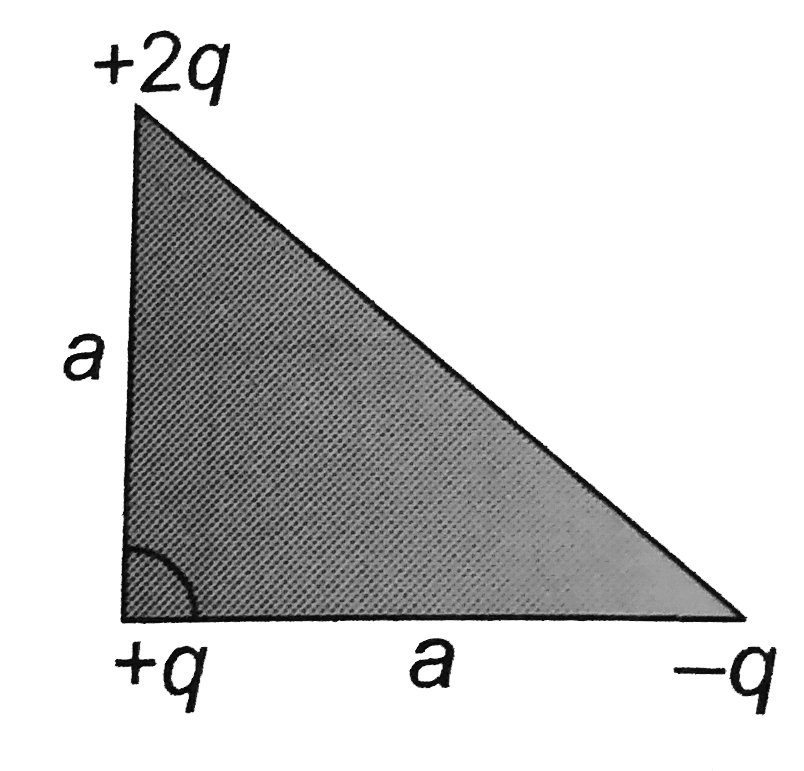
View Solution
