For a low altitude orbit if r≅rp, where rp is planet radius, show that for a given average planetary density, the orbital period of satellite is independent of the size of the planet. Calculate its value average density is ρ.
The correct Answer is:[√3πGρ]
Topper's Solved these Questions
- GRAVITATIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - GRAVITATIONEXERCISE - Discussion Qns
31 Videos
- GRAVITATIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - GRAVITATIONEXERCISE - Concetual MCQ
39 Videos
- GRAVITATIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - GRAVITATIONEXERCISE - Unsolved Numerical
94 Videos
- FLUID MECHANICSBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - FLUID MECHANICSEXERCISE - Unsolved Numerical Problems
86 Videos
- KINEMATICSBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - KINEMATICSEXERCISE - Unsolved Numerical Pro.
83 Videos
Similar Questions
" For a given density of planet,the orbital period of a satellite near the surface of the planet of radius "R" is proportional to "R^(N)" .Find the value of "Lambda
View SolutionThe density of the core of planet is ρ1 and that of the outer shell is ρ2. The radius of the core that of the planet are R and 2R respectively. Gravitastional intensity at the surface of the planet is same as at a depth R. Find the ratio ρ1ρ2 , assuming them to be uniform independently.
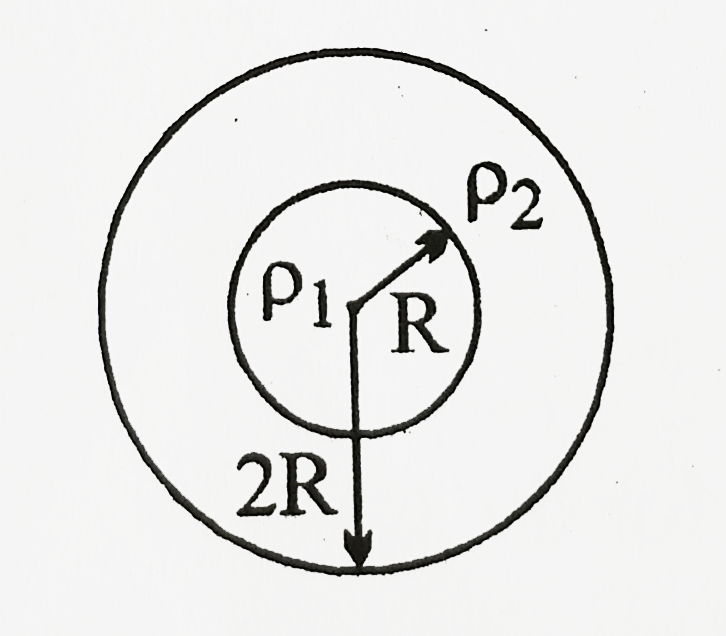
View Solution
Knowledge Check
The orbital period of sa satellite near the surface of the planet of radius R is given by (ρ is the density of the planet):
A3π(ρG)1/2B4π(3ρG)1/2C4π(ρG)1/2D3π(4ρG)1/2If ρ is the density of the planet , the time period of nearby satellite is given by
A√4π3GρB√4πGρC√3πGρD√πGρIf r represents the radius of the orbit of a satellite of mass m moving around a planet of mass M , the velocity of the satellite is given by
Av2=gMRBv2=GMmRCv=GMrDv2=GMr
If R denotes the radius of orbit of a satellite of mass m revolving around a planet of mass M, the sqiared orbital velocity of the satellite is given by
View SolutionThe period of revolution of a satellite in a low-altitude circular orbit around a spherical planet of uniform density ρ is
View SolutionIf the radius of a planet is R and its density is ρ , the escape velocity from its surface will be
View SolutionConsider a hypothetical planet which is very long and cylinderical. The density of the planet is ρ, its radius is R.
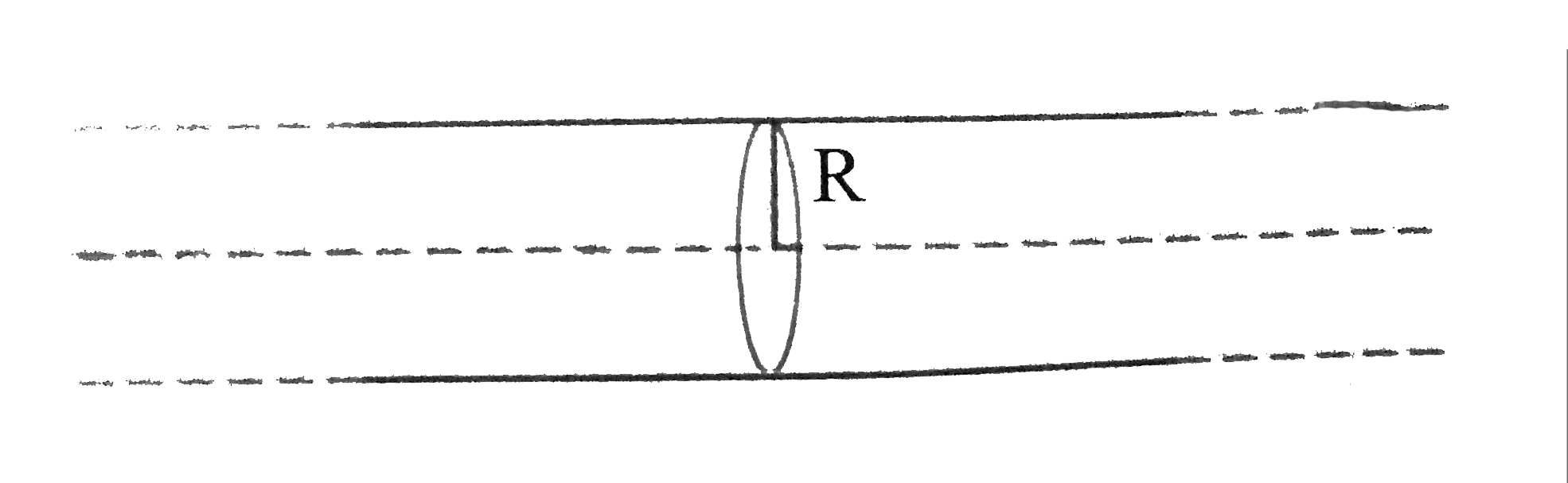
What is the possible orbital speed of the satellite in moving around the planet in circular orbit in a plane which is perpendicular to the axis of planet?
View SolutionThe average density and time period of a planet are ρ and T. A satellite is revolving just above the surface of planet. If the universal gravitational constant is G, then T2 is equal to
View Solution
