The density of the core of planet is $ρ_{1}$ and that of the outer shell is $ρ_{2}$ . The radius of the core that of the planet are R and 2R respectively. Gravitastional intensity at the surface of the planet is same as at a depth R. Find the ratio $\frac{ρ_{1}}{ρ_{2}}$ , assuming them to be uniform independently.

Video Solution

Text Solution

Verified by Experts

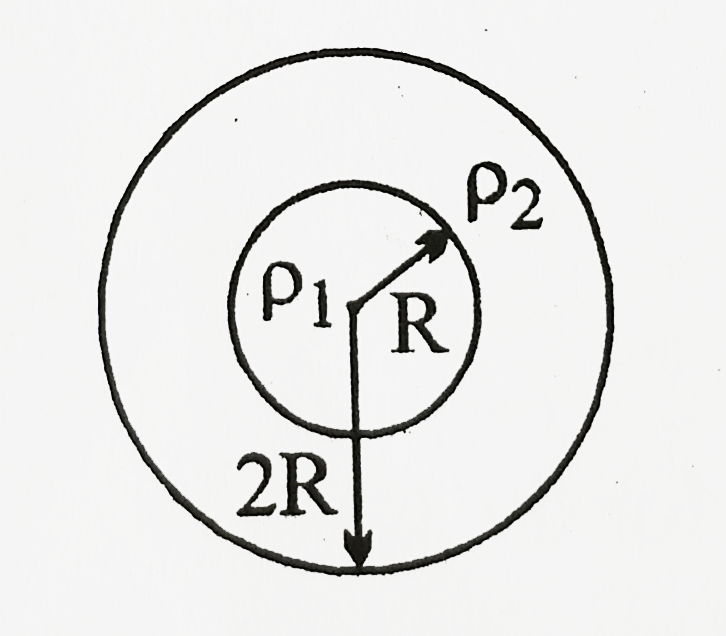
The correct Answer is: $7 / 3$

$\frac{G M_{core}}{R^{2}} = \frac{G M_{Total}}{4 R^{2}}, \frac{G \frac{4}{3} π R^{3} ρ}{R^{2}} = \frac{G \frac{4}{3} π R^{3} [ρ_{1} + 7 ρ_{2}]}{4 R^{2}}$
$4 ρ_{1} = ρ_{1} + 7 ρ_{2} \Rightarrow \frac{ρ_{1}}{ρ_{2}} = \frac{7}{3}$

|

Updated on:21/07/2023

Class 11 PHYSICS GRAVITATION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Suppose there are two planets, 1 and 2, having the same density but their radii are $R_{1} and R_{2}$ respectively, where $R_{1} > R_{2} .$ The accelerations due to gravity on the surface of these planets are related as
A $g_{1} > g_{2}$
B $g_{1} < g_{2}$
C $g_{1} = g_{2}$
DCan't say anything
Question 2 - Select One
The orbital period of sa satellite near the surface of the planet of radius R is given by ( $ρ$ is the density of the planet):
A $\frac{3 π}{{(ρ G)}^{1 / 2}}$
B $\frac{4 π}{{(3 ρ G)}^{1 / 2}}$
C $\frac{4 π}{{(ρ G)}^{1 / 2}}$
D $\frac{3 π}{{(4 ρ G)}^{1 / 2}}$
Question 3 - Select One
If the radius of a planet is R and its density is $ρ$ , the escape velocity from its surface will be
A $v_{e} \propto ρ R$
B $v_{e} \propto \sqrt{ρ} R$
C $v_{e} \propto \frac{\sqrt{ρ}}{R}$
D $v_{e} \propto \frac{1}{\sqrt{ρ} R}$

BANSAL-GRAVITATION-EXERCISE -3 [Miscellaneous Exercise]

Home
Ask Doubt
Profile