For angles of projection of a projectile at angle (45∘−θ)and(45∘+θ), the horizontal ranges described by the projectile are in the ratio of :
2:1
1:2
1:1
2:3
The correct Answer is:C
For angle (45∘−θ),R=u2sin(90∘−2θ)g=u2cos2θg
For angle (45∘+θ),R=u2sin(90∘+2θ)g=u2cos2θg
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - MOTIONEXERCISE - B. Medical entrance1videos
MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - MOTIONEXERCISE - Medical entrance12videos
MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - MOTIONEXERCISE - A. Taking it together1videos
MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - MEASUREMENT AND ERRORSEXERCISE - Subjective19videos
MEASUREMENT AND ERRORS
- BOOK - DC PANDEY ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - (C )Medical entrances gallery32videos
MOTION IN A PLANE
Similar Questions
For angles of projection of a projectile at angles (45∘ – θ) and (45∘+θ), the horizontal ranges described by the projectile are in the ratio of:
View SolutionTwo projectiles A and B are projected with angle of projection 150 for the projectile A and 450 for the projectile B. If RAandRB be the horizontal range for the two projectile then.
View Solution
Knowledge Check
- Question 1 - Select One
Galileo writes that for angles of projection of a projectile at angles (45+θ) and (45−θ), the horizontal ranges described by the projectile are in the ratio of (if θ≤45)
A2:1B1:2C1:1D2:3
An object is projected at an angle of 45∘ with the horizontal. The horizontal range and the maximum height reached will be in the ratio.
View SolutionTwo projectiles are projected angle (π4+θ) and (π4−θ) with the horizontal , where θ<π4, with same speed. The ratio of horizontal ranges described by them is
View SolutionFind the ratio of horizontal range and the height attained by the projectile. i.e. for θ=45∘
View SolutionA point P on the trajectory of a projectile projected at angle with θ with horizontal subtrends angles α and β at the point of landing them
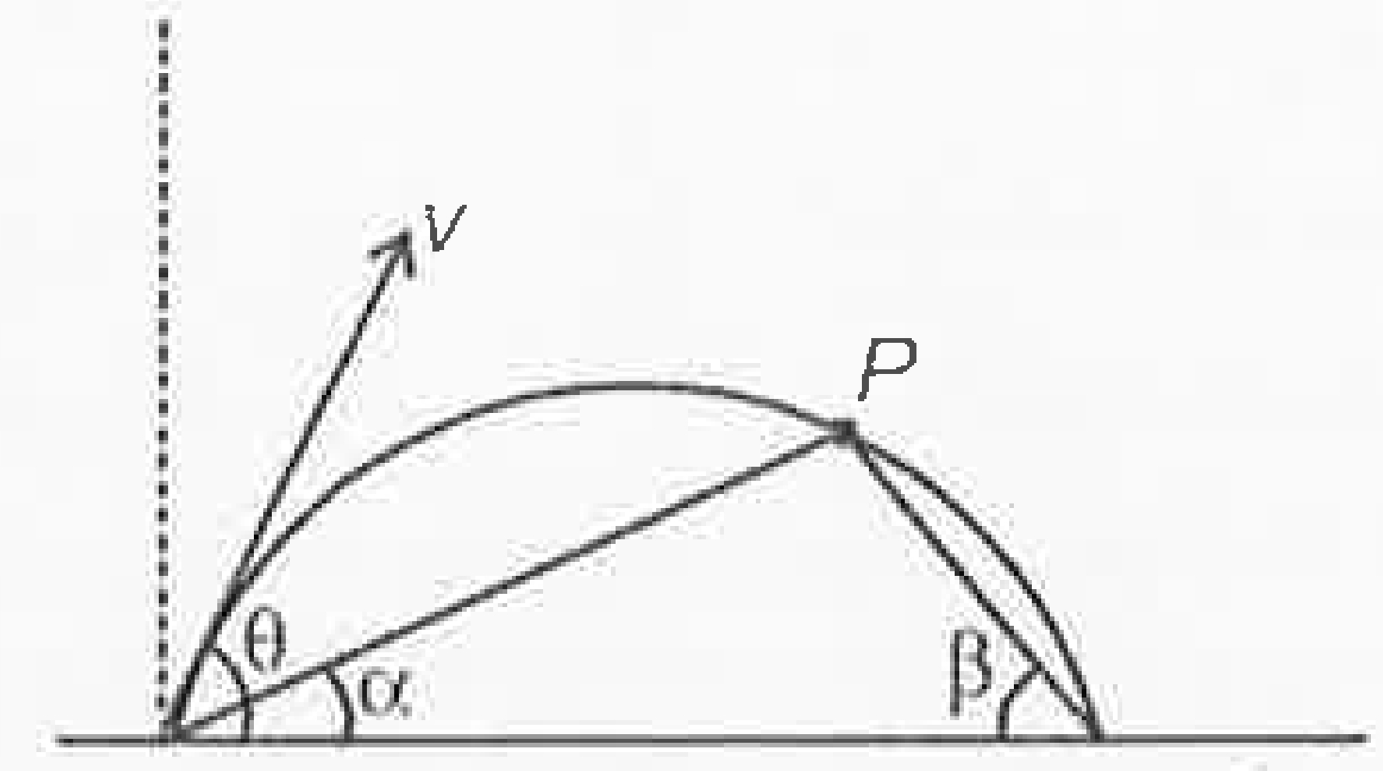 View Solution
View SolutionFour projectiles are projected with the same speed at angles 20∘,35∘,60∘and75∘
with the horizontal. The range will be the maximum for the projectile whose angle of projection isView SolutionThe range of a projectile, when launched at an angle of 15∘ with the horizontal is 1.5 km. what is the range of the projectile, when launched at an angle of 45∘ to the horizontal with the same speed ?
View SolutionA projectile is projected with speed u at an angle θ with the horizontal . The average velocity of the projectile between the instants it crosses the same level is
View Solution
