Four identical spheres each of mass $M$ and radius $R$ are placed on the vertices of a square of edge $L$ . Find the $M . I .$ about an axis
( $a$ ) coinciding with the side of a square and
( $b$ ) coinciding with the diagonal of a square.

Video Solution

Text Solution

Verified by Experts

(a)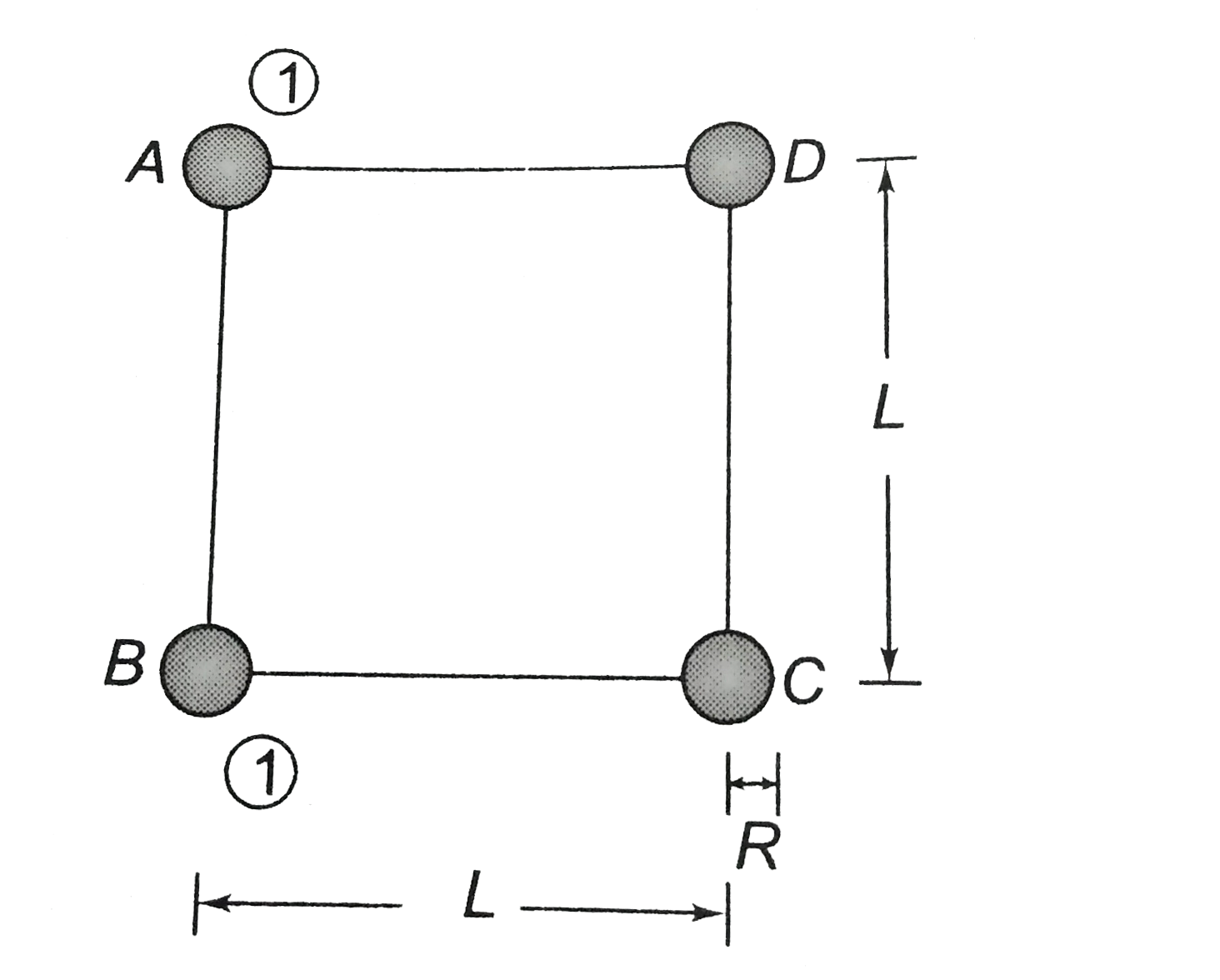
$I_{1 - 1} = ?$
For two spheres $A$ and $B$ , we have direct formulae and for $C$ and $D$ , we will use the parallel axes theorem
$I_{1 - 1} = I_{A} + I_{B} + I_{C} + I_{D}$
$M . I .$ of $A$ and $B$ about $1 - 1 = \frac{2}{5} M R^{2}$
$M . I .$ of $C$ and $D$ about $1 - 1$ is
$(I_{c . m}) + M d^{2} = \frac{2}{5} M R^{2} + M L^{2}$
$I_{1 - 1} = \frac{2}{5} M R^{2} \times 2 + (\frac{2}{5} M R^{2} + M L^{2}) \times 2$
$= \frac{8}{5} M R^{2} + 2 M L^{2}$
(b)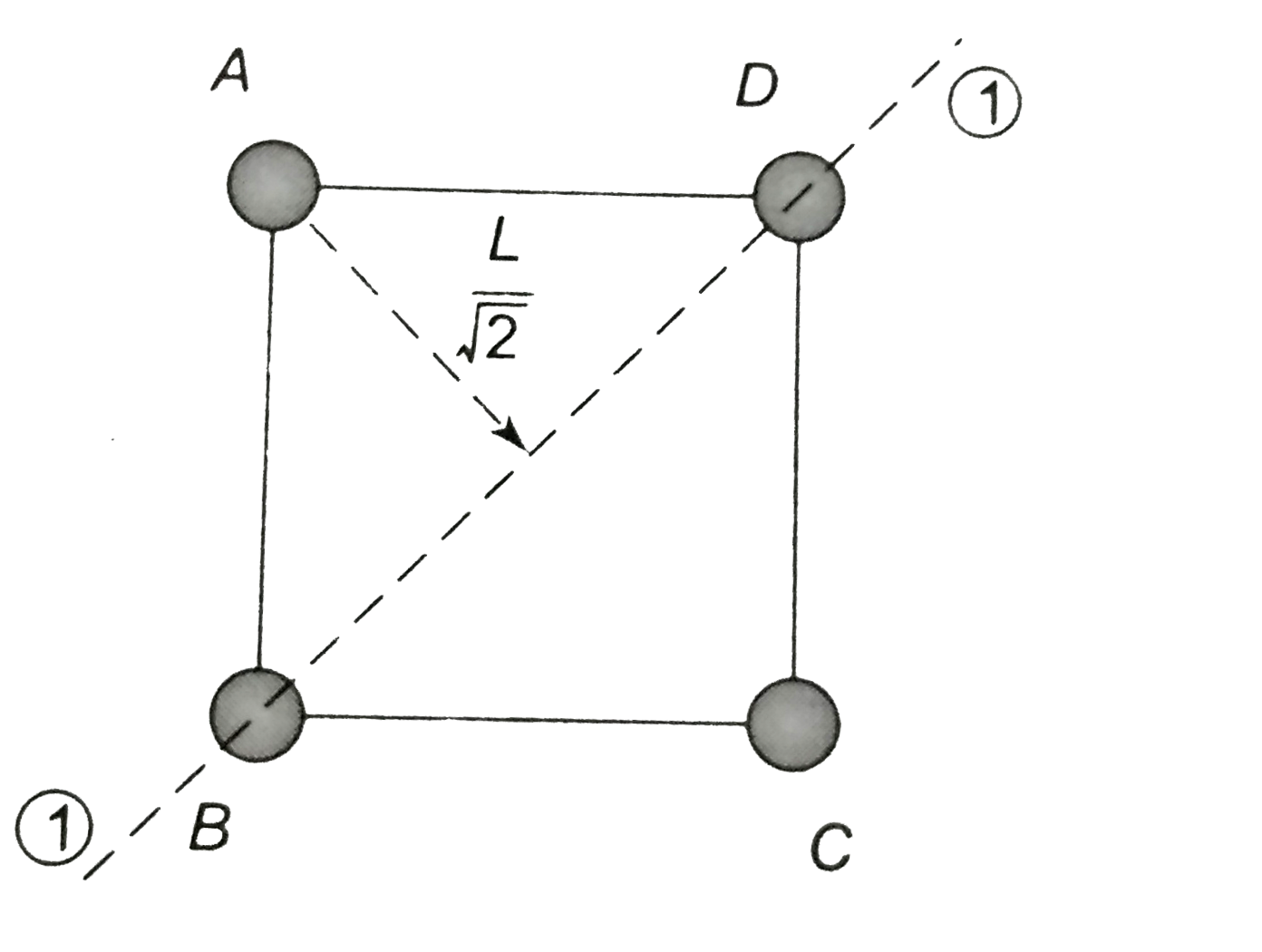
$I_{1 - 1} = I_{B} + I_{D} + I_{A} + I_{C}$
$= \frac{2}{5} M R^{2} \times 2 + ⎡ ⎣ \frac{2}{5} M R^{2} + M {(\frac{L}{\sqrt{2}})}^{2} ⎤ ⎦ \times 2$
$= \frac{8}{5} M R^{2} + M L^{2}$

Updated on:21/07/2023