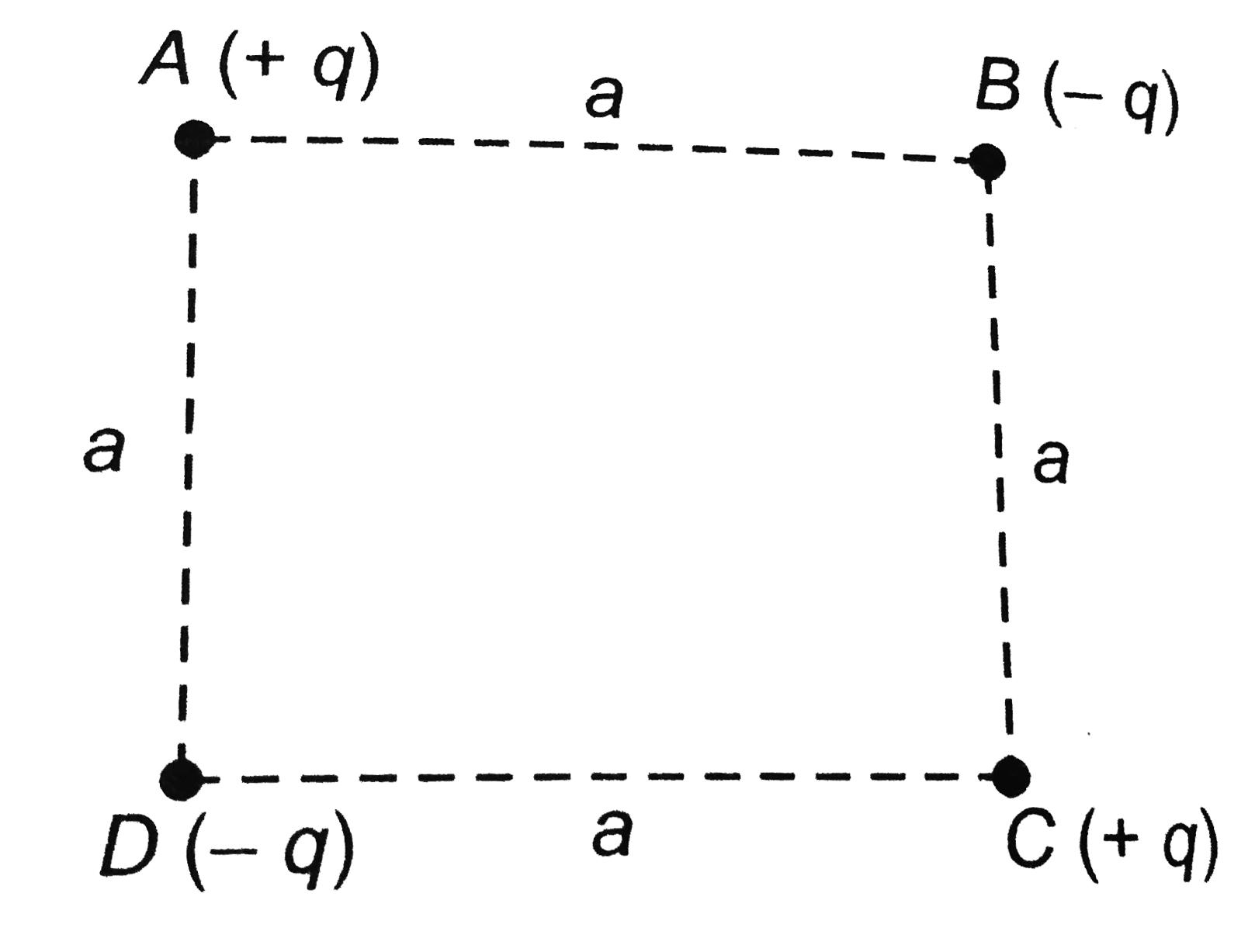
Four point charge q,−q,2Q and Q are placed in order at the corners A, B, C and D of a square. If the field at the midpoint of CD is zero then the value of q/Q is 5√5x. Find the value of x.
The correct Answer is:2
To solve the problem, we need to analyze the electric field at the midpoint of line segment CD in the square formed by the charges. Let's denote the corners of the square as follows:
- A (top left corner) has charge q
- B (top right corner) has charge −q
- C (bottom right corner) has charge 2Q
- D (bottom left corner) has charge Q
Step 1: Identify the Midpoint of CD
The midpoint M of line segment CD can be expressed in terms of coordinates. If we assume the square has a side length a, the coordinates of the corners are:
- C: (a,0)
- D: (0,0)
Thus, the coordinates of midpoint M are:
M=(a2,0)
Step 2: Calculate the Electric Field at M due to Each Charge
The electric field →E due to a point charge q at a distance r is given by:
→E=k⋅qr2^r
where k is Coulomb's constant and ^r is the unit vector pointing away from the charge.
Electric Field due to Charge at A (q):
- Distance from A to M: a√2
- Direction: towards the right (positive x-direction)
EA=k⋅q(a√2)2=2kqa2
Electric Field due to Charge at B (-q):
- Distance from B to M: a2
- Direction: towards the left (negative x-direction)
EB=−k⋅(−q)(a2)2=4kqa2
Electric Field due to Charge at C (2Q):
- Distance from C to M: a2
- Direction: towards the left (negative x-direction)
EC=−k⋅(2Q)(a2)2=−8kQa2
Electric Field due to Charge at D (Q):
- Distance from D to M: a2
- Direction: towards the right (positive x-direction)
ED=k⋅Q(a2)2=4kQa2
Step 3: Set Up the Equation for Zero Electric Field
For the electric field at point M to be zero, the sum of the electric fields due to all charges must equal zero:
EA+EB+EC+ED=0
Substituting the expressions we found:
2kqa2+4kqa2−8kQa2+4kQa2=0
This simplifies to:
6q−4Q=0
Thus:
6q=4Q⟹qQ=46=23
Step 4: Relate to Given Ratio
According to the problem, we have:
qQ=5√5x
Setting the two ratios equal gives:
23=5√5x
Cross-multiplying yields:
2x=15√5⟹x=15√52
Final Answer
Thus, the value of x is:
15√52
- B (top right corner) has charge −q
- C (bottom right corner) has charge 2Q
- D (bottom left corner) has charge Q
The midpoint M of line segment CD can be expressed in terms of coordinates. If we assume the square has a side length a, the coordinates of the corners are:
- C: (a,0)
- D: (0,0)
M=(a2,0)
The electric field →E due to a point charge q at a distance r is given by:
→E=k⋅qr2^r
where k is Coulomb's constant and ^r is the unit vector pointing away from the charge.
- Distance from A to M: a√2
- Direction: towards the right (positive x-direction)
EA=k⋅q(a√2)2=2kqa2
- Distance from B to M: a2
- Direction: towards the left (negative x-direction)
EB=−k⋅(−q)(a2)2=4kqa2
- Distance from C to M: a2
- Direction: towards the left (negative x-direction)
EC=−k⋅(2Q)(a2)2=−8kQa2
- Distance from D to M: a2
- Direction: towards the right (positive x-direction)
ED=k⋅Q(a2)2=4kQa2
For the electric field at point M to be zero, the sum of the electric fields due to all charges must equal zero:
EA+EB+EC+ED=0
Substituting the expressions we found:
2kqa2+4kqa2−8kQa2+4kQa2=0
This simplifies to:
6q−4Q=0
Thus:
6q=4Q⟹qQ=46=23
According to the problem, we have:
qQ=5√5x
Setting the two ratios equal gives:
23=5√5x
Cross-multiplying yields:
2x=15√5⟹x=15√52
Thus, the value of x is:
15√52
Topper's Solved these Questions
Similar Questions
Charges q,2q,3q and 4q are placed at the corners A,B,C and D of a square as shown in the following figure. The directon of electric field at the centre of the square is along

View SolutionFour point charges Q , q Q and q are placed at the corners of a square of side 'a' as shown in the Fig. Find the
potential energy of this system .
View Solution
Knowledge Check
Point charges g, -q, 2Q and Q are placed in order at the corners A, B, Cand D of a square of side 2b. If the field at the midpoint of CD is zero, then qQ is
A1B2C2√25D5√52q,2q, 3q and 4q charges are placed at the four corners A,B,C and D of a square. The field at the centre P of the square has the direction parallel to
 AABBCBCACDBD
AABBCBCACDBDThere are four point charges +q,−q,+q and −q are placed at the corners A,B,C and D respectively of a square of side a. The potential energy of the system is 14πε0 times
 Aq2a(−4+√2)Bq22a(−4+√2)C4q2aD−4√2q2a
Aq2a(−4+√2)Bq22a(−4+√2)C4q2aD−4√2q2a
Four charges +q,+2q,+3q and +4q are placed at the four corners ABCD of a square of side a. Calculate the field and potential at the centre of the square.
View SolutionFour point charges Q , q Q and q are placed at the corners of a square of side 'a' as shown in the Fig. Find the
resultant electric force on a charge Q
View SolutionFour point charges Q.q Q and q are placed at the corners of a square side 'a' as shown in the figures.

Find the Potential energy of this system.
View SolutionCharges +q,−q,+q and −q are placed at the corners A,B,C and D respectively of a square of side a. The potential energy of the system is 1/4πε0 times
View SolutionFour point charges −Q,−q,2q and 2Q are placed, one at each corner of the square. The relation between Q and q for which the potential at the centre of the square is zero is
View Solution
