From a high tower, at time t=0, one stone is dropped from rest and simultaneously another stone is projected vertically up with an initial velocity .The graph of distance S between the two stones plotted against time t will be
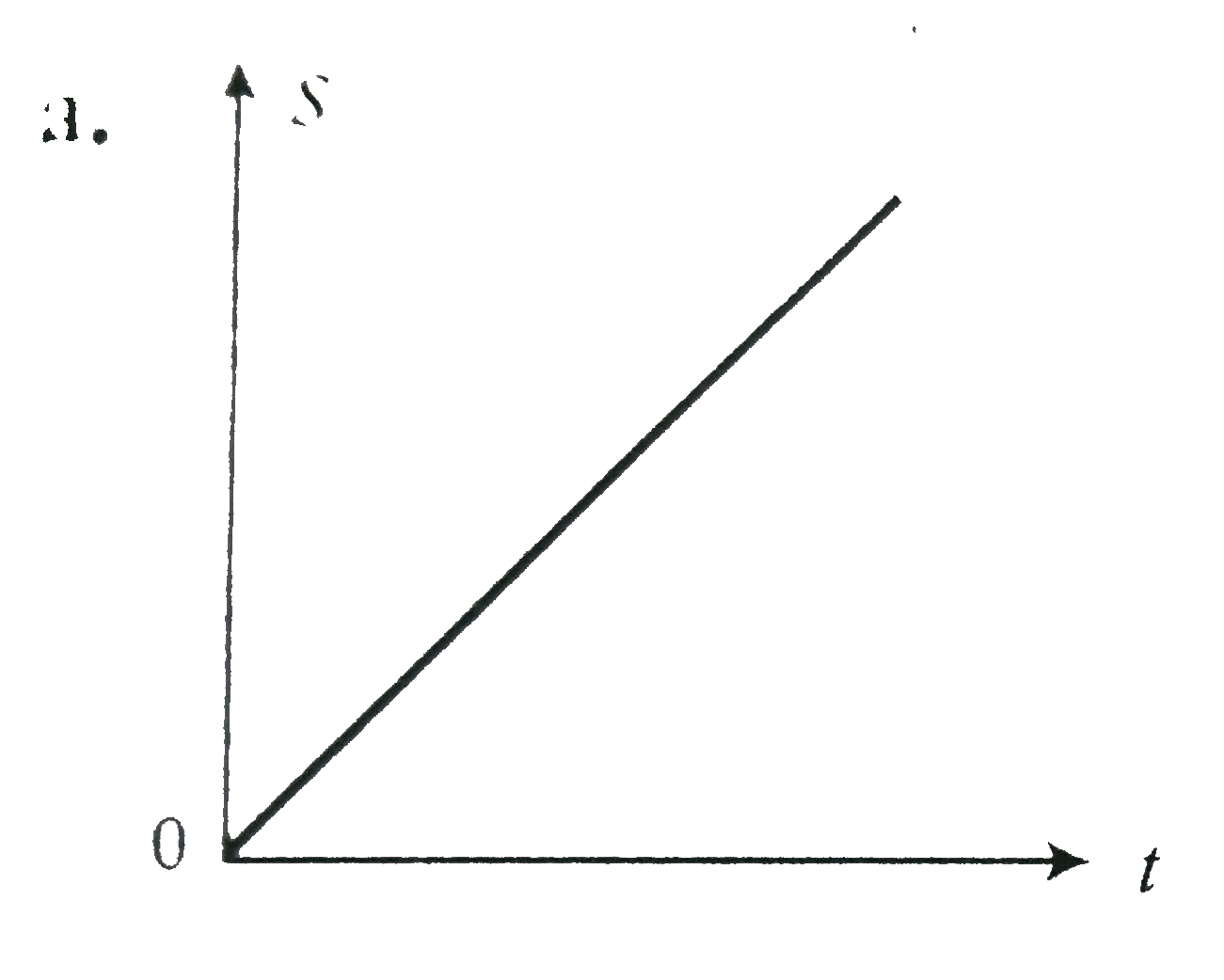 .
.
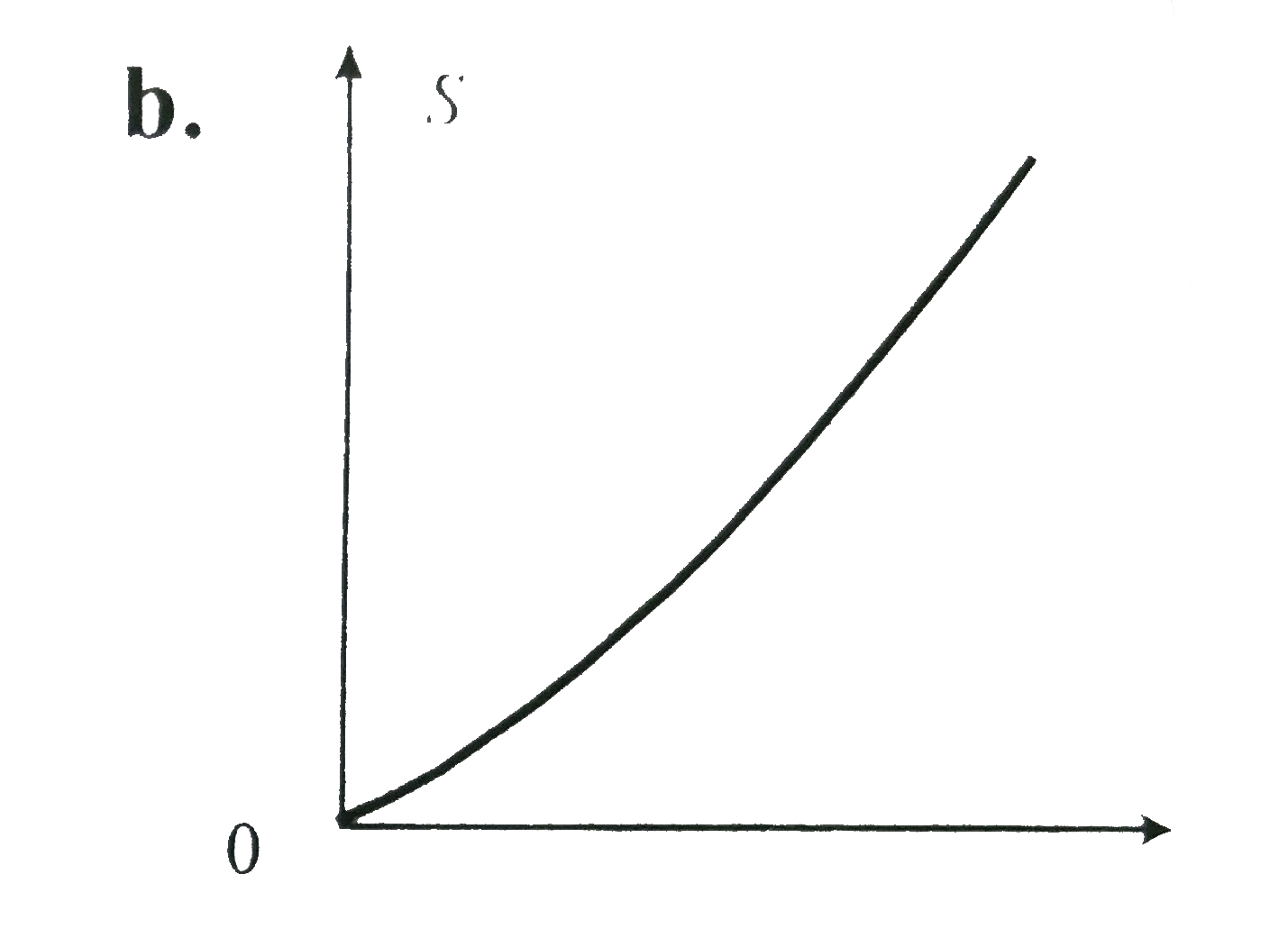 .
.
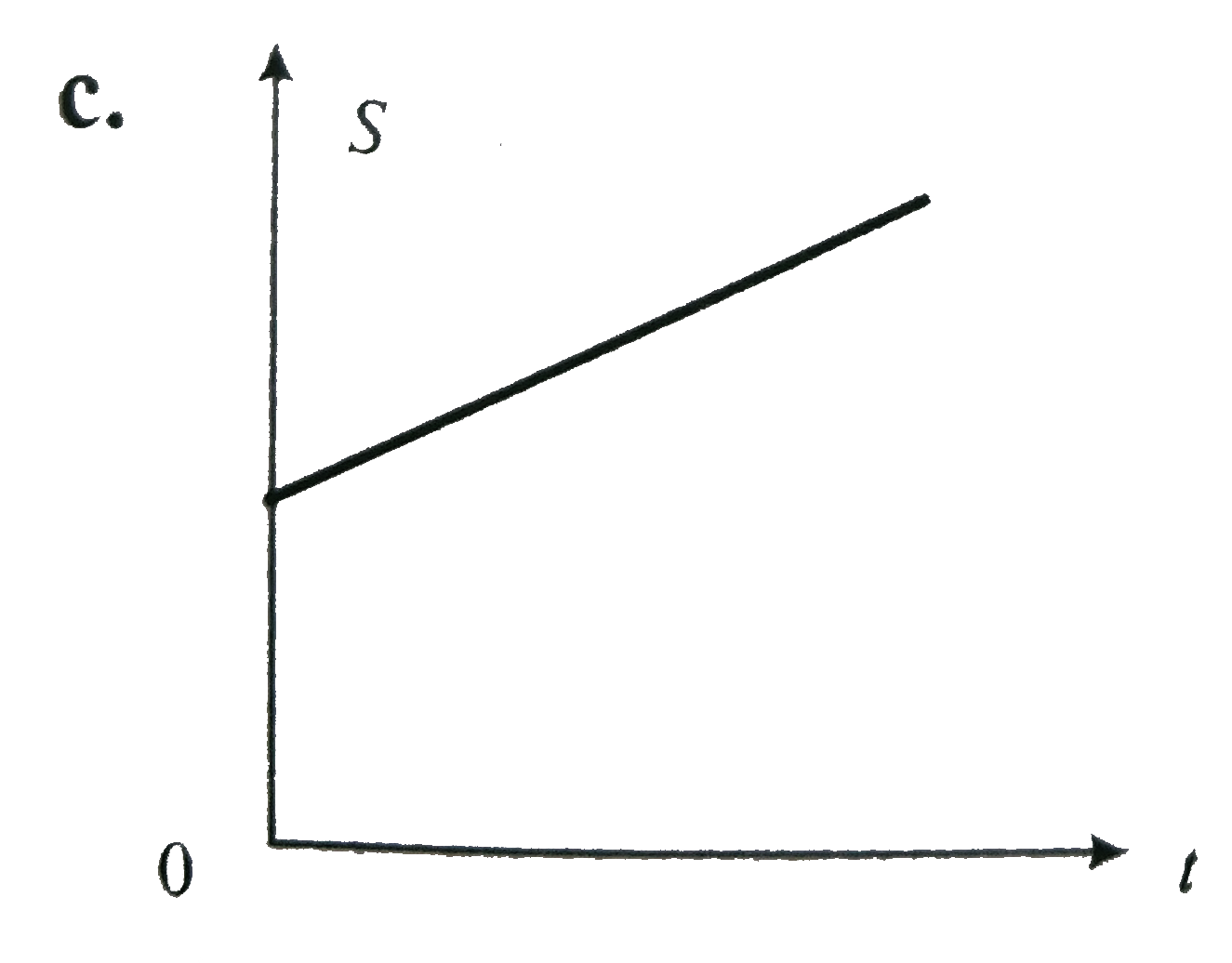 .
.
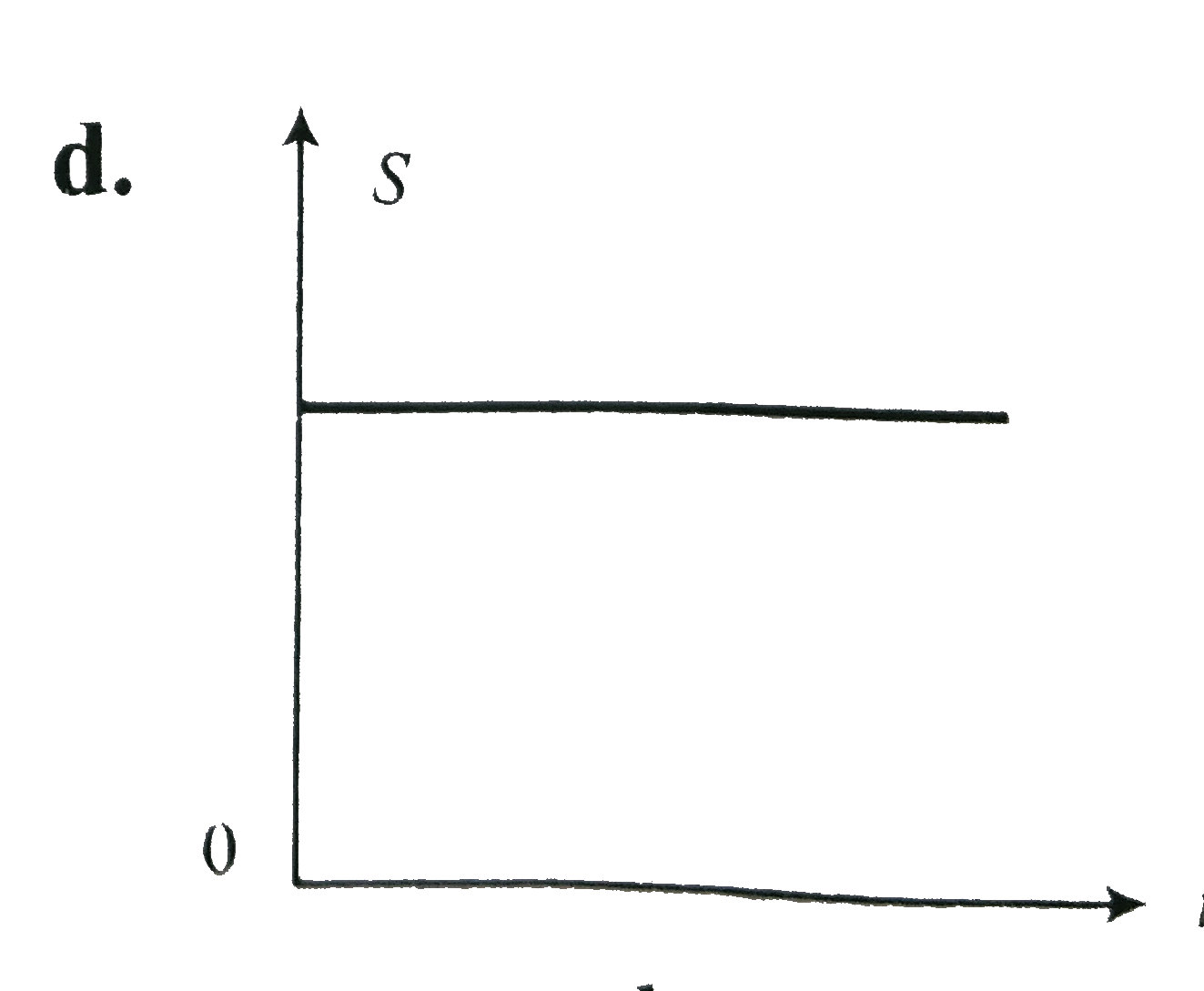 .
.
The correct Answer is:A
To solve the problem, we need to analyze the motion of the two stones and determine the distance S between them as a function of time t.
1. Identify the Initial Conditions:
- Stone 1 is dropped from rest from a height h at time t=0.
- Stone 2 is projected upwards with an initial velocity u at the same time.
2. Determine the Distance Traveled by Each Stone:
- For Stone 1 (dropped from rest):
- The distance S1 it falls after time t can be calculated using the equation of motion:
S1=12gt2
where g is the acceleration due to gravity.
- For Stone 2 (projected upwards):
- The distance S2 it travels upwards after time t can be calculated as:
S2=ut−12gt2
Here, ut is the distance it would travel upwards without gravity, and 12gt2 accounts for the downward acceleration due to gravity.
3. Calculate the Distance Between the Two Stones:
- The distance S between the two stones at any time t is given by:
S=h−S1−S2
- Substituting the expressions for S1 and S2:
S=h−(12gt2)−(ut−12gt2)
- Simplifying this expression:
S=h−ut+gt2
4. Determine the Nature of the Graph:
- The equation S=h−ut+gt2 is a quadratic equation in t since it can be rearranged to:
S=−12gt2+ut+h
- This indicates that the graph of distance S against time t will be a parabola opening downwards (since the coefficient of t2 is negative).
5. Conclusion:
- The graph of the distance S between the two stones plotted against time t will be a downward-opening parabola.
- Stone 1 is dropped from rest from a height h at time t=0.
- Stone 2 is projected upwards with an initial velocity u at the same time.
- For Stone 1 (dropped from rest):
- The distance S1 it falls after time t can be calculated using the equation of motion:
S1=12gt2
where g is the acceleration due to gravity.
- The distance S2 it travels upwards after time t can be calculated as:
S2=ut−12gt2
Here, ut is the distance it would travel upwards without gravity, and 12gt2 accounts for the downward acceleration due to gravity.
- The distance S between the two stones at any time t is given by:
S=h−S1−S2
- Substituting the expressions for S1 and S2:
S=h−(12gt2)−(ut−12gt2)
- Simplifying this expression:
S=h−ut+gt2
- The equation S=h−ut+gt2 is a quadratic equation in t since it can be rearranged to:
S=−12gt2+ut+h
- This indicates that the graph of distance S against time t will be a parabola opening downwards (since the coefficient of t2 is negative).
- The graph of the distance S between the two stones plotted against time t will be a downward-opening parabola.
Topper's Solved these Questions
- KINEMATICS-1Book:CENGAGE PHYSICS ENGLISHChapter:KINEMATICS-1Exercise:Graphical cancept
Explore 1 Video
- KINEMATICS-1Book:CENGAGE PHYSICS ENGLISHChapter:KINEMATICS-1Exercise:Multiple Correct
Explore 16 Videos
- KINEMATICS-1Book:CENGAGE PHYSICS ENGLISHChapter:KINEMATICS-1Exercise:Single Correct
Explore 52 Videos
- GRAVITATIONBook:CENGAGE PHYSICS ENGLISHChapter:GRAVITATIONExercise:INTEGER_TYPE
Explore 1 Video
- KINEMATICS-2Book:CENGAGE PHYSICS ENGLISHChapter:KINEMATICS-2Exercise:Exercise Integer
Explore 9 Videos
Similar Questions
One stone is dropped from a tower from rest and simultaneously another stone is projected vertically upwards from the tower with some initial velocity. The graph of distance (s) between the two stones varies with time (t) as (before either stone hits the ground).
View SolutionA stone is dropped from a height 300 m and at the same time another stone is projected vertically upwards with a velocity of 100m/sec. Find when and where the two stones meet.
View Solution
Knowledge Check
A stone is dropped from the top of a tower and one second later, a second stone is thrown vertically downward with a velocity 20ms−1. The second stone will overtake the first after travelling a distance of (g=10ms−2)
Ag2⎡⎢ ⎢⎣n(u−gn2)(u−gn)⎤⎥ ⎥⎦2Bg2⎡⎢ ⎢⎣n(u2−gn)u−gn⎤⎥ ⎥⎦2Cg2⎡⎢ ⎢⎣n(u2−gn)u2−gn⎤⎥ ⎥⎦2Dg5[u−gnu2−gn]2
A stone is dropped from a height h. simultaneously, another stone is thrown up from the ground which reaches a height 4h. The two stones will cross each other after time:-
View SolutionA stone is dropped from a tower of height 200 m and at the same time another stone is projected vertically up at 50 m/s. The height at which they meet ? [g=10m/s2]
View SolutionA stone is allowed to fall from the top of a tower 300 m height and at the same time another stone is projected vertically up from the ground with a velocity 100ms−1 . Find when and where the two stones meet ?
View SolutionA stone is dropped freely while another thrown vertically downward with an initial velocity of 4 m/s from the same point simultaneously. The distance of separation between them will become 30 m after a time of
View SolutionTwo bodies are thrown simultaneously from a tower with same initial velocity v0: one vertically upwards, the other vertically downwards. The distance between the two bodies after time t is
View SolutionFrom a 10 m high building a ston 'A' is dropped, and simultaneously another identical stone 'B' is thrown horizontally with an initial speed of 5ms−1. Which one of the following statement is true?
View SolutionA stone is allowed to fall from the top of a tower 100m high and at the same time another stone is projected vertically upwards from the ground with a velocity of 25m/s. Calculate when and where the two stones will meet.
View Solution
