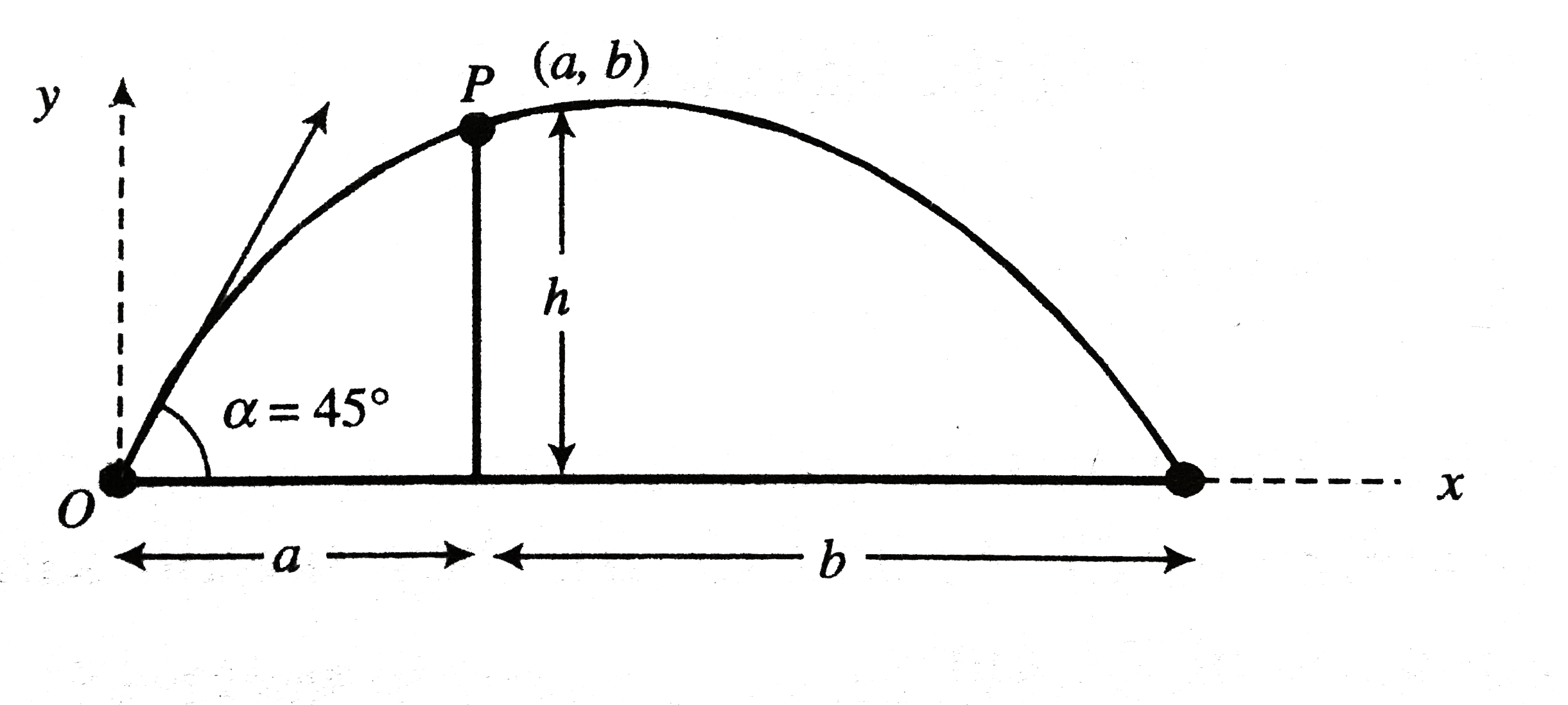
From a point on the ground at a distance a from the foot of a pole, a ball is thrown at an angle of 45∘, which just touches the top of the pole and strikes the ground at a distance of b, on the outer side of it. Find the height of the pole.
Let h be the height of the pole.
We have y=xtan∝(1−xR)
Since the top of pole lies on curve (1),
h=atan45∘(1−1a+b)=a[a+b−aa+b]=aba+b.
 .
.
Topper's Solved these Questions
- KINEMATICS-2BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-2EXERCISE - Solved Examples
7 Videos
- KINEMATICS-2BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-2EXERCISE - Exercise 5.1
15 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-1EXERCISE - Integer
9 Videos
- KINETIC THEORY OF GASESBOOK - CENGAGE PHYSICSCHAPTER - KINETIC THEORY OF GASESEXERCISE - Compression
2 Videos
Similar Questions
From a pint on the ground at a distance 15m from the foot of a vertical wall, a ball is thrown at an angle of 45∘ which just clears the top of the wall and afterwards strikes the ground at a distance 5m on the other side. Find the height of the wall.
View SolutionA ball projected from ground at an angle of 45∘ just clears a wall infront. If point of projection is 4m from the foot of wall and ball strikes the ground at a distance of 6m on the other side of the wall, the height of the wall is
View Solution
Knowledge Check
From a point on the ground at a distance of 2m from the fot of a verticle wal, a ball is thrown at an angle of 45∘ which just clears the top of the wall and then strikes the ground at distance of 4m from the foot of the wall on the other side. The height of the wall is
A3mB2/3mC3/4mD4/3mA body is projected at an angle of 45∘ with horizontal from a point on ground at distance 6m from the foot of a vertical pole. It just crosses the top of the pole and falls on ground on the other side at a distance of 3m from the foot of the pole. The height of the pole is:
A1mB2mC3mD4mA ball projected from ground at an angle of 45∘ just clears a wall infront. If point of projection is 4m from the foot of wall and ball strikes the ground at a distance of 6m on the other side of the wall, the height of the wall is
A4.4mB2.4mC3.6mD1.6m
From a point on the ground which is at a distance of 50 m from the foot of the towe, the angle of elevation of the top of the tower is observed to be 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the bottom of a window 10 m above the ground level from a point on the ground is 30∘ A pole projecting outwards from the bottom of the window makes an angle of 30∘ with the wall. If the angle of elevation of the top of the pole observed from the same point on the ground is 60∘, find the length of the pole to the nearest whole number.
View SolutionA ball is projectedfrom ground at an angle 45∘ with horizontal from distance d1 from the foot of a pole and just after touching the top of pole it the falls on ground at distance d2 from pole on other side, the height of pole is
View SolutionA particle just clear a wall of height b at a distance a and strikes the ground at a distance from the point of projection, the angle of projection is :
View SolutionA ball is projected from at an angle 45∘ with horizontal from distance d1 from the foot of a pole and just after touching the top of pole it the falls on ground at distance d2 from pole on other side, the height of pole is
View Solution
