From the top of tower of height 80m, two stones are projected horizontally with velocities 20ms−1 and 30ms−1 in opposite directions, Find the distance between both the stones on reaching the ground (in102m).
The correct Answer is:2
80=12×10
t2=805⇒t=4
x1=20×4=80
x2=30×4=120
Separation x1+x2=80+120=200m.
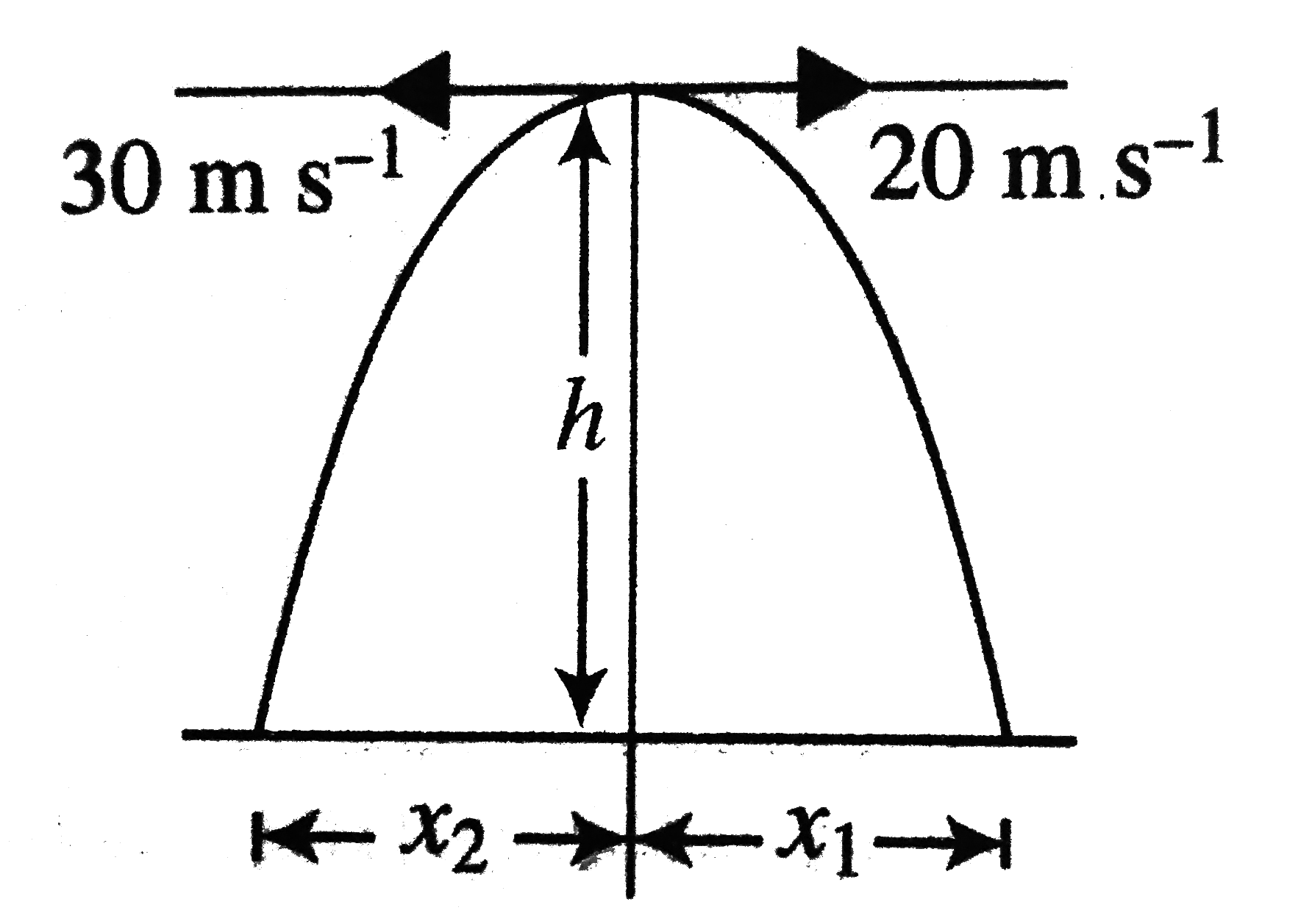 .
.
Topper's Solved these Questions
Similar Questions
A body projected horizontally with a velocity V from the top of a tower of height h touches the level ground at a distance X from the foot of the tower. Another body is projected horizontally with velocity 2V simultaneously from the same point exactly in the opposite direction. On reaching the ground, separation between the two bodies is?
View SolutionTwo stones AandB are projected simultaneously from the top of a 100−m high tower. Stone B is projected horizontally with speed 10ms−1, and stone A is droppd from the tower. Find out the following :
(a) Time of flight of the two stone
(b) Distance between two stones after 3s
(c ) Angle of strike with ground
(d) Horizontal range of particle B.View Solution
Knowledge Check
- Question 1 - Select One
From the top of a tower of height 78.4m two stones are projected horizontally with 10m/s and 20m/s in opposite directions. On reaching the ground, their separation is
A120mB100mC200mD150m - Question 2 - Select One
From a tower of height 40m, two bodies are simultaneously projected horizontally in opposite direction, with velocities 2ms−1and8ms−1. respectively.
The horizontal distance between two bodies, when their velocity are perpendicular to each other, is.A1 mB0.5 mC2 mD4 m - Question 3 - Select One
A stone is project vertically upward from the top of a tower of height 60 m. The stone moves according to the law S=(10)t−5t2, where S is in meters and t in second The maximum height , reached by the stone , from the ground , is
A60 mB55 mC65 mD50 m
From top of a tower of hight 80m, person drops a stone. And after one second another stone is thrown down from the top of the same tower with some initial velocity, such that both the stones reach the ground at the same instant. Calculate the magnitude of the initial velociy (in m/s) of the second stone. (Take g=10m/s2)
View SolutionA stone is projected at 30∘ above to the horizontal with a speed 20ms−1 from the top of a tower of height 120m .The time after which it reaches the ground is (g=10ms−2)
View SolutionFrom the top of a tower of height 100 m, a ball is projected with a velocity of 10m/sec. It takes 5 seconds to reach the ground. If g=10m/sec2 then the angle of projection is
View SolutionFrom the top of a tower, 80m high from the ground a stone is thrown in the horizontal direction with a velocity of 8ms1. The stone reaches the ground after a time t and falls at a distance of d from the foot of the tower. Assuming g=10ms2, the time t and distance d are given respectively by
View SolutionA ball is thrown horizontally with a speed of 20m/s from the top of a tower of height 100m
Horizontal distance travelled by the before it strickes the ground isView Solution

