Helium gas goes through a cycle ABCDA (consisting of two isochoric and two isobaric lines) as shown in figure. Eficiency of this cycle is nearly:
(Assume the gas to be close to ideal gas)
12.5%
15.4%
9.1%
10.5%
The correct Answer is:B
η%P0V0f2(P0V0)+f2(2P0)V0+2P0V0=132+3+2
=20013=15.4%
Correct choice : (b).
Topper's Solved these Questions
- HEAT AND THERMODYNAMICSBOOK - MODERN PUBLICATIONCHAPTER - HEAT AND THERMODYNAMICSEXERCISE - Recent Competitve Questions
17 Videos
- HEAT AND THERMODYNAMICSBOOK - MODERN PUBLICATIONCHAPTER - HEAT AND THERMODYNAMICSEXERCISE - Multiple Choice Questions (LEVEL-II)
55 Videos
- GRAVITATIONBOOK - MODERN PUBLICATIONCHAPTER - GRAVITATIONEXERCISE - Recent Competitive Questions
8 Videos
- LAWS OF MOTIONBOOK - MODERN PUBLICATIONCHAPTER - LAWS OF MOTIONEXERCISE - Revision test
43 Videos
Similar Questions
Helium gas goes through a cycle ABCDA (consisting of two isochoric and isobaric lines) as shown in figure Efficiency of this cycle is nearly: (Assume the gas to be close to ideal gas)

View SolutionHelium gas goes through a cycle ABCDA (consisting of two isochoric and isobaric lines) as shown in figure Efficiency of this cycle is nearly: (Assume the gas to be close to ideal gas)

View Solution
Knowledge Check
Helium gas goes through a cycle ABCDA (consisting of two isochoric and isobaric lines) as shown in figure Efficiency of this cycle is nearly: (Assume the gas to be close to ideal gas)
 A15.4%B9.1%C10.5%D12.5%
A15.4%B9.1%C10.5%D12.5%Helium gas goes through a cycle ABCDA (consisting of two isochoric and isobaric lines) as shown in figure Efficiency of this cycle is nearly: (Assume the gas to be close to ideal gas)
 A15.4%B9.1%C10.5%D12.5%
A15.4%B9.1%C10.5%D12.5%An ideal monoatomic gas is carried around the cycle ABCDA as shown in the figure. The efficiency of the gas cycle is
 A421B221C431D231
A421B221C431D231
An ideal gas whose adiabatic exponent equals γ goes through a cycle consisting of two isochoric and two isobaric lines. Find the efficiency of such a cycle, if the absolute temperature of the gas rises n times both in a isochoric heating and in the isobaric expansion.
View SolutionAn ideal gas with the adiabatic exponent γ goes through a direct (clockwise) cycle conisting of adiabatic, isobaric, and isochoric lines. Find the efficiency of the cycle if in the adiabatic process the volume of the ideal gas.
(a) increases n-fold ,
(b) decreases n-fold.
View SolutionAn ideal gas is carried through a thermodynamic cycle consisting of two isobaric and two isothermal processes as shown in figure. Show that the net work done in the entire cycle is given by the equation. Wnet=p1(V2−V1)Inp2p1
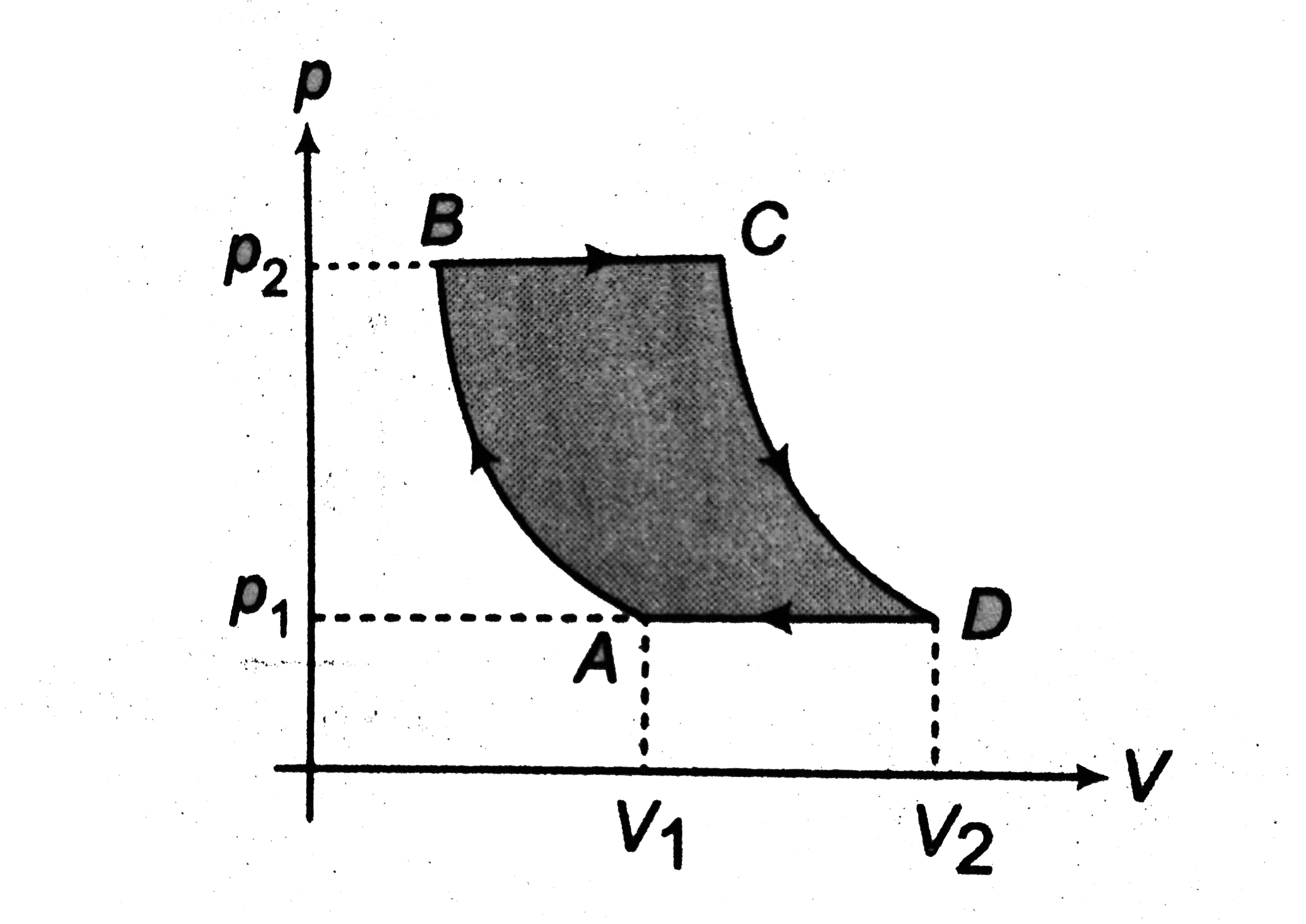
View SolutionAn ideal gas is carried through a thermodynamic cycle consisting of two isobaric and two isothermal processes as shown in figure. Show that the net work done in the entire cycle is given by the equation. Wnet=p1(V2−V1)Inp2p1

View SolutionAn ideal monoatomic gas is carried around the cycle ABCDA as shown in the figure. The efficiency of the gas cycle is

View Solution
