An ideal gas with the adiabatic exponent γ goes through a direct (clockwise) cycle conisting of adiabatic, isobaric, and isochoric lines. Find the efficiency of the cycle if in the adiabatic process the volume of the ideal gas.
(a) increases n-fold ,
(b) decreases n-fold.
To find the efficiency of a cycle consisting of adiabatic, isobaric, and isochoric processes for an ideal gas, we need to analyze both scenarios: when the volume increases n-fold during the adiabatic process and when it decreases n-fold.
Step 1: Understand the Cycle
- The cycle consists of three processes:
1. Adiabatic Process (1 to 2): No heat exchange (Q = 0).
2. Isobaric Process (2 to 3): Constant pressure.
3. Isochoric Process (3 to 1): Constant volume.
Step 2: Analyze the Adiabatic Process
- For an ideal gas undergoing an adiabatic process, the relation between pressure (P), volume (V), and temperature (T) is given by:
PVγ=constant
- If the volume increases n-fold, we have:
V2=nV1
- The temperatures can be related as:
T2T1=(V1V2)γ−1=(1n)γ−1
- If the volume decreases n-fold:
V2=V1n
- The relation becomes:
T2T1=nγ−1
Step 3: Calculate Heat Transfer for Each Process
- Adiabatic Process (1 to 2):
Q1→2=0
- Isobaric Process (2 to 3):
Q2→3=nCp(T3−T2)
- Isochoric Process (3 to 1):
Q3→1=nCv(T1−T3)
Step 4: Total Heat Transfer in the Cycle
- The total heat added to the system is:
Qtotal=Q2→3+Q3→1=nCp(T3−T2)+nCv(T1−T3)
Step 5: Calculate Work Done in the Cycle
- The work done in the cycle is given by:
W=Qtotal−ΔU
- For a cyclic process, the change in internal energy (ΔU) is zero, thus:
W=Qtotal
Step 6: Calculate Efficiency
- The efficiency (η) of the cycle is given by:
η=WQtotal
- Since W=Qtotal, the efficiency becomes:
η=Q2→3Qtotal
Step 7: Substitute Values for Efficiency
- Substitute the expressions for Q2→3 and Q3→1 into the efficiency formula to find the final result.
- The cycle consists of three processes:
1. Adiabatic Process (1 to 2): No heat exchange (Q = 0).
2. Isobaric Process (2 to 3): Constant pressure.
3. Isochoric Process (3 to 1): Constant volume.
- For an ideal gas undergoing an adiabatic process, the relation between pressure (P), volume (V), and temperature (T) is given by:
PVγ=constant
- If the volume increases n-fold, we have:
V2=nV1
- The temperatures can be related as:
T2T1=(V1V2)γ−1=(1n)γ−1
- If the volume decreases n-fold:
V2=V1n
- The relation becomes:
T2T1=nγ−1
- Adiabatic Process (1 to 2):
Q1→2=0
- Isobaric Process (2 to 3):
Q2→3=nCp(T3−T2)
- Isochoric Process (3 to 1):
Q3→1=nCv(T1−T3)
- The total heat added to the system is:
Qtotal=Q2→3+Q3→1=nCp(T3−T2)+nCv(T1−T3)
- The work done in the cycle is given by:
W=Qtotal−ΔU
- For a cyclic process, the change in internal energy (ΔU) is zero, thus:
W=Qtotal
- The efficiency (η) of the cycle is given by:
η=WQtotal
- Since W=Qtotal, the efficiency becomes:
η=Q2→3Qtotal
- Substitute the expressions for Q2→3 and Q3→1 into the efficiency formula to find the final result.
Topper's Solved these Questions
- THERMODYNAMICS AND MOLECULAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - Liquids Capillary Effects
25 Videos
- THERMODYNAMICS AND MOLECULAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - Phase Transformations
35 Videos
- THERMODYNAMICS AND MOLECULAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - Kinetic Theory Of Gases
51 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Relativistic Mechanics
49 Videos
Similar Questions
Calculate the efficiency of a cycle consisting of isothermal, isobaric, and isochoric line, if in the isothermal process the volume of the ideal gas with the adiabatic exponent γ.
(a) increased n−fold ,
(b) decreases n−fold.
View SolutionAn ideal gas with the adiabatic exponent γ goes through a cycle (Fig. 2.3) within which the absolute temperature varies τ−fold. Find the efficiency of this cycle.
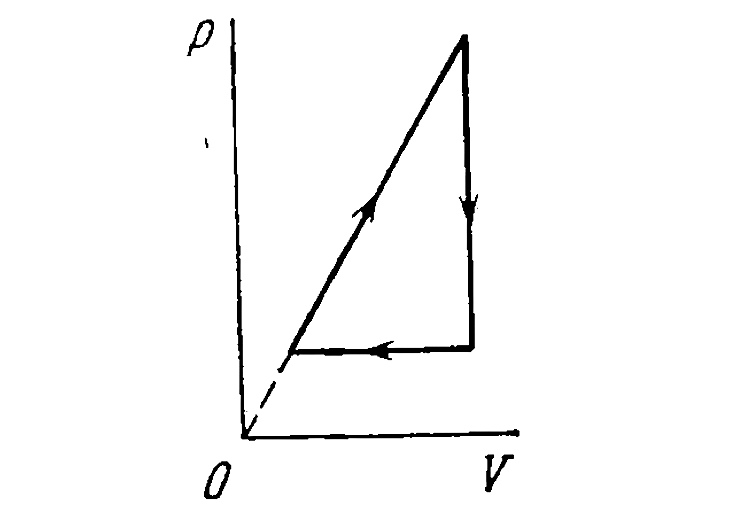 .
.
View Solution
Knowledge Check
Helium gas goes through a cycle ABCDA (consisting of two isochoric and isobaric lines) as shown in figure Efficiency of this cycle is nearly: (Assume the gas to be close to ideal gas)
 A15.4%B9.1%C10.5%D12.5%
A15.4%B9.1%C10.5%D12.5%Helium gas goes through a cycle ABCDA (consisting of two isochoric and isobaric lines) as shown in figure Efficiency of this cycle is nearly: (Assume the gas to be close to ideal gas)
 A15.4%B9.1%C10.5%D12.5%
A15.4%B9.1%C10.5%D12.5%An ideal gas with adiabatic exponent γ is heated at constant pressure. It absorbs Q amount of heat. Fraction of heat absorbed in increasing the temperature is
AγB1γC1−1γD2γ
An ideal gas whose adiabatic exponent equals γ goes through a cycle consisting of two isochoric and two isobaric lines. Find the efficiency of such a cycle, if the absolute temperature of the gas rises n times both in a isochoric heating and in the isobaric expansion.
View SolutionHydrogen is used in a Carnot cycle as a working substance. Find the efficiency of the cycle, if as a result of an adiabatic expansion
(a) the gas volume increases n=2.0 times ,
(b) the pressure decreases n=2.0 times.
View SolutionAn ideal gas with the adiabatic exponent γ goes through a process p=p0−αV, where p0 and α are positive constants, and V is the volume. At what volume will the gas entropy have the maximum value ?
View SolutionAn ideal gas with adiabatic exponent gamma is heated isochorically.If it absorbs Q amount heat then, fraction of heat absorbed in increasing the internal energy is
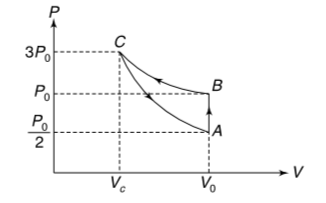
View SolutionOne mole of an ideal gas is carried through a thermodynamics cycle as shown in the figure. The cycle consists of an isochoric, an isothermal and an adiabatic process. Find the adiabatic exponent of the gas.
View Solution
