How do you find experimentally the refractive index of material of a prism ?
Aim: To find the refractive index of a prism.
Material required : Prism, piece of white chart of size 20×20 cm, pencil, pins, scale and protector.
Procedure :
1) Take a prism and place it on the white chart in such a way that the triangular base of the prism is on the chart.
2) Draw a line around the prism base using pencil. Remove the prism and name the vertices of the triangle as formed as P, Q and R.
3) Measure the angle between PQ and PR. This is the angle of the prism (A).
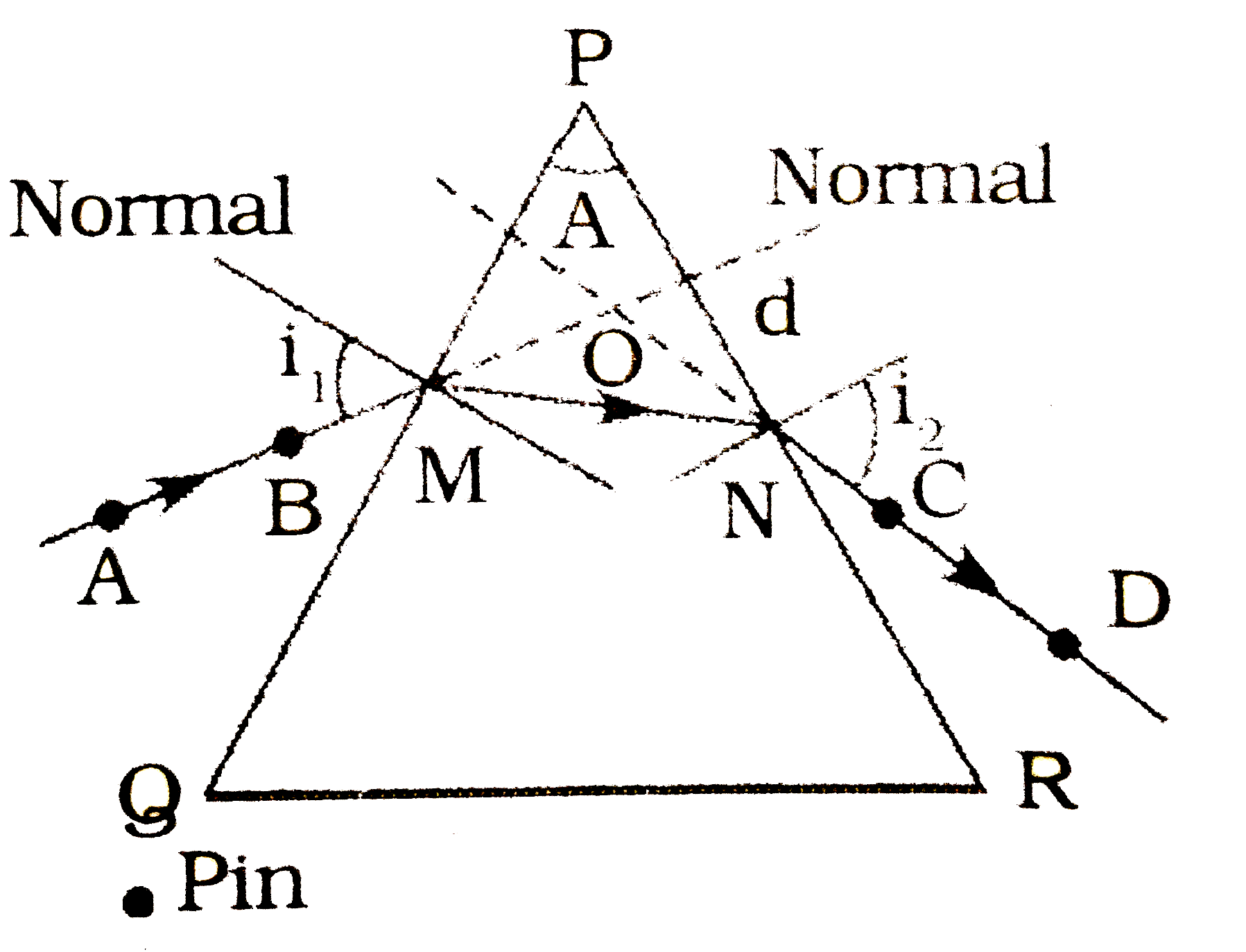
4) Mark M on the side of ΔPQR and also draw a perpendicular to PQ at M.
5) Draw a line with 30∘ to the normal at M. This line denotes the incident ray. Note the value in the table.
6) Place the prism in its position (Δ) again.
7) Now fix two pins vertically on the line at the points A and B.
8) Look for the angles of pins through the prism from the other side (PR) and fix another two pins at points C and D in such a way that all the four pins appear to lie along the same straight line.
9) Now remove the prism and take out pins.
10) Draw a line joining C and D and extend it to meet PR at N. This is the emerging ray.
11) Draw a normal to PR at N .
12) The angle between the normal at N and emergent ray is the angle of emergence . Measure this angle and note its value in the above table.
13) Now join the points M and N by a straight line. The line passing through A,B,M,N,C and D represents the path of light when it suffers refraction through the prism.
14) Extend both incident and emergent rays till they meet at point 'O'.
15) Measure ∠MON. This is the angle of deviation, denoted by 'd'. Note this value in the table.
16) Repeat the process for different angles of incidence and measure corresponding angles of deviations.
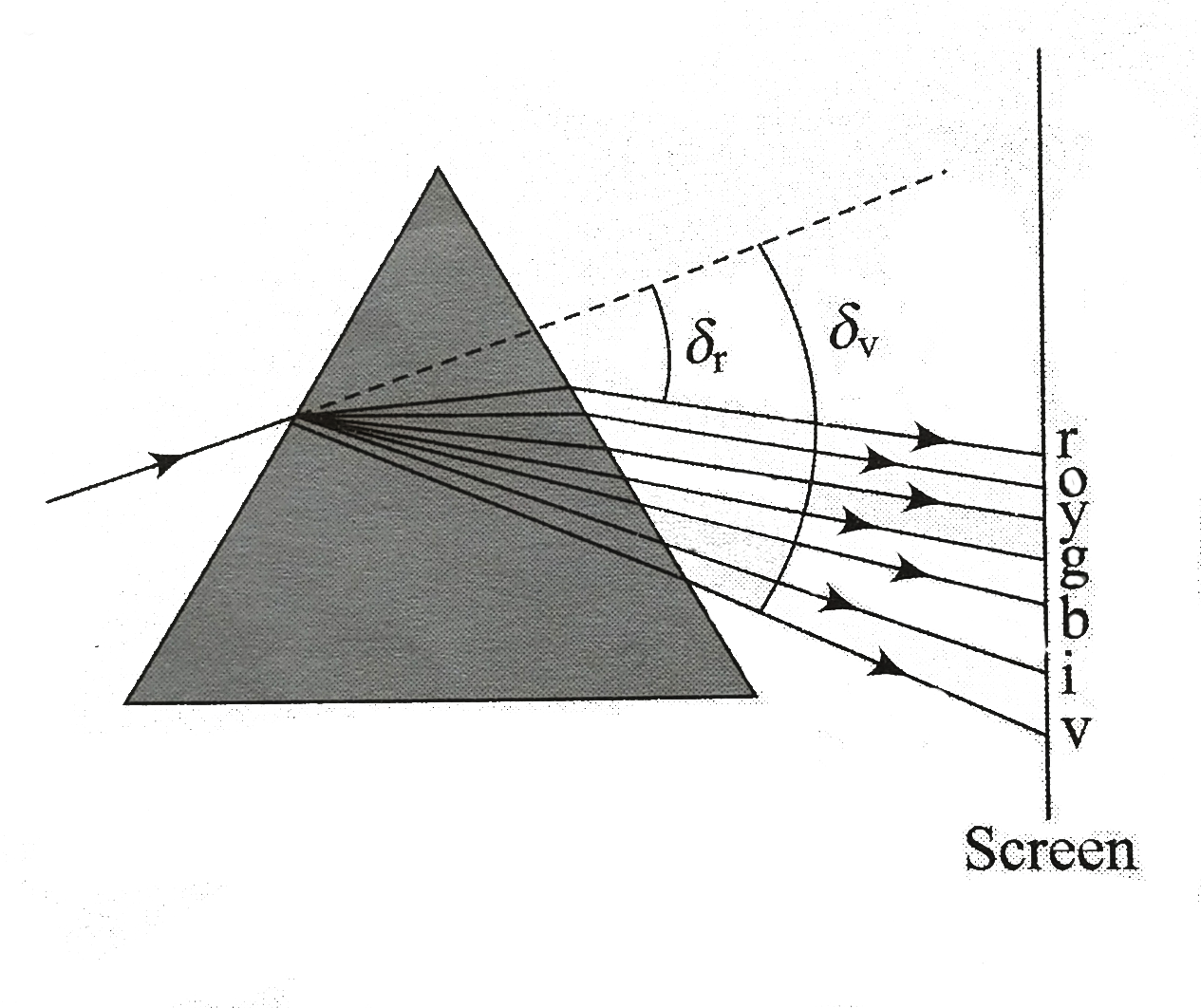
17) Take angle of incidence along X-axis and the angle of deviation along y-axis . Draw a graph.
18) We obtain a curve.
19) Draw a tangent line to the curve, parallel to X-axis , at the lowest point of the graph. The point where this line cuts Y-axis gives the angle of minimum deviation (D).
(20) The refractive index of prism n= sin(A+D2)sin(A2)
(21) Using this formula , we can measure the refractive index of the material of the prism.

Topper's Solved these Questions
- HUMAN EYE AND COLOURFUL WORLDBOOK - VGS PUBLICATION-BRILLIANTCHAPTER - HUMAN EYE AND COLOURFUL WORLDEXERCISE - TEXTUAL LESSON PART IMPROVE YOUR LEARNING (ASKING QUESTIONS AND MAKING HYPOTHESIS)
1 Videos
- HUMAN EYE AND COLOURFUL WORLDBOOK - VGS PUBLICATION-BRILLIANTCHAPTER - HUMAN EYE AND COLOURFUL WORLDEXERCISE - TEXTUAL LESSON PART IMPROVE YOUR LEARNING (EXPERIMENTATION AND FIELD INVESTIGATION)
3 Videos
- HUMAN EYE AND COLOURFUL WORLDBOOK - VGS PUBLICATION-BRILLIANTCHAPTER - HUMAN EYE AND COLOURFUL WORLDEXERCISE - CREATIVE QUESTIONS FOR NEW MODEL EXAMINATION (SECTION-IV) (APPLICATION TO DAILY LIFE, CONCERN TO BIODIVERSITY)
1 Videos
- GRAVITATIONBOOK - VGS PUBLICATION-BRILLIANTCHAPTER - GRAVITATIONEXERCISE - ADDITIONAL PROBLEMS
4 Videos
- KINETIC THEORYBOOK - VGS PUBLICATION-BRILLIANTCHAPTER - KINETIC THEORYEXERCISE - Additional Problems
5 Videos
Similar Questions
If the angle of minimum deviation is of 60∘ for an equilateral prism , then the refractive index of the material of the prism is
View SolutionThe refracting angle of the prism is 60∘ and the refractive index of the material of the prism is √3. Calculate the angle of minimum deviation in degree.
View Solution
Knowledge Check
If the angle of minimum deviation is of 60∘ for an equilateral prism , then the refractive index of the material of the prism is
A1.41B1.5C1.6D1.73The refracting angle of a prism is A, the refractive index of the material of the prism is cot(A2). The angle of minimum deviation is :
A180∘−2AB90∘−AC180∘+2AD180∘−3AThe angle of minimum deviation of a prism is (π−2A) . Where A represents angle of the prism. The refractive index of material of prism is
AsinA2BcosA2CtanA2DcotA2
The refracting angle of the prism is 60∘ and the refractive index of the material of the prism is √3. Calculate the angle of minimum deviation in degree.
View Solution(a) If the refracting angle of a prism is 60∘ and the minimum deviation is 30∘, then find the angle of incidence.
(b) A ray of light passes through an equilateral prism such that the angle of emergence is equal to the angle of incidence and each is equal to 3/4th of the angle of prism. Find the angle of deviation.
(c) The refracting of a prism is A and the refractive index of the material of the prism is cotA/2. Find the angle of deviation.
(d) The angle of a prism is 60∘. What is the angle of incidence for minimum deviation? The refractive index of the material of the prism is √2.
View SolutionThe angle of minimum deviation from a prism is 37∘. If the angle of prism is 53∘, find the refractive index of the material of the prism.
View SolutionA thin prism of angle A=6∘ produces a deviation d=3∘. Find the refractive index of the material of prism.
View SolutionThe refractive angle of a prism is 60∘ . What is the angle of minimum deviation, if the refractive index of the material of the prism is √2 ?
View Solution
