The angle of minimum deviation from a prism is 37∘. If the angle of prism is 53∘, find the refractive index of the material of the prism.
To find the refractive index of the material of the prism given the angle of minimum deviation and the angle of the prism, we can use the formula for the refractive index (n) of a prism:
n=sin(A+Dmin2)sin(A2)
Where:
- A is the angle of the prism,
- Dmin is the angle of minimum deviation.
1. Identify the given values:
- Angle of prism A=53∘
- Angle of minimum deviation Dmin=37∘
2. Calculate A+Dmin2:
A+Dmin2=53∘+37∘2=90∘2=45∘
3. Calculate A2:
A2=53∘2=26.5∘
4. Find the sine values:
- sin(45∘)=√22≈0.707
- sin(26.5∘) can be calculated using a calculator or sine table. It is approximately 0.447.
5. Substitute the values into the refractive index formula:
n=sin(45∘)sin(26.5∘)=0.7070.447
6. Calculate the refractive index:
n≈1.58
7. Round off the value:
The refractive index of the material of the prism is approximately 1.6.
Final Answer:
The refractive index of the material of the prism is n≈1.6.
- A is the angle of the prism,
- Dmin is the angle of minimum deviation.
- Angle of prism A=53∘
- Angle of minimum deviation Dmin=37∘
A+Dmin2=53∘+37∘2=90∘2=45∘
A2=53∘2=26.5∘
- sin(45∘)=√22≈0.707
- sin(26.5∘) can be calculated using a calculator or sine table. It is approximately 0.447.
n=sin(45∘)sin(26.5∘)=0.7070.447
n≈1.58
The refractive index of the material of the prism is approximately 1.6.
The refractive index of the material of the prism is n≈1.6.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBook:HC VERMA ENGLISHChapter:GEOMETRICAL OPTICSExercise:Question For short Answer
Explore 18 Videos
- GEOMETRICAL OPTICSBook:HC VERMA ENGLISHChapter:GEOMETRICAL OPTICSExercise:Exercises
Explore 78 Videos
- GAUSS LAWBook:HC VERMA ENGLISHChapter:GAUSS LAWExercise:Short Question
Explore 7 Videos
- LIGHT WAVESBook:HC VERMA ENGLISHChapter:LIGHT WAVESExercise:Question for short Answer
Explore 11 Videos
Similar Questions
The angle of minimum deviation measured with a prism is 30∘ and the angle of prism is 60∘. The refractive index of prism material is
View SolutionIf the angle of minimum deviation is of 60∘ for an equilateral prism , then the refractive index of the material of the prism is
View SolutionThe angle of incidence for a ray of light at a refracting surface of a prism is 45∘. The angle of prism is 60∘. If the ray suffers minimum deviation through the prism, the angle of minimum deviation and refractive index of the material of the prism respectively, are :
View SolutionA ray of light falling at an angle of 50∘ is refracted through a prism and suffers minimum deviation. The angle of the prism is 60∘. Find the angle of minimum deviation and refraction index of the material of the prism.
View SolutionThe angle of minimum deviation produced by a 60∘ prism is 40∘. Calculate the refractive index of the material of the prism.
View SolutionA thin prism of angle A=6∘ produces a deviation d=3∘. Find the refractive index of the material of prism.
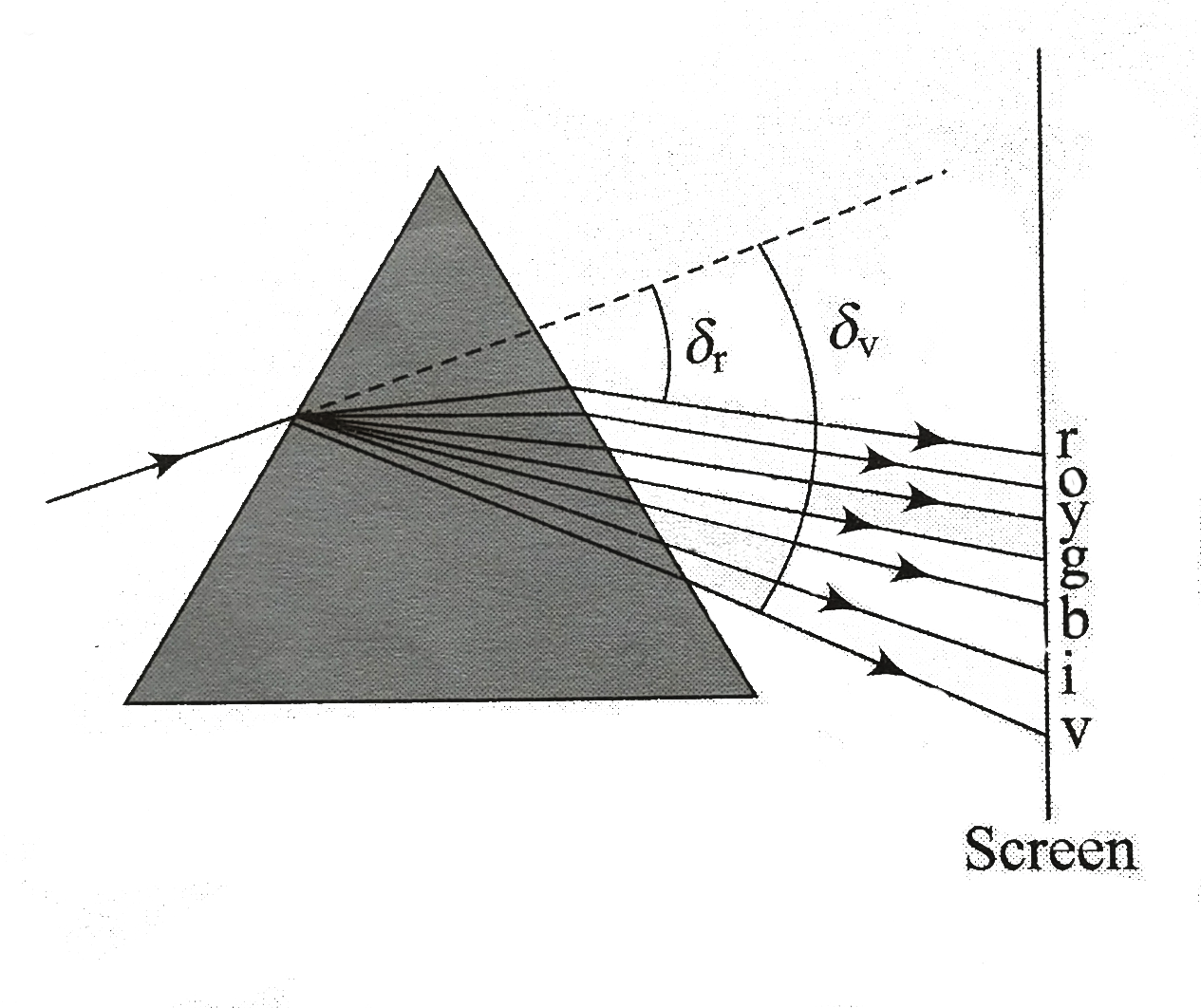
View SolutionFor a ray of light refracted through a prism of angle 60∘, the angle of incidence is equal to the angle of emergence, each equal to 45∘. Find the refractive index of the material of the prism.
View SolutionThe angle of minimum deviation for a glass prism with μ=√3 equals the angle of the prism. What is the angle of the prism?
View SolutionWhen light of wavelength λ is incident on an equilateral prism, kept on its minimum deviation position, it is found that the angle of deviation equals the angle of the prism itself. The refractive index of the material of the prism for the wavelength λ is
View SolutionThe angle of minimum deviation produced by an equilateral prism is 46∘ The refractive index of material of the prism.
View Solution
