How much heat must be absorbed by ice of mass m= 720g at −10∘C to take it to the liquid state at 15∘C?
To solve the problem of how much heat must be absorbed by ice of mass m=720g at −10∘C to take it to the liquid state at 15∘C, we will break the process into three steps:
Step 1: Heating the Ice from −10∘C to 0∘C
First, we need to heat the ice from −10∘C to 0∘C. The formula for heat absorbed or released is given by:
Q1=m⋅Cice⋅ΔT
Where:
- m=720g=0.720kg (mass of ice)
- Cice=2.09kJ/kg⋅K (specific heat capacity of ice)
- ΔT=0−(−10)=10K
Calculating Q1:
Q1=0.720kg⋅2.09kJ/kg⋅K⋅10K=15.048kJ
Step 2: Melting the Ice at 0∘C
Next, we need to melt the ice at 0∘C to convert it into water. The heat required for this phase change is given by:
Q2=m⋅Lf
Where:
- Lf=334kJ/kg (latent heat of fusion for ice)
Calculating Q2:
Q2=0.720kg⋅334kJ/kg=240.48kJ
Step 3: Heating the Water from 0∘C to 15∘C
Finally, we need to heat the water from 0∘C to 15∘C. The formula for heat absorbed is:
Q3=m⋅Cwater⋅ΔT
Where:
- Cwater=4.186kJ/kg⋅K (specific heat capacity of water)
- ΔT=15−0=15K
Calculating Q3:
Q3=0.720kg⋅4.186kJ/kg⋅K⋅15K=45.186kJ
Total Heat Absorbed
Now, we can find the total heat absorbed by adding all three quantities:
Qtotal=Q1+Q2+Q3
Calculating Qtotal:
Qtotal=15.048kJ+240.48kJ+45.186kJ=300.714kJ
Final Answer
The total heat that must be absorbed by the ice to convert it to water at 15∘C is approximately 300.71kJ.
---
First, we need to heat the ice from −10∘C to 0∘C. The formula for heat absorbed or released is given by:
Q1=m⋅Cice⋅ΔT
Where:
- m=720g=0.720kg (mass of ice)
- Cice=2.09kJ/kg⋅K (specific heat capacity of ice)
- ΔT=0−(−10)=10K
Q1=0.720kg⋅2.09kJ/kg⋅K⋅10K=15.048kJ
Next, we need to melt the ice at 0∘C to convert it into water. The heat required for this phase change is given by:
Q2=m⋅Lf
Where:
- Lf=334kJ/kg (latent heat of fusion for ice)
Q2=0.720kg⋅334kJ/kg=240.48kJ
Finally, we need to heat the water from 0∘C to 15∘C. The formula for heat absorbed is:
Q3=m⋅Cwater⋅ΔT
Where:
- Cwater=4.186kJ/kg⋅K (specific heat capacity of water)
- ΔT=15−0=15K
Q3=0.720kg⋅4.186kJ/kg⋅K⋅15K=45.186kJ
Now, we can find the total heat absorbed by adding all three quantities:
Qtotal=Q1+Q2+Q3
Calculating Qtotal:
Qtotal=15.048kJ+240.48kJ+45.186kJ=300.714kJ
The total heat that must be absorbed by the ice to convert it to water at 15∘C is approximately 300.71kJ.
Topper's Solved these Questions
- BOOK - RESNICK AND HALLIDAYCHAPTER - HEAT-MEASUREMENT AND TRANSFEREXERCISE - SOLVED PROBLEM 19.022videos
HEAT-MEASUREMENT AND TRANSFER
- BOOK - RESNICK AND HALLIDAYCHAPTER - HEAT-MEASUREMENT AND TRANSFEREXERCISE - SOLVED PROBLEM 19.031videos
HEAT-MEASUREMENT AND TRANSFER
- BOOK - RESNICK AND HALLIDAYCHAPTER - GRAVITATIONEXERCISE - PRACTICE QUESTIONS (INTEGER TYPE)4videos
GRAVITATION
- BOOK - RESNICK AND HALLIDAYCHAPTER - HYDROGEN ATOMEXERCISE - PRACTICE QUESTIONS(Integer Type)6videos
HYDROGEN ATOM
Similar Questions
Knowledge Check
- Question 1 - Select One
How much heat energy must be supplied to change36 g of ice at 0∘C to Water at room temperature 25∘C?
Data for Water
ΔH∘fusion=9Kj/mol,cp(liquid)=4JK−1g−1A18KJB3.6KjC22KJD21.6Kj - Question 2 - Select One
How much heat is reuired to convert 5.0 g of ice at −10.0∘ C to liquid water at 15.0∘ C ? (Assume heart capacities are indendent o ftemperature.)
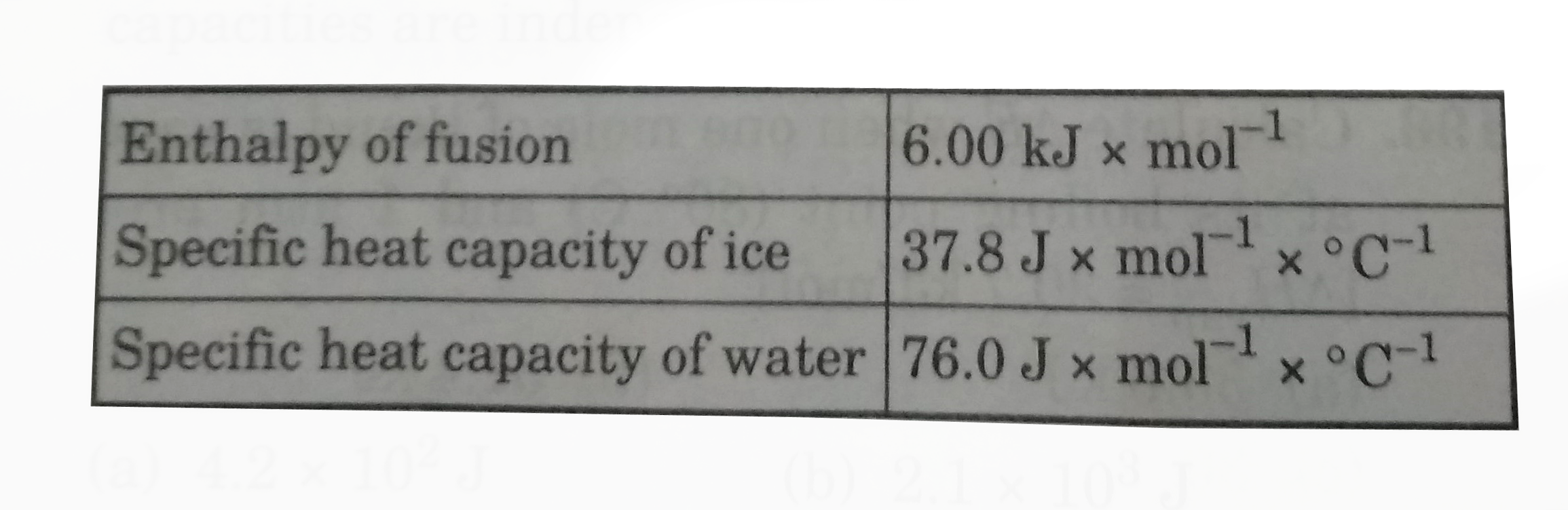 A4.2×102jB2.1×103JC9.3×103JD3.8×104J
A4.2×102jB2.1×103JC9.3×103JD3.8×104J - Question 3 - Select One
How much energy must be supplied to change 36g of ice at 36g of ice at 0∘C water at room temperture , 25∘C?
 A12kJB16kJC−210kJD22kJ
A12kJB16kJC−210kJD22kJ
How much heat should be supplied to convert ice of mass 250 g at 0∘C into water at 20∘C?
View SolutionHow much heat should be supplied to convert ice of mass 250 g at 0∘C into water at 20∘C?
View SolutionCalculate the amount of heat that must be absorbed by 5g of ice at 120∘C to convert it to water at 2∘C. Use the specfic heat of ice (2.09Jg−11∘C−1), enthalpy of fusion of ice (334Jg−1), and specific heat of water (4.18Jg−1.∘C−1) for calculation
Strategu : We must calculate the amount of heat absorbed during three steps. ltbrrgt (i) Wariming 50g of ice from −12∘C to its melting point 0∘C. This requires the specific heat of ice.
Melting the ice with no change in temperature . This needs the entholpy of fusion of ice at 0∘C.
Warming the resulting liquid from 0∘C to 20∘C. This requires specific heat of water
50gIce at−12∘CStep1−−→50gIce at0∘CSetp2−−→50gWater at0∘CSetp3−−→50gWater at20∘CView SolutionHow many calories of heat will be absorbed when 3 kg of ice at 0∘C melts ?
View SolutionHow much energy must be supplied to change 36 g of ice at 0∘C to water at room temperature 25∘C? Data for waterΔH∘fusion6.01kJ/molCP liquid4.18JK−1g−1
View Solution

