(i) A harmonic wave in a stationary medium is represented by y=asin(kx–ωt). Write the equation of this wave for an observer who is moving in negative x direction with constant speed v0.
(ii) The Doppler flow meter is a device that measures the speed of blood flow, using transmitting and receiving elements that are placed directly on the skin. The transmitter emits a continuous sound wave whose frequency is 5MHz. When the sound is reflected from the red blood cells, its frequency is changed in a kind of Doppler effect. The cells are moving with the same velocity as the blood. The receiving element detects the reflected sound, and an electronic counter measures its frequency, which is Doppler- shifted relative to the transmitter frequency. From the change in frequency the speed of the blood flow can be determined. Typically, the change in frequency is around 600Hz for flow speeds of about 0.1m/s. Assume that the red blood cell is directly moving away from the source and the receiver. (a) Estimate the speed of the sound wave in the blood?
(b) A segment of artery is narrowed down by plaque to half the normal cross-sectional area. What will be the Doppler change in frequency due to reflection from the red blood cell in that region?
The correct Answer is:(i) y=asin[(ωkv0)t−kx]
(ii) (a) 1700 m/s
(b) 1200 Hz
Step by step video, text & image solution for (i) A harmonic wave in a stationary medium is represented by y = a sin (kx – omegat). Write the equation of this wave for an observer who is moving in negative x direction with constant speed v_(0). (ii) The Doppler flow meter is a device that measures the speed of blood flow, using transmitting and receiving elements that are placed directly on the skin. The transmitter emits a continuous sound wave whose frequency is 5 M Hz. When the sound is reflected from the red blood cells, its frequency is changed in a kind of Doppler effect. The cells are moving with the same velocity as the blood. The receiving element detects the reflected sound, and an electronic counter measures its frequency, which is Doppler- shifted relative to the transmitter frequency. From the change in frequency the speed of the blood flow can be determined. Typically, the change in frequency is around 600 Hz for flow speeds of about 0.1 m//s. Assume that the red blood cell is directly moving away from the source and the receiver. (a) Estimate the speed of the sound wave in the blood? (b) A segment of artery is narrowed down by plaque to half the normal cross-sectional area. What will be the Doppler change in frequency due to reflection from the red blood cell in that region? by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A long taut string is plucked at its centre. The pulse travelling on it can be described as y(x,t)=e−(x+2t)2+e−(x−2t)2. Draw the shape of the string at time t=0, a short time after t=0 and a long time after t=0.
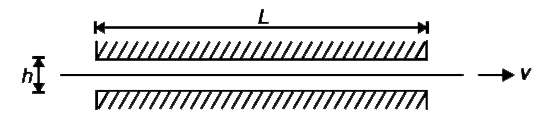
View SolutionDuring a painting process, a thin, flat tape of width b (dimension perpendicular to the plane of the figure) is pulled through a paint filled channel of length L. The density and viscosity of the paint liquid is ρ and η respectively. The tape is pulled at a constant speed v and width of the channel is h. Find the minimum force needed to pull the tape.
View Solution(i) The cause of increases in kinetic energy when a man starts running without his feet slipping on ground is asked to two students. Their answers are– Harshit: Cause of increase in kinetic energy is work done by friction force. Without friction the man cannot run. Akanksha: Cause of increase in kinetic energy is work done by internal (muscle) forces of the body. Who is right? (ii) An inextensible rope is hanging from a tree. A monkey, having mass m, climbs to a height h grabbing the rope tightly. The monkey starts from rest and ends up hanging motionlessly on the rope at height h. (a) How much work is done by gravity on the monkey? (b) How much work is done by the rope on the monkey? (c) Using work – energy theorem, explain the increase in mechanical energy of the monkey.
View SolutionA wave representing by the equation y = a cos(kx - omegat) is superposed with another wave to form a stationary wave such that x = 0 is a node. The equation for the other wave is
View SolutionWhich of the following equations can form stationary waves? (i) y= A sin (omegat - kx) (ii) y= A cos (omegat - kx) (iii) y= A sin (omegat + kx) (iv) y= A cos (omegat - kx) .
View SolutionA harmonic wave is travelling in a stationary medium whose equation of this wave w.r.t. a frame which is moving along -ve x-axis with a constant speed v w.r.t. stationary medium. Also, find speed of wave in moving frame.
View SolutionIn a stationary wave represented by y=asinωtcoskx, amplitude of the component progerssive wave is
View SolutionIn a stationary wave represented by y=asinωtcoskx, amplitude of the component progerssive wave is
View SolutionA simple harmonic progressive wave is given by y=Asin(omegat-kx) . What is (i) the particle velocity at a point x and time t (ii) the wave speed ?
View Solutionसमीकरण y=Asin2(kx−ωt) एक तरंग गति को निरूपित करता है जिसके आयाम और आवृत्ति है, क्रमश :
View SolutionA wave represented by the equation y=acos(kx−ωt) is superposed with another wave to form stationary wave such that the point x=0 is a node. The equation for the other wave is:
View SolutionA wave represented by the equation y=acos(kx−ωt) is superposed with another wave to form stationary wave such that the point x=0 is a node. The equation for the other wave is:
View SolutionThe transverse wave moving along the positive x-axis y=Asin(kx-omegat) Is given by This is the second wave moving along the negative x-axis, y= -A sin(kx+omegat) Is given by, imputed with. The point is x = 0
View SolutionA wave represented by the equation y=acos(kx−ωt) is superimposed with another wave to form a stationary wave such that point x=0 is a node. The equation for the other wave is
View SolutionA wave represented by the equation y=acos(kx−ωt) is superposed with another wave to form stationary wave such that the point x=0 is a node. The equation for the other wave is:
View Solution
