Ideal gas equation for n mole of gasSolution in Tamil
Boyle.s law: For a given gas at low pressure (density) kept in a container of volume Y, experiments revealed the following information.
When the gas is kept at constant temperature, the pressure of the gas is inversely proportional to the volume P∝1V
Charles law: When the gas is kept at constant pressure, the volume of the gas is directly proportional to absolute temperature V∝T.
By combining these two equations we have PV CT. Here C is a positive constant. We can infer that C is proportional to the number of particles in the gas container by considering the following argument. If we take two containers of same type of gas with same volume V, same pressure P and same temperature T, then the gas in each container obeys the above equation. PV = CT. If the two containers of gas is considered as a single system, then the pressure and temperature of this combined system will be same but volume will be twice and number of particles will also be double.
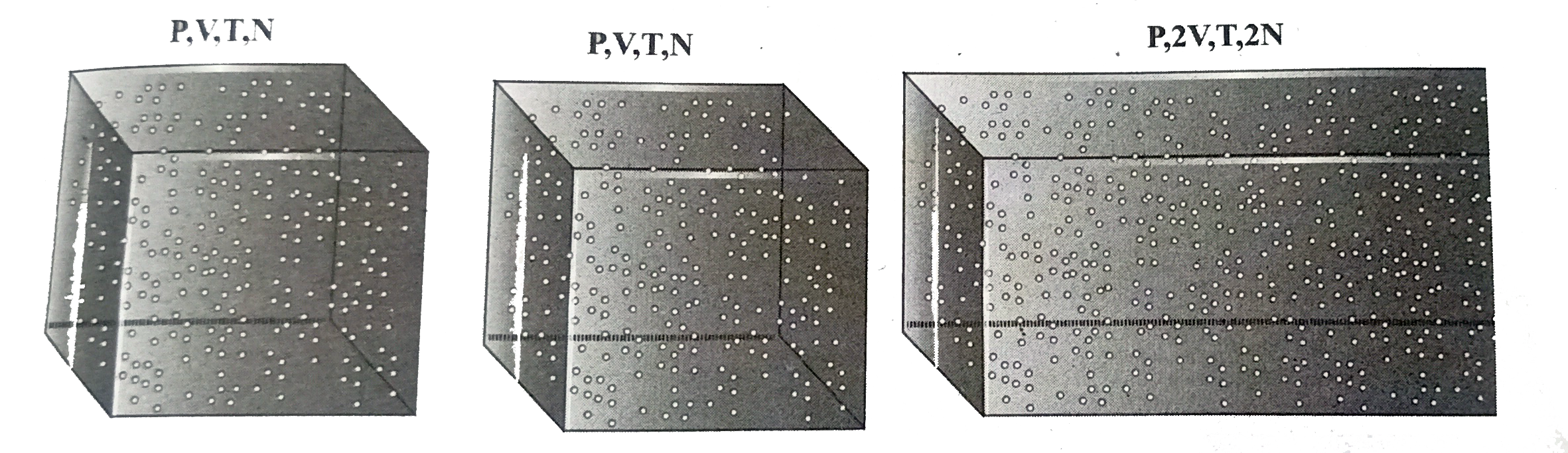
For this combined system, V becomes 2V, so C should also double to match with the ideal gas equation P(2V)T=2C. It implies that C must depend on the number of particles in the gas also should have the dimension of [PVT]=JK−1. So we can write the constant C as k times the umber of particles N.
Here λ is the Boltznnann constant (1.381×1023JK−1) and it is found to be a universal constant, So the idcal gas law can be stated as follows
PV =NKT
The equation (1) can also be expressed in terms of mole. Suppose if a gas contains μ mole of particles then the total number of particles can be written as N=μNA where NA is Avogadro number (6.023×1023mol−1) Substiuting for N from equation (2), the equation (1) becomes PV=μNAkT. Here NAk=R called universal gas constant and its value is 8.314J/mol.K
So the ideal gas law can be written for μ mole of gas as
P=μRT
.(3) This is called the equation of state for an ideal gas. It relates the pressure, volume and temperature of thermodynamic system at equilibrium.
Topper's Solved these Questions
Similar Questions
What are the limitations of dimensional analysis?
00:43View SolutionThe force F acting on a body moving in a circular path depends on mass of the body (m) velocity(v) and radius (r) of the circular path. Obtain the expression for the force by dimensional analysis method (k=1)
03:44View SolutionFor two mole of an ideal gas :
01:54View SolutionFor n moles of ideal gas, the equation of state may be written as
05:11View SolutionWhat is the equation of state of an ideal gas for n moles ?
02:32View Solutionआदर्श गैस से आप क्या समझते है ? गैस के किसी एक मोल के लिये आदर्श गैस समीकरण लिखिए |
03:43View Solutionएक मोल आदर्श गैस का अवस्था समीकरण लिखिए।
03:17View SolutionGive the expression of kinetic gas equation for n mol of ideal gas.
01:20View SolutionFor n moles of an ideal gas, the ideal equation may be written as "_____________" .
01:00View SolutionWrite ideal gas equation for one mole of gas.
01:20View SolutionGive the ideal gas equation for n moles of a gas.
02:05View SolutionWrite ideal gas equation for one mole of gas.
04:06View SolutionIdeal gas equation for n mole of gas
05:20View SolutionDerive an equation for n moles of an ideal gas.
01:40View SolutionDistinguish between ideal gas and real gas. Write an equation for one mole of an ideal gas.
02:46View SolutionFor two mole of an ideal gas:
01:47View Solution

