Identical springs of stecl and copper (YSteel>Ycopper) are equally stretched.
 Less work is done on copper spring.
Less work is done on steel spring.
Equal work is cone on both the springs.
Data is incomplete.
The correct Answer is:B
To solve the problem, we need to analyze the work done on identical springs made of steel and copper when they are equally stretched. We know that the Young's modulus of steel is greater than that of copper.
1. Understanding Work Done on Springs:
The work done (W) on a spring when it is stretched can be expressed as:
W=F⋅ΔL2
where F is the force applied and ΔL is the extension of the spring.
2. Relating Force and Extension to Young's Modulus:
Young's modulus (Y) is defined as:
Y=F⋅LA⋅ΔL
Rearranging this equation gives us:
ΔL=F⋅LA⋅Y
3. Substituting Extension into Work Done:
Now, we can substitute ΔL into the work done equation:
W=F⋅(F⋅LA⋅Y)2
Simplifying this gives:
W=F2⋅L2⋅A⋅Y
4. Analyzing the Relationship:
From the equation W=F2⋅L2⋅A⋅Y, we can see that the work done is inversely proportional to Young's modulus (Y):
W∝1Y
This means that as Young's modulus increases, the work done decreases.
5. Comparing Steel and Copper:
Given that Ysteel>Ycopper, we can conclude:
Wsteel<Wcopper
Therefore, less work is done on the steel spring compared to the copper spring.
6. Final Conclusion:
Since the work done on the steel spring is less than that on the copper spring, the correct answer is that less work is done on the steel spring.
The work done (W) on a spring when it is stretched can be expressed as:
W=F⋅ΔL2
where F is the force applied and ΔL is the extension of the spring.
Young's modulus (Y) is defined as:
Y=F⋅LA⋅ΔL
Rearranging this equation gives us:
ΔL=F⋅LA⋅Y
Now, we can substitute ΔL into the work done equation:
W=F⋅(F⋅LA⋅Y)2
Simplifying this gives:
W=F2⋅L2⋅A⋅Y
From the equation W=F2⋅L2⋅A⋅Y, we can see that the work done is inversely proportional to Young's modulus (Y):
W∝1Y
This means that as Young's modulus increases, the work done decreases.
Given that Ysteel>Ycopper, we can conclude:
Wsteel<Wcopper
Therefore, less work is done on the steel spring compared to the copper spring.
Since the work done on the steel spring is less than that on the copper spring, the correct answer is that less work is done on the steel spring.
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - APPLICATIONS OF ELASTIC BEHAVIOUR OF MATERIALS
8 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - HOTS
8 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - STRESS-STRAIN CURVE
5 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - NCERT Exemplar
5 Videos
- MOTION IN A PLANE BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MOTION IN A PLANE EXERCISE - Assertion And Reason
15 Videos
Similar Questions
Knowledge Check
A spring is made of steel and not of copper because
Asteel is more elastic than copperBsteel is less elastic than copperCsteel or less plastic than copperDsteel is less plastic than copperTwo springs A and B are identical except that A is stiffer than B i.e., kA>kB. If the two springs are stretched by the same force, then
Amore work is done on B i.e., WB>WABmore work is done on A i.e., WA>WBCwork done on A and B are equalDwork done depends upon the way in which they are stretched
Why are springs manufactured by using steel and not copper?
View SolutionTwo exactly similar wires of steel and copper are stretched by equal force. If the total elongation is 2 cm, then how much is the elongation in steel and copper wire respectively? Given, Ysteel=20×1011dyne/cm2,Ycopper=12×1011Dyne/cm2
View SolutionA steel bolt is insertede into a copper tube as shown in the figure. Find the forces induced in the bolt and in the tube when the nut is turned through one revolution. Assume that the length of the tube is l, the pitch of the bolt thread is h and the cross sectional areas of the steel bolt and the copper tube are As and Ac respectively.

View SolutionTwo vertical rods of equal lengths, one of steel and the other of copper, are suspended from the ceiling at a distance l apart and are connected rigidly to a rigid horizontal bar at their lower ends. If AS and AC be their respective cross-sectional areas, and YS and YC,their respective Young's moduli of elasticities, where should a vertical force F be applied to the horizontal bar in order that the bar remains horizontal?
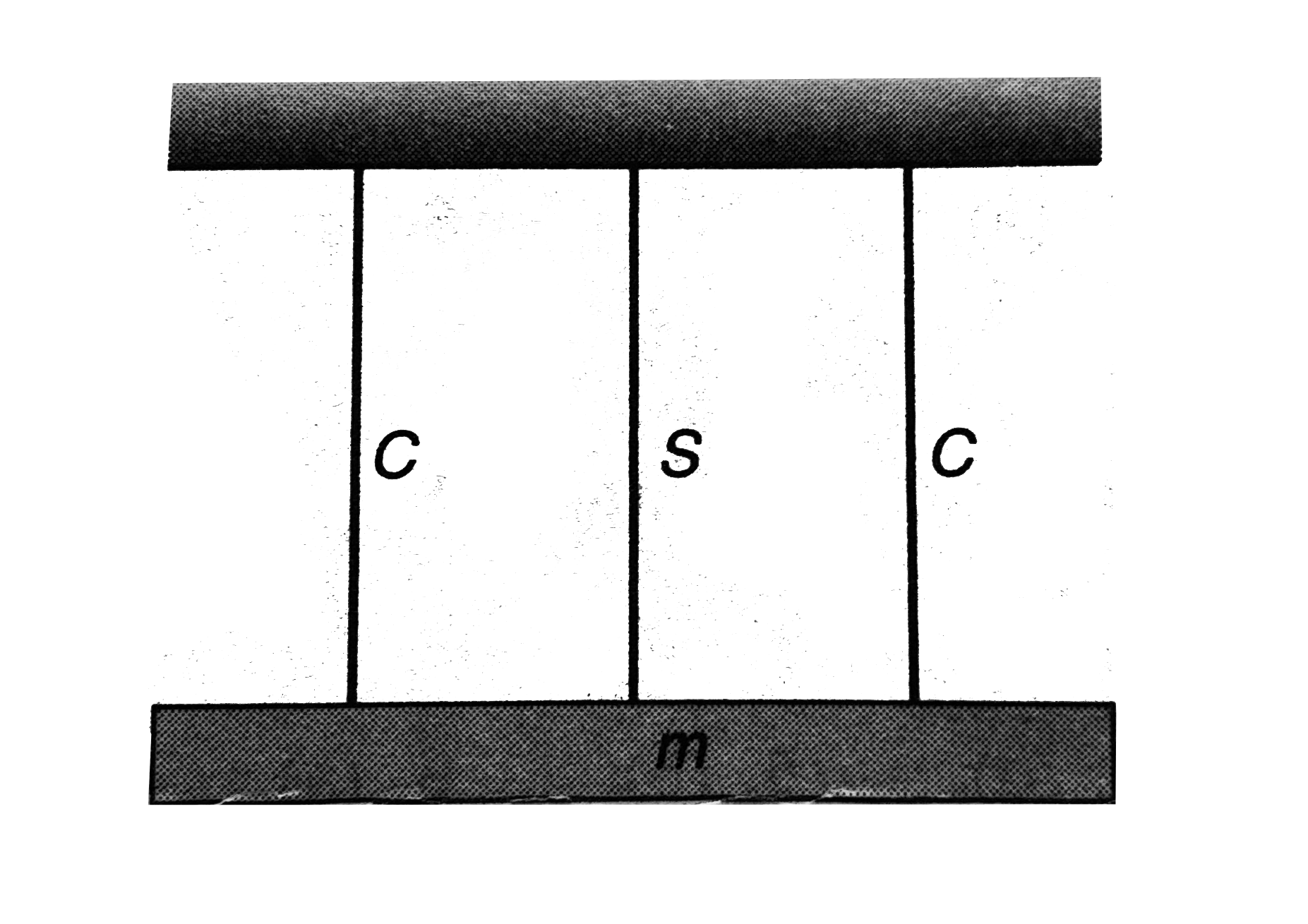
View SolutionA homogeneous block with a mass m hangs on three vertical wires of equal length arranged symmetrically . Find the tensions of the wires if the middle wire is of steel and the other two are of copper . All the wires have the same cross - section . Consider the modulus of elasticity of steel to be double than that of copper .
View SolutionTwo identical rectangular strips. One of copper and the other of steel, are riveted together to from a bimetallic strip. On heating. the strip will
(αcopper>αsteel)
View Solution
