If a wire of length 4 m and cross-sectional area of 2 m2 is stretched by a force of 3 kN, then determine the change in length due to this force, Given, Young's modulus of material of wire is 110×109Nm−2
60.2×10−3mm
54.5×10−6mm
56.4×10−3mm
62.5×10−3mm
Given, area of cross-section, A=2m2
Force, F=3kN=3×103N
Length L = 4m
Young's modules, Y=110×109Nm−2
Change in length ΔL=?
Apply, Y=StressStrain=F/AΔLL=FLAΔL
⇒ΔL=FLAY=3×103×42×110×109
=0.0545×10−6m=54.5×10−6mm
Topper's Solved these Questions
- ELASTICITY BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - ELASTICITY EXERCISE - EXERCISE 1
66 Videos
- ELASTICITY BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - ELASTICITY EXERCISE - EXERCISE 2
48 Videos
- CURRENT ELECTRICITYBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - CURRENT ELECTRICITYEXERCISE - MHT CET CORNER
32 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - MHT CET CONER
34 Videos
Similar Questions
If a wire of length 4 m and cross-sectional area of 2m2 is stretched by a force of 3 kN, then determine the change in length due to this force . Given, Young's modulus of material of wire is 110×109Nm−2
View SolutionA body of mass 3.14 kg is suspended from one end of a non uniform wire of length 10m, such that the radius of the wire is changing uniformly from 9.8×10−4 m at one end to 5×10−4 m at the other end. Find the change in length of the wire in mm. Young's modulus of the material of the wire is 2×1011N/m2 (mass of wire is negligible)
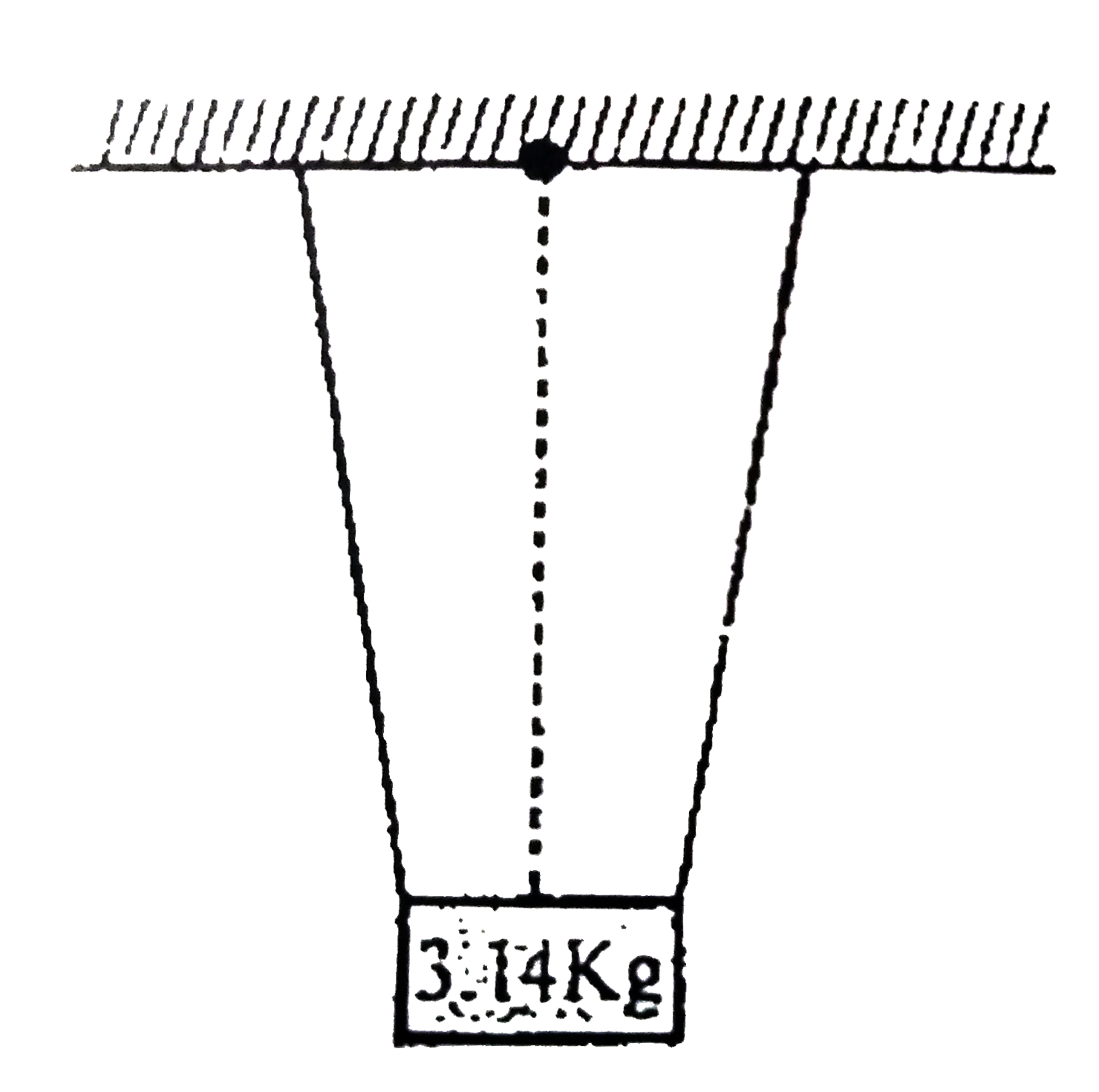
View Solution
Knowledge Check
A wire of length 10 m and cross-section are 10−6m2 is stretched with a force of 20 N. If the elongation is 1 mm, the Young's modulus of material of the wire will be
A1010N/m2B1011N/m2C2×1010N/m2D2×1011N/m2A wire 3m long and having cross - sectional area of 0.42m2 is found to stretched by 0.2 m under a tension of 1000 N. The value of young's modulus for the material of the wire is
A4.6×104N/m2B3.6×104N/m2C4×104N/m2D6.6×10−4N/m2When a wire 2 m long and 0.05cm2 in cross-section is stretched by a mass of 2 kg, it increases in length by 0.04 mm. Young's modulus of the material of the wire is
(g=10ms−2)A2×109Nm−2B20×1010Nm−2C20×1011Nm−2D20×108Nm−2
A steel wire of length 3.0m is stretched through 3.0 mm. the cross-sectional area of the wire is 5.0mm2. Calculate the elastic potential energy stroed in the wire in the stretched condition. Young's modulus of steel is 2.0×1011Nm−2.
View SolutionA metal wire 4m long and 2×10−7sq.m in cross-section is streched by a force of 30N. If the work done in streching that wire is 4.5×10−2J the young's modulus of the wire is
View SolutionA wire suspended vertically from one of its ends is stretched by attaching a weight of 20 N to its lower end. If its length changes by 1% and if the Young's modulus of the material of the wire is 2×1011N/m2, then the area of cross section of the wire is
View SolutionA wire of length L and area of cross-section A, is stretched by a load. The elongation produced in the wire is I. If Y is the Young's modulus of the material of the wire, then the torce corstant of the wire is
View SolutionA wire of 10m long and 1mm2 area of cross section is strechted by a force of 20N. If the elongation is 2mm the young's modulus of the material of the wire (in Pa) is
View Solution
