If displacement x and velocity v related as
4v2=25−x2m in a SHM Then time period of given SHM is (consider SI unit)
π
2π
4π
6π
The correct Answer is:C
To find the time period of the simple harmonic motion (SHM) given the relationship between displacement x and velocity v as 4v2=25−x2, we can follow these steps:
Step 1: Differentiate the given equation
We start with the equation:
4v2=25−x2
Differentiating both sides with respect to time t:
ddt(4v2)=ddt(25−x2)
This gives us:
4⋅2vdvdt=0−2xdxdt
Simplifying, we have:
8vdvdt=−2xdxdt
Step 2: Substitute acceleration and velocity
We know that:
- dvdt is the acceleration a
- dxdt is the velocity v
Substituting these into the equation gives:
8va=−2xv
If we divide both sides by v (assuming v≠0):
8a=−2x
Step 3: Express acceleration in terms of displacement
Rearranging the equation:
a=−28x=−14x
This shows that the acceleration is proportional to the displacement x and is directed opposite to it, which is characteristic of SHM.
Step 4: Relate to the standard SHM equation
In SHM, the acceleration can also be expressed as:
a=−ω2x
Comparing the two expressions for acceleration:
−14x=−ω2x
This implies:
ω2=14
Taking the square root gives:
ω=12
Step 5: Calculate the time period
The time period T of SHM is given by the formula:
T=2πω
Substituting the value of ω:
T=2π12=4π
Conclusion
Thus, the time period of the given SHM is:
4π
We start with the equation:
4v2=25−x2
Differentiating both sides with respect to time t:
ddt(4v2)=ddt(25−x2)
This gives us:
4⋅2vdvdt=0−2xdxdt
Simplifying, we have:
8vdvdt=−2xdxdt
We know that:
- dvdt is the acceleration a
- dxdt is the velocity v
8va=−2xv
If we divide both sides by v (assuming v≠0):
8a=−2x
Rearranging the equation:
a=−28x=−14x
This shows that the acceleration is proportional to the displacement x and is directed opposite to it, which is characteristic of SHM.
In SHM, the acceleration can also be expressed as:
a=−ω2x
Comparing the two expressions for acceleration:
−14x=−ω2x
This implies:
ω2=14
Taking the square root gives:
ω=12
The time period T of SHM is given by the formula:
T=2πω
Substituting the value of ω:
T=2π12=4π
Thus, the time period of the given SHM is:
4π
Topper's Solved these Questions
- BOOK - A2ZCHAPTER - OSCILLATION AND SIMPLE HARMONIC MOTIONEXERCISE - Velocity , Acceleration And Energy Of Simple Harmonic Motion40videos
OSCILLATION AND SIMPLE HARMONIC MOTION
- BOOK - A2ZCHAPTER - OSCILLATION AND SIMPLE HARMONIC MOTIONEXERCISE - Spring Particle System24videos
OSCILLATION AND SIMPLE HARMONIC MOTION
- BOOK - A2ZCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - Chapter Test30videos
NEWTONS LAWS OF MOTION
- BOOK - A2ZCHAPTER - PROPERTIES OF MATTEREXERCISE - Chapter Test29videos
PROPERTIES OF MATTER
Similar Questions
If the displacement (x) and velocity (v) of a particle executing SHM are related through the expression 3v2=30−x2. If the time period of the particle is T=π√n, then what is the value of n?
View SolutionIf the distance y of a point moving on a straight line measured from a fixed origin on it and velocity v are connected by the relation 4v2=25−y2, then show that the motion is simple harmonic and find its time period.
View Solution
Knowledge Check
- Question 1 - Select One
Dispalcement (x) and velocity (v) of a particle exectuting S.H.M are related as 2v2=9−x2. The time period of particle is
A4πB2πC4√2πD2√2π - Question 2 - Select One
If the displacement x and velocity v of a aprticle executing SHM are related as 4v2=25−x2. Then its maximum displacement in metre (x,v are in SI) is
A1B2C5D6 - Question 3 - Select One
A particle is performing a linear S.H.M about the mean position with the equation of velocity given by 4v2−25−x2. Then the period of motion is
A2πBπC3πD4π
A particle performing S.H.M. about their mean position with the equation of velocity is given by 4v2=25−x2, then the period of motion is
View SolutionIf x,v and a denote the displacement, the velocity and the acceleration of a particle executing simple harmonic motion of time period T, then, which of the following does not change with time ?
View SolutionA particle is executing SHM and its velocity v is related to its position (x) as v2+ax2=b, where a and b are positive constant. The frequency of oscillation of particle is
View SolutionAssertion : A particle performing SHM at certain instant is having velocity v. It again acquire a velocity v for the first time after a time interval of T second. Then the time period of oscillation is T second.
Reason : A particle performing SHM can have same velocity at two instants in one cycle.View SolutionThe displacement (x) is plotted with time (t) for a particle executing SHM as shown below. The correct equation of SHM is
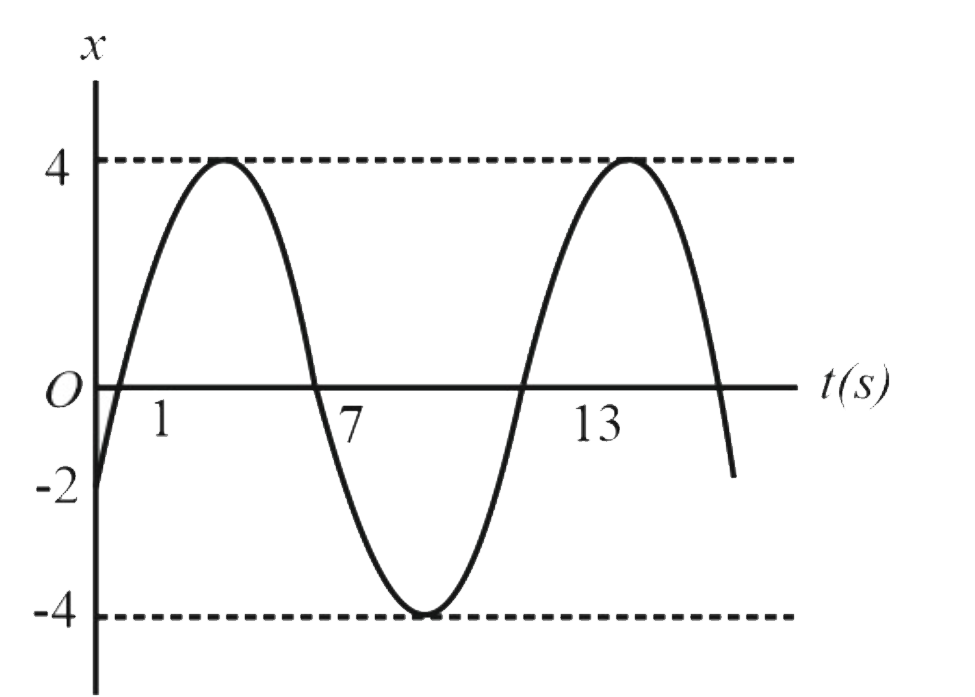 View Solution
View Solution

