If μ,R and S represent coefficient of friction, normal reaction and distance moved, then the general expression for work against friction
μ RS
μ+R/S
μR/S
μS/R
The correct Answer is:A
To derive the general expression for work done against friction, we can follow these steps:
1. Understand the Components:
- Let μ be the coefficient of friction.
- Let R be the normal reaction force.
- Let S be the distance moved.
2. Identify the Force of Friction:
- The force of friction F can be expressed as:
F=μR
This equation states that the frictional force is proportional to the normal force and the coefficient of friction.
3. Calculate Work Done Against Friction:
- Work done W against friction is given by the formula:
W=F×S
where F is the force of friction and S is the distance moved.
4. Substitute the Expression for Friction:
- Now, substituting F=μR into the work equation:
W=(μR)×S
5. Final Expression:
- Therefore, the general expression for work done against friction becomes:
W=μRS
Final Answer:
The general expression for work done against friction is:
W=μRS
- Let μ be the coefficient of friction.
- Let R be the normal reaction force.
- Let S be the distance moved.
- The force of friction F can be expressed as:
F=μR
This equation states that the frictional force is proportional to the normal force and the coefficient of friction.
- Work done W against friction is given by the formula:
W=F×S
where F is the force of friction and S is the distance moved.
- Now, substituting F=μR into the work equation:
W=(μR)×S
- Therefore, the general expression for work done against friction becomes:
W=μRS
The general expression for work done against friction is:
W=μRS
Similar Questions
Knowledge Check
the work done against force of friction is
A8.7 JB10.7 JC7.8 JD12.7 J
A 70 kg man stands in contact against the inner wall of a hollow cylindrical drum of radius 3 m rotating about its vertical axis with 200 rev/min. The coefficient of friction between the wall and his clothing is 0.15 m What is the minimum rotational speed of the cylinder to enable the man to remain stuck to the wall (without falling) when the floor is suddenly removed ?
View SolutionConsider Example 8, taking the coefficient of friction, μ, to be 0.5 and calculate the maximm compression of the spring.
View SolutionStatement I: The coefficient of friction can be greater than unity.
Statement II: The force of friction is dependent on normal reaction and the ration of force of friction and normal reaction cannot exceed unity.
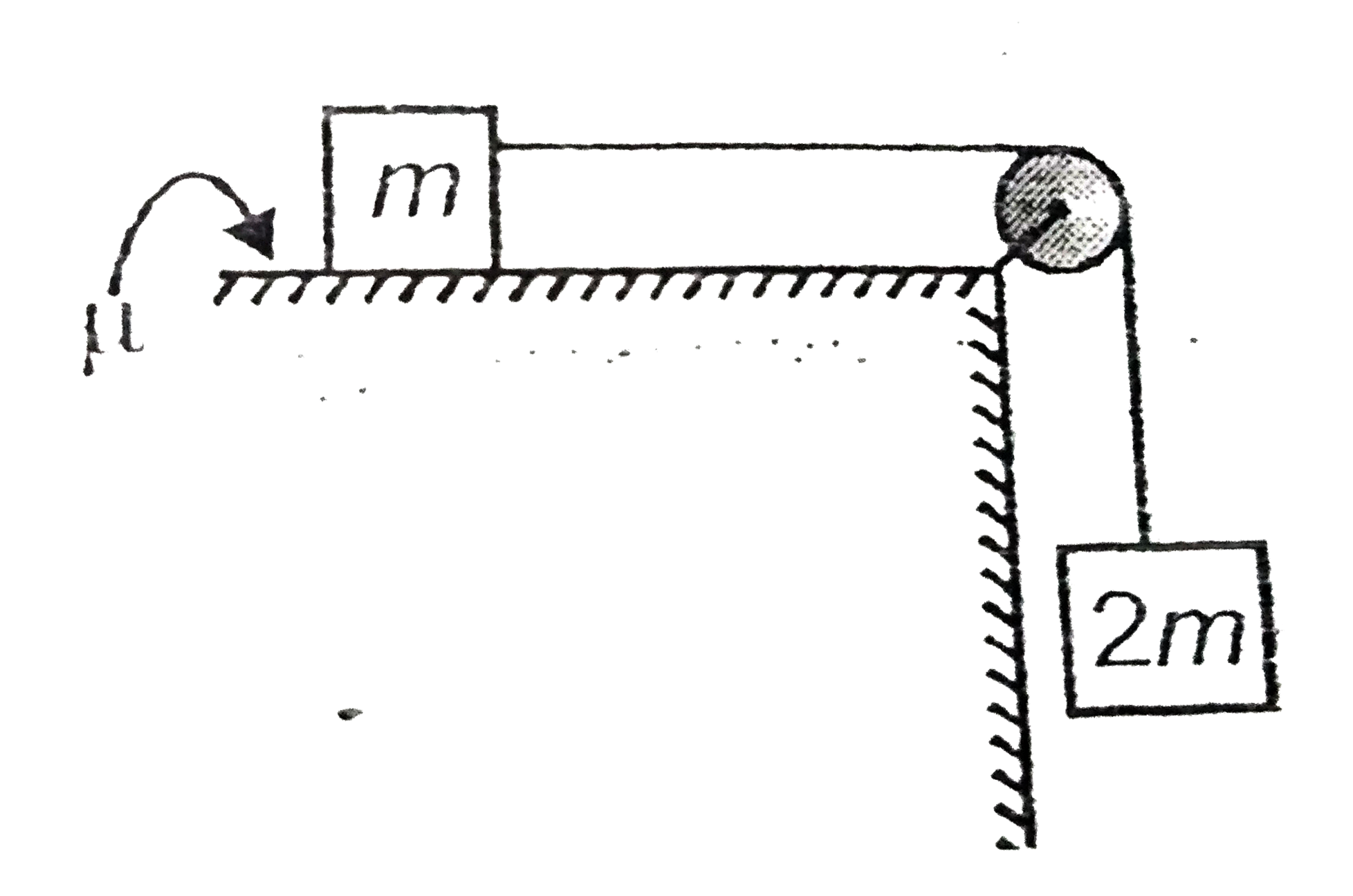
View SolutionConsider the system shown in the figure. Coefficient of friction between the block and table is μ=0.5. The system is released from rest. Find the work done by friction, when the speed of block is 10 m/s (m=1kg)
View SolutionA body is moving up an inclined plane of angle θ with an initial kinetic energy E. The coefficient of friction between the plane and body is μ. The work done against friction before the body comes to rest is
View SolutionA small coin of mass 40 g is placed on the horizontal surface of a rotating disc. The disc starts from rest and is given a constant angular acceleration α=2 rad s−2. The coefficient of static friction between the coin and the disc is μs=3/4 and the coefficient of kinetic friction is μk=0.5. The coin is placed at a distance r=1m from the centre of the disc. The magnitude of the resultant force on the coin exerted by the disc just before it starts slipping on the disc is?

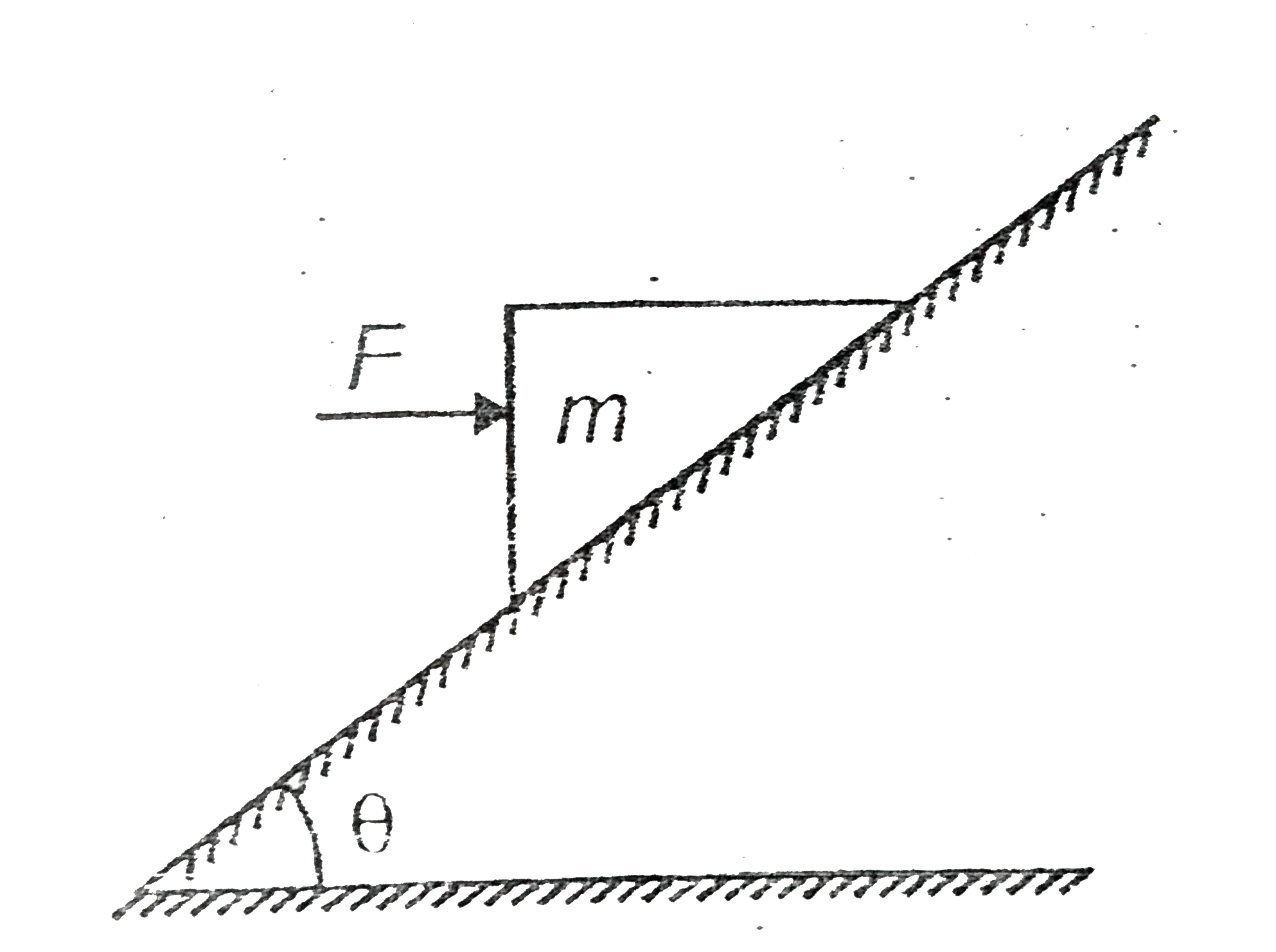
View SolutionA prismatic block of mass m is lifted through a distanced along the slant. If the coefficient of friction between the block and slant surface is 1μ. find the
(A) Work done by force F, friction and gravity.
(B) Speed of the block after moving through a distance d.
Assuming,μ=1/6,θ=45%∘andF=2mg
View Solution
