Consider Example 8, taking the coefficient of friction, μ, to be 0.5 and calculate the maximm compression of the spring.
To solve the problem of calculating the maximum compression of the spring when the coefficient of friction (μ) is 0.5, we can follow these steps:
1. Identify Given Values:
- Coefficient of friction, μ = 0.5
- Let the mass of the object be m.
- Let the gravitational acceleration be g (approximately 9.81 m/s²).
- Let the spring constant be k.
- Let the initial velocity of the object be v.
2. Understand Forces Acting on the Object:
- The spring force (F_spring) acts to compress the spring and is given by Hooke's law:
Fspring=k⋅xm
- The frictional force (F_friction) opposes the motion and is given by:
Ffriction=μ⋅m⋅g=0.5⋅m⋅g
3. Work Done by Forces:
- The work done by the spring force (W_spring) when compressed by a distance xm is:
Wspring=−12kx2m
- The work done against friction (W_friction) is:
Wfriction=−μ⋅m⋅g⋅xm=−0.5⋅m⋅g⋅xm
4. Apply the Work-Energy Theorem:
- The change in kinetic energy (ΔK) is equal to the total work done:
ΔK=Kfinal−Kinitial
- Since the object comes to rest at maximum compression, Kfinal=0 and Kinitial=12mv2:
ΔK=0−12mv2=−12mv2
5. Set Up the Equation:
- According to the work-energy theorem:
−12mv2=Wspring+Wfriction
- Substitute the expressions for work:
−12mv2=−12kx2m−0.5⋅m⋅g⋅xm
6. Rearrange the Equation:
- Rearranging gives:
12kx2m+0.5⋅m⋅g⋅xm−12mv2=0
7. Form a Quadratic Equation:
- This can be rewritten as:
12kx2m+(0.5⋅m⋅g)xm−12mv2=0
- This is a standard quadratic equation in the form ax2+bx+c=0 where:
- a=12k
- b=0.5⋅m⋅g
- c=−12mv2
8. Solve the Quadratic Equation:
- Using the quadratic formula:
xm=−b±√b2−4ac2a
- Substitute the values of a, b, and c to find xm.
9. Calculate Maximum Compression:
- After substituting the numerical values and solving, you should arrive at the maximum compression xm.
Final Answer:
The maximum compression of the spring xm is approximately 1.35 meters.
- Coefficient of friction, μ = 0.5
- Let the mass of the object be m.
- Let the gravitational acceleration be g (approximately 9.81 m/s²).
- Let the spring constant be k.
- Let the initial velocity of the object be v.
- The spring force (F_spring) acts to compress the spring and is given by Hooke's law:
Fspring=k⋅xm
- The frictional force (F_friction) opposes the motion and is given by:
Ffriction=μ⋅m⋅g=0.5⋅m⋅g
- The work done by the spring force (W_spring) when compressed by a distance xm is:
Wspring=−12kx2m
- The work done against friction (W_friction) is:
Wfriction=−μ⋅m⋅g⋅xm=−0.5⋅m⋅g⋅xm
- The change in kinetic energy (ΔK) is equal to the total work done:
ΔK=Kfinal−Kinitial
- Since the object comes to rest at maximum compression, Kfinal=0 and Kinitial=12mv2:
ΔK=0−12mv2=−12mv2
- According to the work-energy theorem:
−12mv2=Wspring+Wfriction
- Substitute the expressions for work:
−12mv2=−12kx2m−0.5⋅m⋅g⋅xm
- Rearranging gives:
12kx2m+0.5⋅m⋅g⋅xm−12mv2=0
- This can be rewritten as:
12kx2m+(0.5⋅m⋅g)xm−12mv2=0
- This is a standard quadratic equation in the form ax2+bx+c=0 where:
- a=12k
- b=0.5⋅m⋅g
- c=−12mv2
- Using the quadratic formula:
xm=−b±√b2−4ac2a
- Substitute the values of a, b, and c to find xm.
- After substituting the numerical values and solving, you should arrive at the maximum compression xm.
The maximum compression of the spring xm is approximately 1.35 meters.
Topper's Solved these Questions
Similar Questions
A block of mass m is attached to one end of a mass less spring of spring constant k. the other end of spring is fixed to a wall the block can move on a horizontal rough surface. The coefficient of friction between the block and the surface is μ then the compession of the spring for which maximum extension of the spring becomes half of maximum compression is .
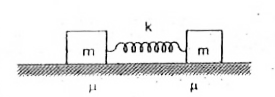
View SolutionTwo blocks each having mass m is attached to each other with a spring having force constant k. The system is placed on a rough horizontal surface having coefficient of friction μ. The maximum initial compression, that can be given to the spring so that when released the blocks do not move is
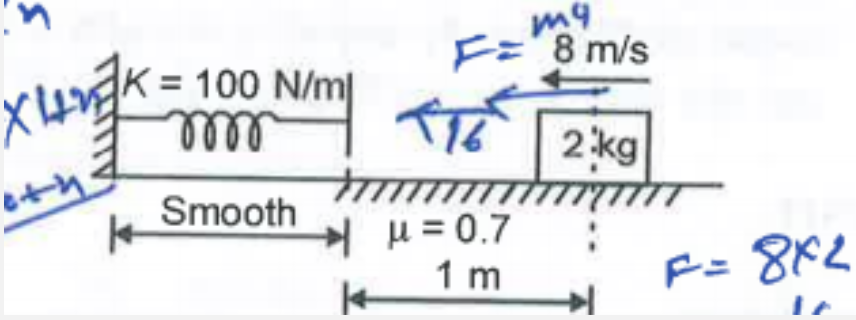
View SolutionFree end of an ideal spring of spring constant 100 N/m is at a distance 1 m from a vertical block of mass 2 kg moving on rough surface with initial speed 8 m/s as shown in figure. If coefficient of friction is 0.7, then maximum compression in spring is (g=10ms−2)
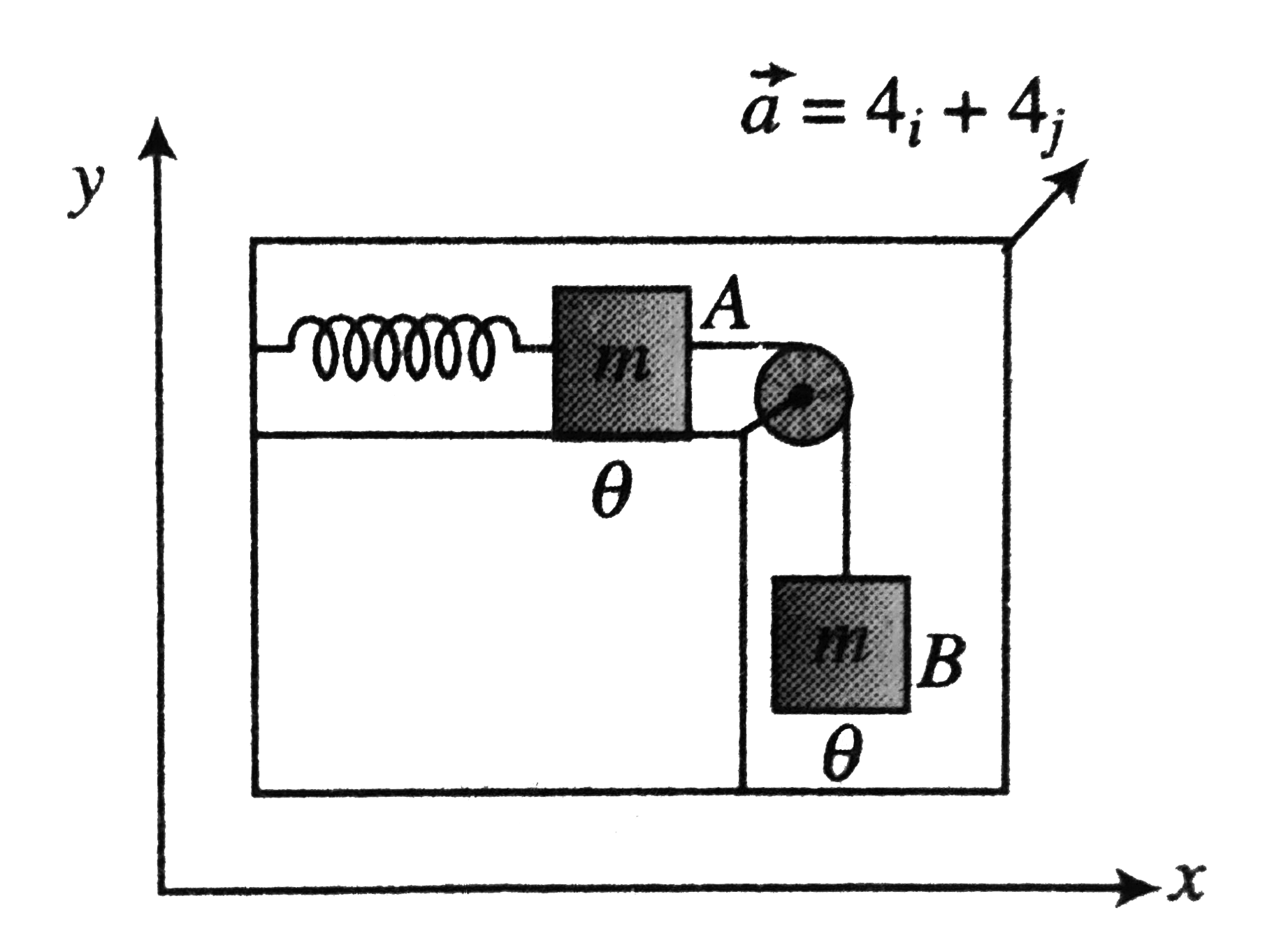
View SolutionThe arrangement shown in figure is at rest. An ideal spring of natural length l0 having spring constant k=220Nm−1, is connected to block A. Blocks A and B are connected by an ideal string passing through a friction less pulley. The mass of each block A and B is equal to m=2kg when the spring was in natural length, the whole system is given an acceleration →a as shown. If coefficient of friction of both surfaces is μ=0.25, then find the maximum extension in 10(m) of the spring. (g=10ms−2).
View SolutionThe coefficient of friction between two surface is 0.2. The maximum angle of friction is
View SolutionA force of 10N is acting on a block of 20kg on horizontal surface with coefficient of friction μ=0.2. Calculate the work done by the force.
View Solution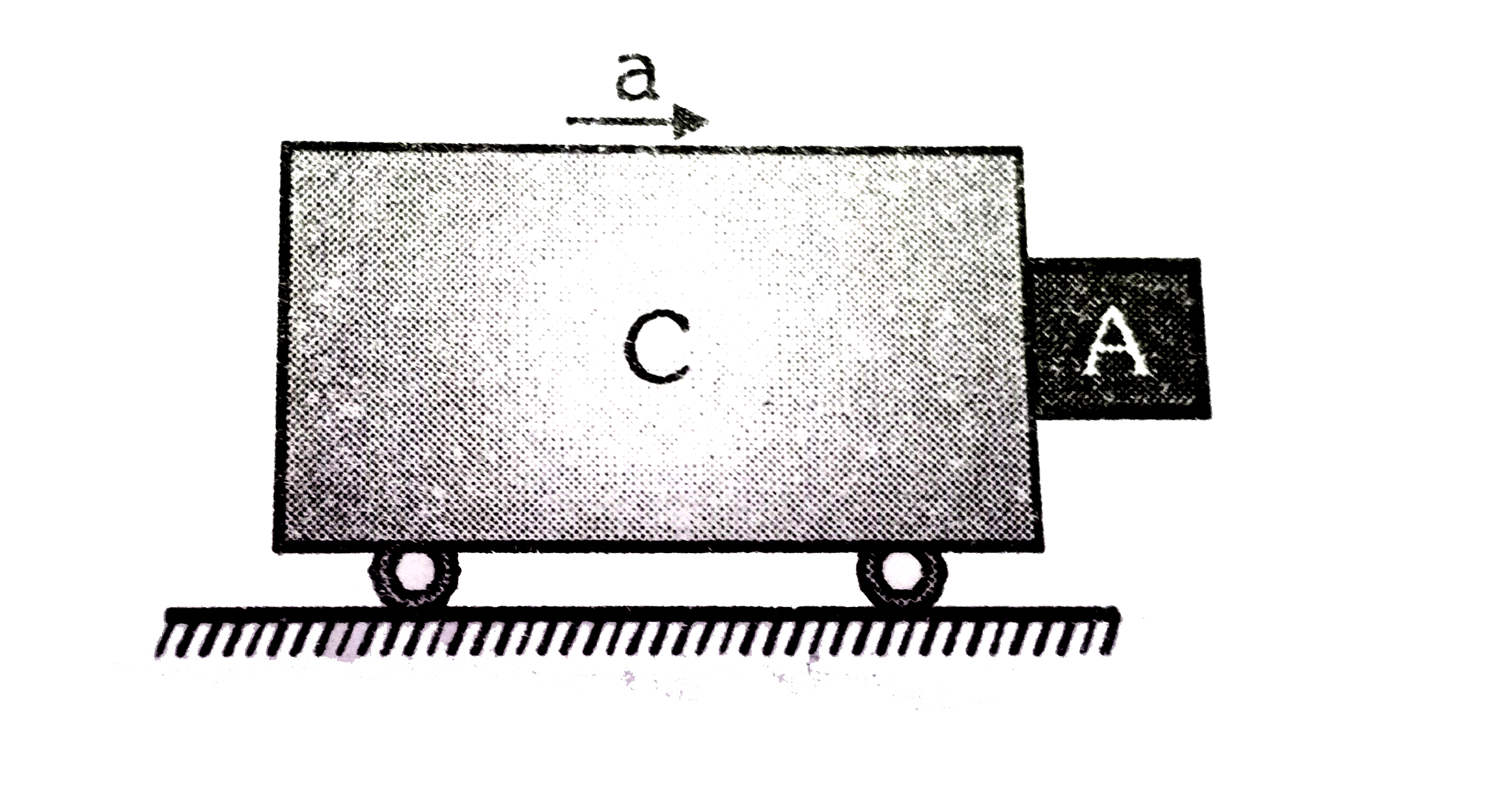
Consider the figure shown here of a moving cart C. if the coefficient of friction between the block A and the cart is μ, then calculate the minimum acceleration a of the cart C so that the block A does not fall.
View Solution .
.
In the figure shown, AB=BC=2m. Friction coefficient everywhere is μ=0.2. Find the maximum compression of the spring.
View SolutionA car is taking turn on a circular path of radius R. If the coefficient of friction between the tyres and road is μ, the maximum velocity for no slipping is
View SolutionBlocks A and B in the Fig are connected by a bar of negligible weight .Mass of each block is 170kg and μA=0.2 and μB=0.4 where μA and μBare the coefficient of limiting friction between bloock and plane calculate the force developed in the bar(g=10ms−2)
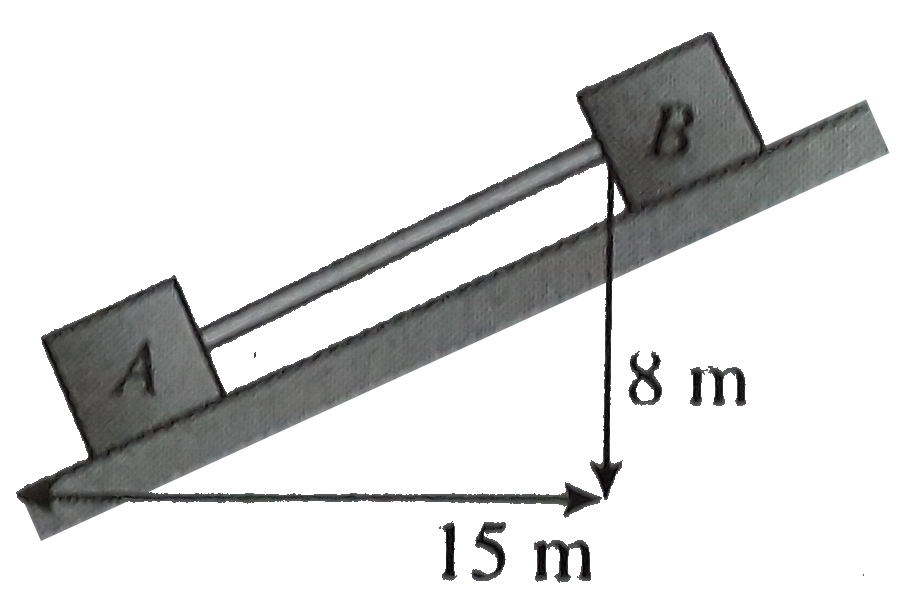
View Solution
