In a certain circuit current changes with time according to i=2√t RMS value of current between t=2s to t=4s will be
3A
3√3A
2√3A
(2−√2)A
The correct Answer is:A
To find the RMS (Root Mean Square) value of the current i=2√t between t=2s and t=4s, we will follow these steps:
Step 1: Understand the RMS formula
The RMS value of a current over a time interval is given by the formula:
IRMS=√1T∫t2t1i2dt
where T=t2−t1 is the duration of the time interval.
Step 2: Define the time interval
In this case, t1=2s and t2=4s. Therefore, the duration T is:
T=4−2=2s
Step 3: Substitute the current function
The current is given as i=2√t. We need to find i2:
i2=(2√t)2=4t
Step 4: Set up the integral
Now we substitute i2 into the RMS formula:
IRMS=√12∫424tdt
Step 5: Simplify the integral
Factor out the constant:
IRMS=√42∫42tdt=√2∫42tdt
Step 6: Calculate the integral
The integral ∫tdt is:
∫tdt=t22
Now evaluate it from 2 to 4:
∫42tdt=(t22)42=422−222=162−42=8−2=6
Step 7: Substitute back into the RMS formula
Now substitute the value of the integral back:
IRMS=√2⋅6=√12
Step 8: Simplify the result
Finally, simplify √12:
IRMS=√4⋅3=2√3
Final Answer
The RMS value of the current between t=2s and t=4s is:
2√3A
The RMS value of a current over a time interval is given by the formula:
IRMS=√1T∫t2t1i2dt
where T=t2−t1 is the duration of the time interval.
In this case, t1=2s and t2=4s. Therefore, the duration T is:
T=4−2=2s
The current is given as i=2√t. We need to find i2:
i2=(2√t)2=4t
Now we substitute i2 into the RMS formula:
IRMS=√12∫424tdt
Factor out the constant:
IRMS=√42∫42tdt=√2∫42tdt
The integral ∫tdt is:
∫tdt=t22
Now evaluate it from 2 to 4:
∫42tdt=(t22)42=422−222=162−42=8−2=6
Now substitute the value of the integral back:
IRMS=√2⋅6=√12
Finally, simplify √12:
IRMS=√4⋅3=2√3
The RMS value of the current between t=2s and t=4s is:
2√3A
Topper's Solved these Questions
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENTEXERCISE - Advance MCQs33videos
ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENT
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENTEXERCISE - Conceptual MCQs63videos
ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENT
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - CURRENT ELECTRICITYEXERCISE - All Questions389videos
CURRENT ELECTRICITY
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - ELECTROSTATICSEXERCISE - Unsolved Numberical Problems73videos
ELECTROSTATICS
Similar Questions
Knowledge Check
- Question 1 - Select One
In a certain circuit current changes with time accroding to i=2√t. r.m.s. value of current between t=2 to t=4s will be
A3AB3√3AC2√3AD(2−√2)A - Question 2 - Select One
The flux in a closed circuit of resistance 10Ω varies with time according to the equation ϕ=3t2−t+1, where ϕ is in weber and t is in , s the value of induced current at t=1 s is
A0.3 AB0.5AC0.7AD1A - Question 3 - Select One
If an alternating current is given by i = a sin (ωt) + b cos (ωt), then RMS value of current is :-
Aa√2+b√2Ba+b√2C√a2+b22Da2+b2√2
If the current in the ineer loop changes according to i=2t2 then, find the current in the capacitor as a function of time.
 View Solution
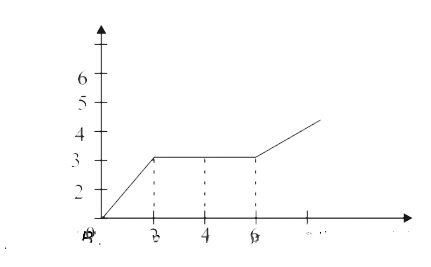
View SolutionThe charge on a capacitor plate in a circuit, as a function of time, is shown in the figure. What is the value of current at t=4s?
 View Solution
View SolutionFlux phi (in weber) in a closed circuit of resistance5 omega varies with time (in second) according to equation ϕ=3t2−5t+2. The magnitude of average induced current between0 to 2s is
View SolutionThe magnetic flux linked with a closed circuit of resistance 8Ω varies with time according to the expression ϕ=(5t2–4t+2) where ϕ is in milliweber and t in second. Calculate the value of induce current at t=15s.
View Solutionr.m.s. value of current i=3+4sin(ωt+π/3) is:
View Solution