In a certain region, uniform electric field $E = - E_{0} ˆ k$ and magnetic field $B = B_{0} ˆ k$ are present. At time $t = 0$ , a particle of mass in and charge q is given a velocity $v = v - 0 ˆ j + v - 0 ˆ k$ . Find the minimum speed of the particle and the time when it happens so.

Video Solution

Text Solution

Verified by Experts

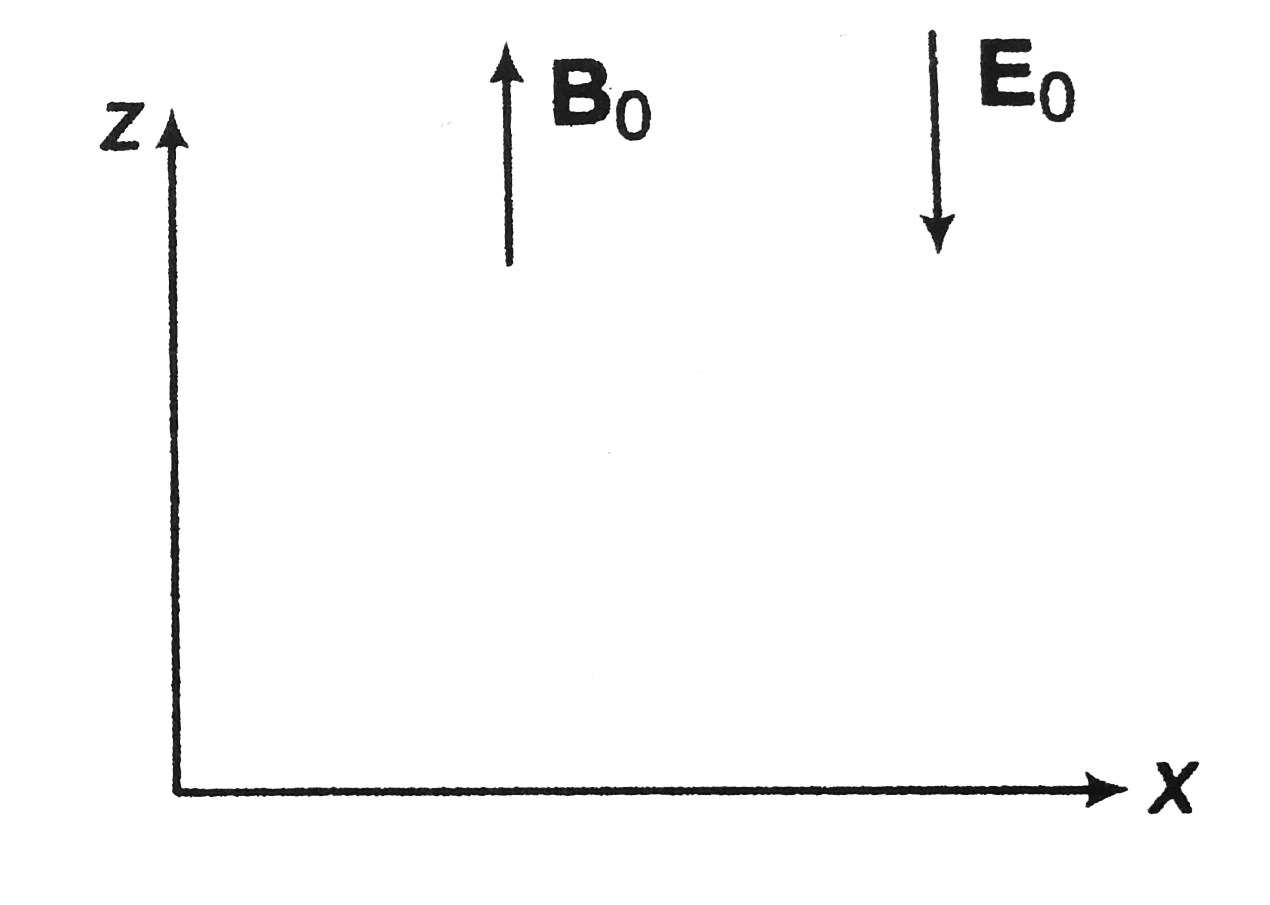
$a_{e} = \frac{q E_{0}}{m}$ (along negative z-direction)
Electric fiedl will make z - component of velocity zero. At that time speed of the particle will be minimum and that minimum, speed ils the other component i.e. $v_{0}$ .
$v_{z} = u_{z} + a_{z} t$
$or 0 = v_{0} - \frac{q E_{0}}{m} t$
$or t = \frac{m v_{0}}{q E_{0}}$

Updated on:21/07/2023

In a certain region uniform electric field E and magnetic field B are present in mutually opposite directions. At the instant $t = 0$ , a particle of mass m carrying a charge q is given velocity $v_{0}$ at angle $θ$ , with the $y -$ axis, in the yz plane. The time after which the speed of the particle would be minimum is equal to

A $\frac{m v_{0}}{q E}$
B $\frac{m v_{0} sin θ}{q E}$
C $\frac{m v_{0} cos θ}{q E}$
D $\frac{2 π m}{q B}$
In a certain region uniform electric field E and magnetic field B are present in mutually opposite directions. At the instant $t = 0$ , a particle of mass m carrying a charge q is given velocity $v_{0}$ at angle $θ$ , with the $y -$ axis, in the yz plane. The time after which the speed of the particle would be minimum is equal to

A $\frac{m V_{0}}{q E}$
B $\frac{m V_{0} sin θ}{q E}$
C $\frac{m V_{0} cos θ}{q E}$
D $\frac{2 π m}{q B}$
A particular region contains uniform electric and magnetic fields, $\to E = E_{0} ˆ i and \to B = B_{0} ˆ i$ respectively. When a particle having mass and charge m and q respectively, enters the region with velocity $\to v = v_{0} ˆ j$ , it is observed that the speed of the particle becomes $2 v_{0}$ after a time t. Find the value of t.
A $t = \frac{2 m v_{0}}{q E}$
B $t = \frac{2 B q}{m v_{0}}$
C $t = \frac{\sqrt{3} B q}{m v_{0}}$
D $t = \frac{\sqrt{3} m v_{0}}{q E}$