In a paricular crash test, a car of mass 1500 kg collies with a wall as shown in fig. The initial and final velocites on the car are →vi=−15.0ˆims−1 and →vf=5.00ˆims−1, respectively. If the collision lasts 0.150s, find the impulse caused by the collision and the average force exerted on the car.

The collision time is short, so we can imagine the car being brought to rest very rapidly and then moving back in the opposite direction with a raduced speed.
Let us assume that the force exerted by the wall on the car is large compred with other forces on the car (such as friction and air resistance). Furthermore, the gravitational force and the normal force exerted by the road on the car are perpendicular to the motion and , therefore, do not affect the horizontal momentum. Therefore, we categorize the problem as one in which we can apply the impusle approximation in the horizontal direction. Evaluating the inital and final momenta of the car, we get
→Pi=m→vi=(1500kg)(−15.0ˆims−1)
=−2.25×104ˆigms−1
→pf=m→vf=(1500kg)(5.0ˆims−1)
=0.75×104ˆikgms−1
Impulse on the car:
→J=Δ→p=→pf=→pi
=0.75×104ˆikgms−1−(−2.25×104ˆikgms−1)
⇒→J=300×104ˆikgms−1
The average force exerted by the wall on the car
→Favg=Δ→pΔt=3.00×104ˆikgms−10.150s=2.00×105ˆiN.
Topper's Solved these Questions
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Solved Examples
11 Videos
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Exercise 6.1
11 Videos
- MISCELLANEOUS VOLUME 2BOOK - CENGAGE PHYSICSCHAPTER - MISCELLANEOUS VOLUME 2EXERCISE - INTEGER_TYPE
10 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Integer type
1 Videos
Similar Questions
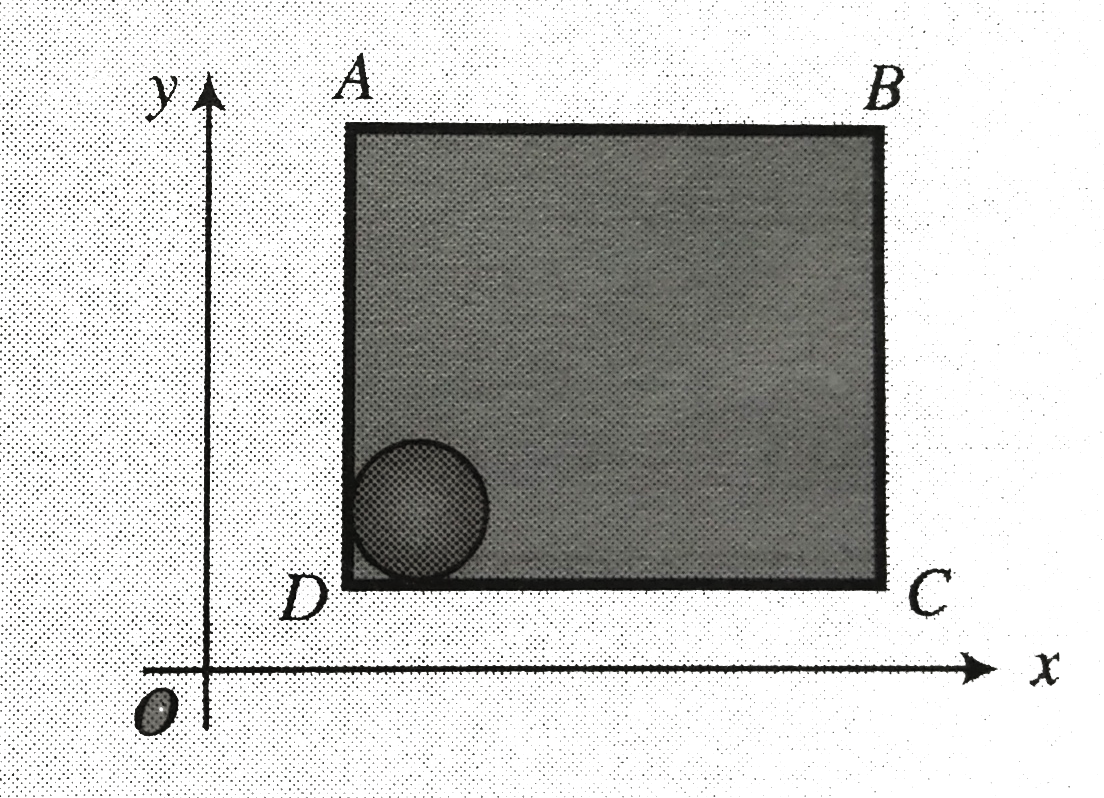
A solid sphere of mass 2 kg is resting inside a cube as shown in fig. The cube is moving with a velocity →v=(5tˆi+2tˆj)ms−1. Here t is time in seconds. All surface are smooth. The sphere is at rest with respect to the cube. What is the total force (in N) exerted by the sphere on the cube?
View SolutionA cricket ball of mass 150 g moving with a velocity of 15 ms−1 is brought to rest by a player in 0.05 s. Calculate the impulse and the average force exerted by the player.
View Solution
Knowledge Check
A particle of mass 2kg moves with an initial velocity of →v=4ˆi+4ˆjms−1. A constant force of →F=20ˆjN is applied on the particle. Initially, the particle was at (0,0). The x-coordinates of the particle when its y-coordinates again becomes zero is given by
A1.2mB4.8mC6.0mD3.2mA body of mass 5 kg statrs from the origin with an initial velocity →u=(30ˆi+40ˆj)ms−1. If a constant force (−6ˆi−5ˆj)N acts on the body, the time in which they component of the velocity becomes zero is
A5sB20sC40sD80sA particl leaves the orgin with an lintial veloity →u=(3ˆI)ms&(−1) and a constant acceleration →a=(−1.0ˆi−05ˆj)ms−1.Itsvelocity vec vandpositvion→−−→r vec r when it reaches its maximum x-coosrdinate aer .
A→v=(−3^)ms−1B→v=(−22ˆj)ms−1C→r=(3ˆ−2ˆj)mD→r=(92ˆI−94ˆj)m
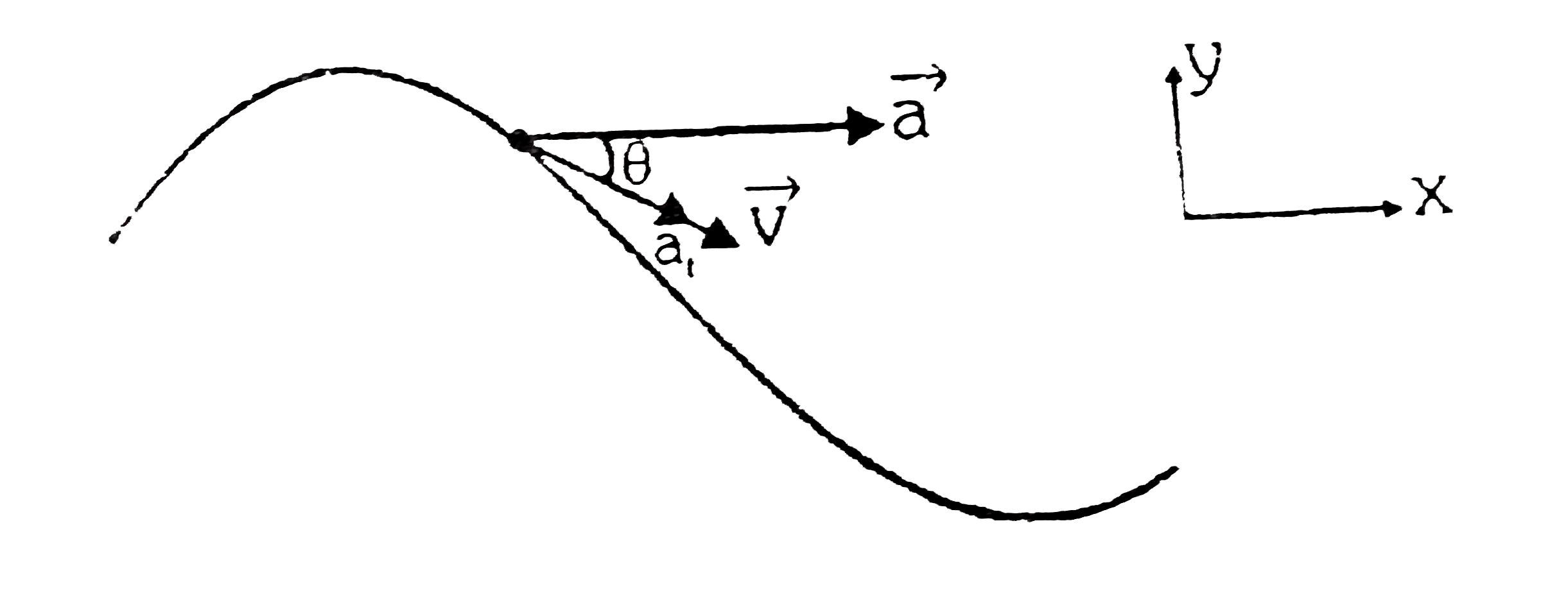
A particle moves with a tangential acceleration at=→a.ˆv where →a=(5ˆi)m/s2. If the speed of the particle is zero at x=0, then find v (in m/s) at x=4.9m.
View SolutionA machine carries a 4.0 kg package from an initial position of →di=(0.50m)ˆi+(0.75m)ˆj+(0.20m)ˆk at t=0 to a final position of →df=(7.50m)ˆi+(12.0m)ˆk at t=12 s. The constant force applied by the machine on the package is →F=(2.00N)ˆi+(4.00N)ˆj+(6.00N)ˆk. For that displacement, find (a) the work done on the package by the machine's force and (b) the average power of the machine's force on the package.
View SolutionA particle of mass 2kg is moving with velocity →v0=(2ˆi−3ˆj)m/s in free space. Find its velocity 3s after a constant force →F=(3ˆi+4ˆj)N starts acting on it.
View SolutionA ball falling with velocity →vi=(−0.65ˆi−0.35ˆj)ms−1 is subjected toa net impulse →I=(0.6ˆi+0.18ˆj)Ns. If the ball has a mass of 0.275 kg, calculate its velocity immediately following the impulse.
View SolutionA particle of mass 2kg moves with an initial velocity of (4ˆi+2ˆj)ms−1 on the x-y plane. A force →F=(2ˆi−8ˆj)N acts on the particle. The initial position of the particle is (2m,3m). Then for y=3m,
View Solution
