In a potentiometer experiment it is found that no current passes through the galvanometer when the terminals of the cell are connected across 0.52m of the potentiometer wire. If the cell is shunted by a resistance of 5Ω balance is obtained when the cell connected across0.4m of the wire. Find the internal resistance of the cell.
2Ω
2.5Ω
1Ω
1.5Ω
The correct Answer is:D
Let l1 be the balancing length without shunt and l2 be the balancing length with shunt across the wire then internal resistance of the cell can be written as follows:
r=R(l1l2−1)
=5(5240−1)=5×1240=1.5Ω
Hence option (d) is correct
Topper's Solved these Questions
- CURRENT ELECTRICITYBook:MODERN PUBLICATIONChapter:CURRENT ELECTRICITYExercise:Objective Type Questions (MULTIPLE CHOICE QUESTIONS) (B. MULTIPLE CHOICE QUESTION JEE (ADVANCED) FOR IIT ENTRANCE)
Explore 9 Videos
- CURRENT ELECTRICITYBook:MODERN PUBLICATIONChapter:CURRENT ELECTRICITYExercise:Objective Type Questions (MULTIPLE CHOICE QUESTIONS) (C. MULTIPLE CHOICE QUESTIONS WITH MORE THAN ONE CORRECT ANSWER)
Explore 18 Videos
- CURRENT ELECTRICITYBook:MODERN PUBLICATIONChapter:CURRENT ELECTRICITYExercise:Objective Type Questions (MULTIPLE CHOICE QUESTIONS) (B. MULTIPLE CHOICE QUESTION FOR COMPETITIVE EXAMINATIONS)
Explore 20 Videos
- ATOMS Book:MODERN PUBLICATIONChapter:ATOMS Exercise:Chapter Practice Test
Explore 16 Videos
- DUAL NATURE OF RADIATION AND MATTER Book:MODERN PUBLICATIONChapter:DUAL NATURE OF RADIATION AND MATTER Exercise:CHAPTER PRACTICE TEST
Explore 15 Videos
Similar Questions
In a ptentiometer experiment it is found that no current passes through the galvanometer when the terminals of a cell are connected across 509 cm of the potentiometer wire. When the cell is cnnected across 490 cm of the wire Find the internal resistance of the cell.
View SolutionIn a potentiometer experiment, no current passes through the galvanometer when the terminals of the cell are connected across 78 cm of the potentiometer wire. Now the cell is shunted by a resistance of 6Ω. A balance point is found across 26 cm of the wire. The internal resistance (in ohms) of the cell is ______
View Solution
Knowledge Check
In a potentiometer experiment, the galvanometer shows no deflection when a cell is connected across 60cm of the potentiometer wire. If the cell is shunted by a resistance of 6Ω, the balance is obtained across 50cm of the wire. The internal resistance of the cell is
A0.5ΩB0.6ΩC1.2ΩD1.5ΩFor a cell of e.m.f. 2 V , a balance is obtained for 50 cm of the potentiometer wire. If the cell is shunted by a 2Ω resistor and the balance is obtained across 40 cm of the wire, then the internal resistance of the cell is
A0.25ΩB0.50ΩC0.80ΩD1.00ΩIt is observed in a potentiometer experiment that no current passes through the galvanometer, when the terminals of the potentiometer, wire. On shunting the cell by a 2Ω resistance, the balancing length is reduced to half. The internal resistance of the cell is:-
A4ΩB2ΩC9ΩD18Ω
The reading on a high resistance voltmeter. When a cell is connected across it is 2.2V. When the terminals of the cell are connected to a resistance of 5Ω the voltmeter reading drop to 1.8V. Find the internal resistance of the cell.
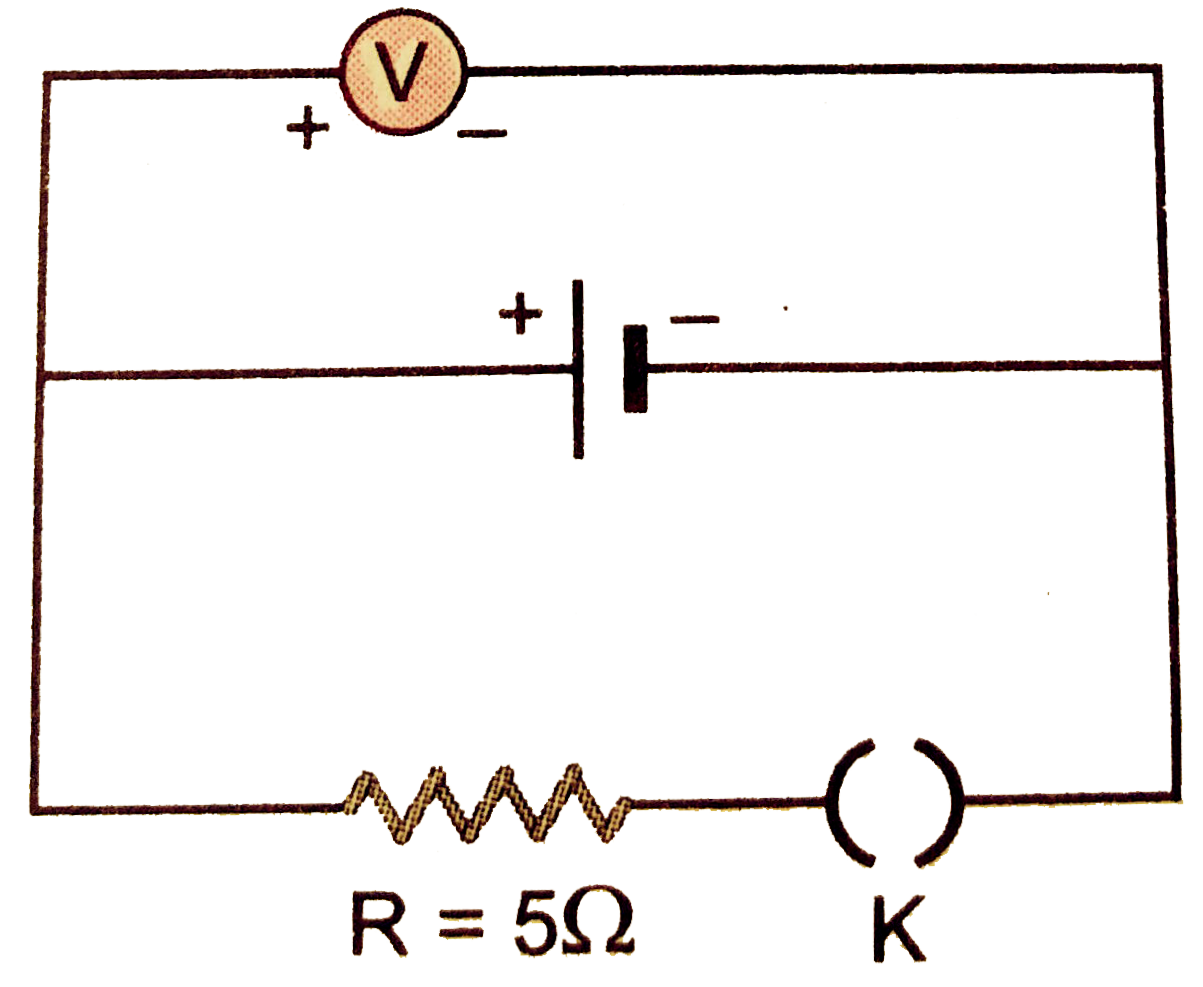
View SolutionThe reading on a high resistance voltmeter when a cell is connected across it is 3 V. When the terminals of the cell are also connected to a resistance of 4 Ω, then the voltmeter reading drops to 1.2 V. Find the internal resistance of the cell.
View SolutionA cell of e.m.f. 1.2 V is balanced by 150 cm of potentiometer wire. When the cell is shunted by a resistance of 4Ω, the balancing length is reduced by 30 cm. What is the internal resistance of the cell ?
View SolutionIn a potentiometer the balancing with a cell is at length of 220cm. On shunting the cell with a resistance of 3Ω balance length becomes 130cm. What is the internal resistance of this cell.
View SolutionThe e.m.f. of a standard cell balances across 150 cm length of a wire of potentiometer. When a resistance of 2Ω is connected as a shunt with the cell, the balance point is obtained at 100cm . The internal resistance of the cell is
View Solution