In a region of space a uniform magnetic field exist in positive z direction and there also exists a uniform electric field along positive y direction. A particle having charge + q and mass m is released from rest at the origin. The particle moves on a curve known as cycloid. If a wheel of radius R were to roll on the X axis, a fixed point on the circumference of the wheel would generate this cycloid. Find the radius R. It is given that strength of magnetic and electric fields are B0 and E0 respectively.
The correct Answer is:R=mE0qB20
To solve the problem, we need to analyze the motion of a charged particle in the presence of both electric and magnetic fields. The particle is subject to forces due to these fields, and we will derive the radius R of the cycloid generated by the motion of the particle.
1. Understanding the Forces:
- The particle with charge +q and mass m is released from rest at the origin.
- The electric field →E is directed along the positive y-axis, and the magnetic field →B is directed along the positive z-axis.
- The force due to the electric field is given by →FE=q→E.
- The force due to the magnetic field is given by →FB=q→v×→B, where →v is the velocity of the particle.
2. Setting Up the Equations of Motion:
- The total force acting on the particle is the sum of the electric and magnetic forces:
→F=→FE+→FB=q→E+q→v×→B
- In component form, this gives:
md→vdt=q→E+q→v×→B
3. Defining the Motion:
- Let the velocity of the particle be →v=vx^i+vy^j.
- The electric field →E=E0^j and the magnetic field →B=B0^k.
4. Writing the Force Components:
- The force in the x-direction is:
mdvxdt=qvyB0
- The force in the y-direction is:
mdvydt=qE0−qvxB0
5. Differentiating the Equations:
- Differentiate the equation for vy:
md2vydt2=−qB0dvxdt
- Substitute dvxdt from the first equation into this equation.
6. Solving the Differential Equations:
- By solving the differential equations, we find:
vy=E0B0sin(qB0tm)
- Integrating vy gives the position y:
y=mE0qB20(1−cos(qB0tm))
7. Finding the Maximum Value of y:
- The maximum value of y occurs when cos(qB0tm)=−1:
ymax=2mE0qB20
- This maximum height corresponds to 2R (the height of the cycloid).
8. Calculating the Radius R:
- Therefore, we can express the radius R as:
R=mE0qB20
Final Answer:
The radius R of the cycloid is given by:
R=mE0qB20
- The particle with charge +q and mass m is released from rest at the origin.
- The electric field →E is directed along the positive y-axis, and the magnetic field →B is directed along the positive z-axis.
- The force due to the electric field is given by →FE=q→E.
- The force due to the magnetic field is given by →FB=q→v×→B, where →v is the velocity of the particle.
- The total force acting on the particle is the sum of the electric and magnetic forces:
→F=→FE+→FB=q→E+q→v×→B
- In component form, this gives:
md→vdt=q→E+q→v×→B
- Let the velocity of the particle be →v=vx^i+vy^j.
- The electric field →E=E0^j and the magnetic field →B=B0^k.
- The force in the x-direction is:
mdvxdt=qvyB0
- The force in the y-direction is:
mdvydt=qE0−qvxB0
- Differentiate the equation for vy:
md2vydt2=−qB0dvxdt
- Substitute dvxdt from the first equation into this equation.
- By solving the differential equations, we find:
vy=E0B0sin(qB0tm)
- Integrating vy gives the position y:
y=mE0qB20(1−cos(qB0tm))
- The maximum value of y occurs when cos(qB0tm)=−1:
ymax=2mE0qB20
- This maximum height corresponds to 2R (the height of the cycloid).
- Therefore, we can express the radius R as:
R=mE0qB20
The radius R of the cycloid is given by:
R=mE0qB20
Topper's Solved these Questions
- MOTION OF CHARGE IN MAGNETIC FIELDBOOK - ARIHANTCHAPTER - MOTION OF CHARGE IN MAGNETIC FIELDEXERCISE - Level-2
13 Videos
- MAGNETIC EFFECT OF CURRENTBOOK - ARIHANTCHAPTER - MAGNETIC EFFECT OF CURRENTEXERCISE - Magnetic effect of Current
45 Videos
- NUCLEAR PHYSICSBOOK - ARIHANTCHAPTER - NUCLEAR PHYSICSEXERCISE - All Questions
54 Videos
Similar Questions
In a region of space a uniform magnetic field perpendicular to the plane of the page and directed towards reader exists. A particle has a trajectory as shown in fig. The particle is-
View SolutionElectric field E = – bx + a exists in a region parallel to the X direction (a and b are positive constants). A charge particle having charge q and mass m is released from the origin X = 0. Find the acceleration of the particle at the instant its speed becomes zero for the first time after release.
View Solution
Knowledge Check
An electron moving with a uniform velocity along the positive x-direction enters a magnetic field directed along the positive y-direction. The force on the electron is directed along
APositive y− directionBNegative y-directionCPositive z- directionDNegative z-directionA positively charged particle is released from rest in an uniform electric field. The electric potential energy of the charge
Aremains a constant because the electric field is uniform.Bincreases because the charge moves along the electric field.Cdecreases because the charge moves along the electric field.Ddecreases because the charge moves opposite to the electric field.A positively charged particle is released from rest in a uniform electric field. The electric potential energy of the charge.
Aremains a constant because the electric field is uniform.Bincrease because the charge moves along the electric field.Cdecreases because the charge moves along the electric field.Ddecreases because the charge moves opposite to the electric field.
Let there be a uniform electric field "E" existing along the positive X-direction.Assume electric potential to be zero at the origin.Potential at the point x=x0 is
View SolutionAn electron in moving along positive x-axis in the presence of uniform magnetic field along positive y-axis. What is the direction of the force acting on it ?
View SolutionA proton is placed in a uniform electric field directed along the positive x-axis . In which direction will it tend to move?
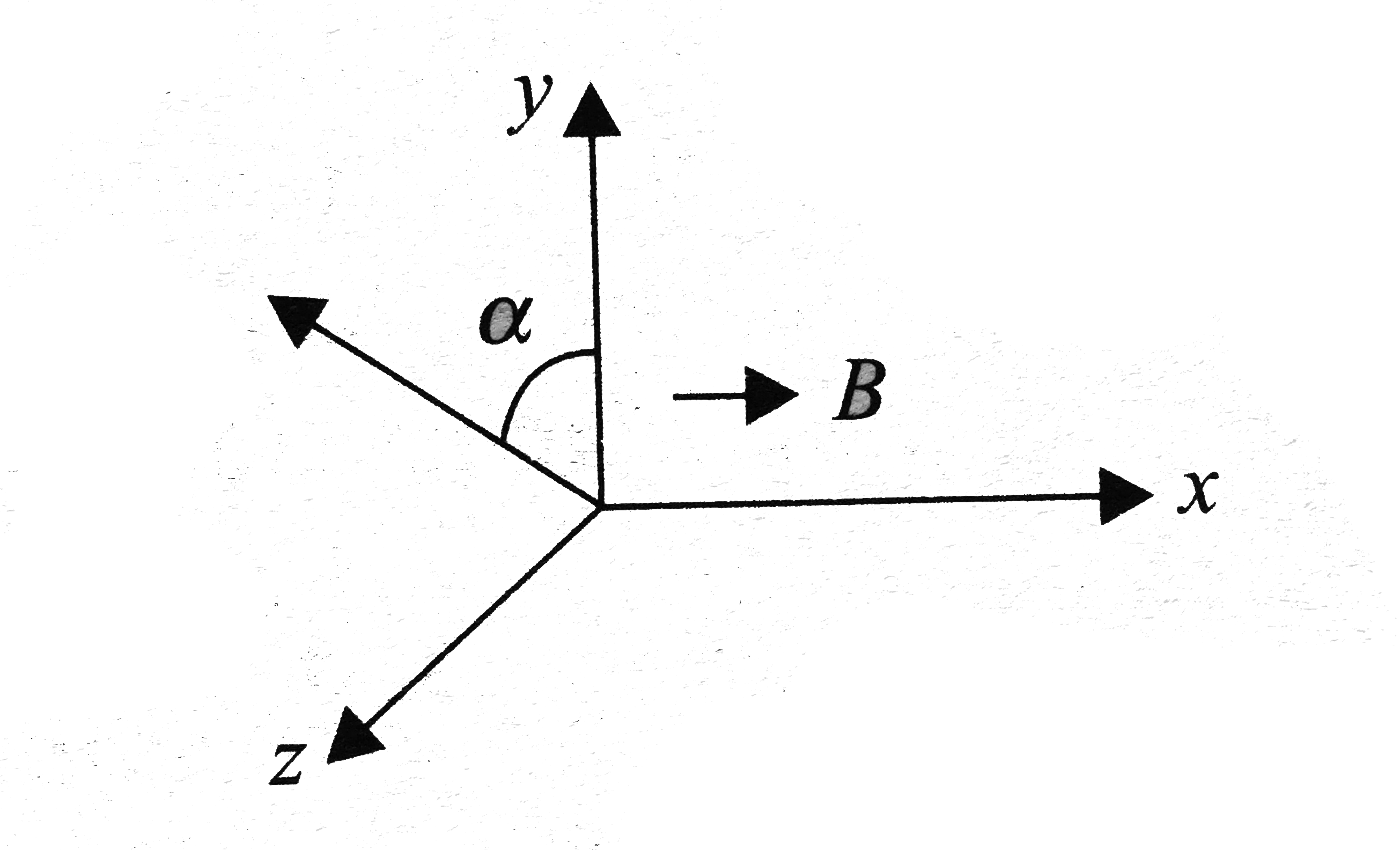
View SolutionIn a region of space, a uniform magnetic field B exists in the x-direction. An electron is fired from the origin with its initial velocity u making an angle α with the y direction in the y-z plane. In the subsequent motion of the electron,
View SolutionA charged particle begins to move from the origin in a region which has a uniform magnetic field in the x-direction and a uniform electric field in the y-direction. Its speed is v when it reaches the point (x, y, z). Then, v will depend
View Solution