In an orbital motion, the angular momentum vector is :
A
along the radius vector
B
parallel to the linear momentum
C
in the orbital plane
D
perpendicular to the orbital plane
Video Solution
Text Solution
The correct Answer is:D
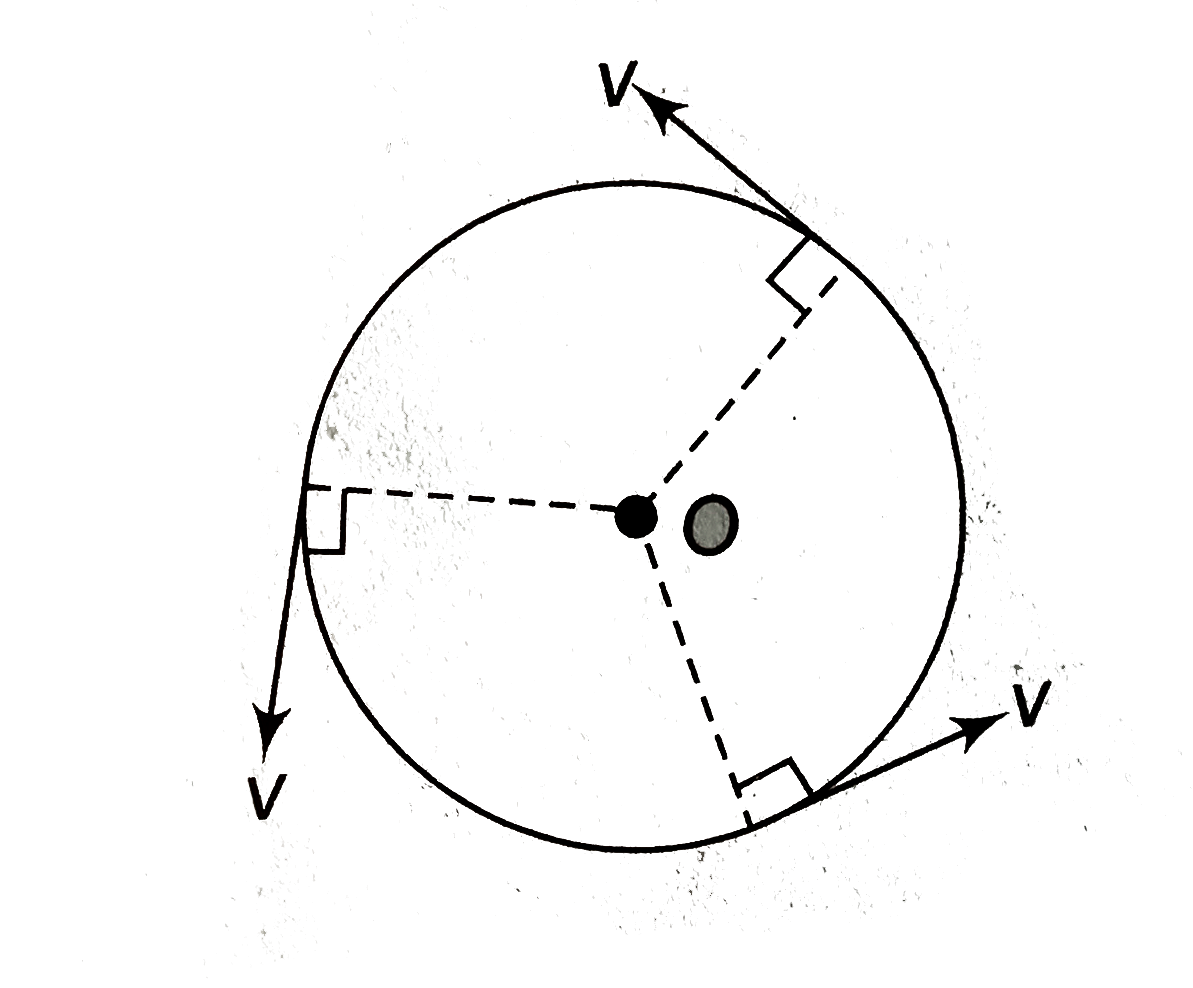
If a body is rotating about an axis, then the sum of the moments of the linear moment of all the particles about the given axis is called the angular momentum of the body about that axis.
J=Iω=mrv
Since direction of velocity is perpendicular to oribital plane and J∝v, therefore in an orbital motion the angular momentum vector is perpendicular to the orbital plane.
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - A2ZCHAPTER - MOCK TESTEXERCISE - Motion With Constant Acceleration15videos
MOCK TEST
- BOOK - A2ZCHAPTER - MOCK TESTEXERCISE - Motion With Constant Acceleration15videos
MOCK TEST
- BOOK - A2ZCHAPTER - KINETIC THEORY OF GASES AND THERMODYNAMICSEXERCISE - Chapter Test29videos
KINETIC THEORY OF GASES AND THERMODYNAMICS
- BOOK - A2ZCHAPTER - MOTION IN TWO DIMENSIONEXERCISE - Chapter Test29videos
MOTION IN TWO DIMENSION
Similar Questions
Knowledge Check
- Question 1 - Select One
Angular momentum is vector product of
Aradius vector and linear momentumBlinear momentum and angular velocityCmomentum of inertia and angular accelerationDlinear velocity and radius vector - Question 2 - Select One
In planetary motion, the angular Momentum conservation leads to the law of
AorbitsBareasCperiodsDconservation of kinetic energy - Question 3 - Select One
The orbital angular momentum of 3p electron is :-
A√3hB√6hCzeroD√2h2π
The orbital angular momentum of a p- electron is
View Solutionthe orbital angular momentum of 4f electron is
View SolutionThe magnitude of an orbital angular momentum vector of an electron is √6h2π into how many components will the vector split if an external field is applied to it ?
View SolutionThe orbital with zero orbital angular momentum is.
View SolutionThe orbital with zero orbital angular momentum is :
View Solution

