In Fig. , a rectangular block floats in a liquid . A block of mass m = 0.500 kg and density ρ=700kg/m3 floats face down in a liquid of density ρf=1200kg/m3 . What are the magnitude of the buoyant force on the block from the liquid , the weight of the liquid displaced by the block (liquid that would be in the space now occupied by the block ) , and the mass of that displaced liquid ? What fraction of the block's volume is submerged ? What fraction of its height is submerged ?

Because the block is floating , the magnitude of the (upward) buoyant force on it must match the magnitude of the (downward) gravitational force on it :
Fb=mg (14−22)
=(0.500kg)(9.8m/s2)=4.9N
Regardless of whether the block is floating , the magnitude of the buoyant force must equal the weight mfg of the liquid displaced by the block . Thus , we have mfg=Fb (14−23)
= 4.9 N
The mass of the displaced liquid is then
mfg=0.500 kg the same as the block .
To find the fraction of the block.s volume that is submerged , let us combine Eqs. 14-22 and 14-23 to write mfg=mg (14−24) and then relate the masses to the densities and volume using the general relation ρ=m/V . Here , we have the submerged volume Vf and the full block volume V . We also have the liquid.s density ρf and the block.s density ρ . Equation 14-24 then becomes
ρfVfg=ρVg.
or VfV=ρρf
=700kg/m31200kg/m3=0.58. (14−24a)
Thus , 58 % of the block.s volume is submerged .
To find the submerged height h , we note that the submerged volume Vf and the area of the bottom face are related by Vf=Ah.
Similarly , the full volume and the full height H are related by V=AH.
Substituting these expressions into Eq. 14-24 a leads to hH=ρρf=0.58.
Thus , 58 % of the block.s height is submerged .
Topper's Solved these Questions
- BOOK - RESNICK AND HALLIDAYCHAPTER - FLUIDSEXERCISE - SAMPLE PROBLEM 14.071videos
FLUIDS
- BOOK - RESNICK AND HALLIDAYCHAPTER - FLUIDSEXERCISE - SAMPLE PROBLEM 14.081videos
FLUIDS
- BOOK - RESNICK AND HALLIDAYCHAPTER - FLUIDSEXERCISE - SAMPLE PROBLEM 14.051videos
FLUIDS
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTROMAGNETIC WAVES EXERCISE - PRACTICE QUESTIONS (Integer Type)3videos
ELECTROMAGNETIC WAVES
- BOOK - RESNICK AND HALLIDAYCHAPTER - FORCE AND MOTION - I EXERCISE - PRACTICE QUESTIONS (Integer Type)3videos
FORCE AND MOTION - I
Similar Questions
Knowledge Check
- Question 1 - Select One
A body floats in a liquid if the buoyant force is
AzeroBgreater than its weightCless than its weightDequal to its weight - Question 2 - Select One
A body floats in a liquid if the buoyant force is
AzeroBgreater than its weightCless than its weightDequal to its weight - Question 3 - Select One
What is the pressure energy of a liquid of mass m and density ρ?
APmρBPρCmρDPmρ
A body of mass 2.0 kg and density 8000 kgm3 is completely dipped in a liquid of density 800 kgm3. Find the force of buoyancy on it.
View SolutionIn Fig , a block of density ρ=800kg/m3 floats face down in a fluid of density ρf=1200kg/m3 . The block has height H = 6.0 m
(a) By what depth h is the block submerged ?
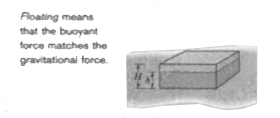
(b) If the block is held fully submerged and then released , what is the magnitude of its acceleration ?View SolutionThe speed of sound in a liquid of density 2×103kg/m3 is 1000 m/s. Determine the bulk modulus of elasticity of the liquid.
View SolutionA body will float in a liquid if weight of the liquid displaced by the body is ________or_______the weight of the body.
View SolutionA block of mass 5 kg and volume 0.05m3 floats in a liquid of density 140kg m−3 .
Volume of block above the surface of liquid isView Solution

