Figure shows a vertical wall of horizontal length L = 5.0 m and depth H = 3.0 m that is fully submerged in water , with its top surface of the water . What is the force on the wall due to the water pressure ?

Let y represent the depth below the top water surface . Then the gauge pressure is
pg=ρgy (14−25)
Note that because the expression contains a variable (namely , depth y) , we cannot use p = F/A to find the force on the wall because there is no one value of pressure . However , if we pick a small area dA on the wall Fig , we can relate the pressure p and force dF on the wall by
pg=dFdA (14−26)

(We use the "d" notation to indicate that we are dealing with tiny portions and differential amounts.) Equation 14-26 tells us that the force on dA is
dF=pgdA (14−27)
For area dA, we could choose a small square at some depth y . However , note that the variable in Eq 14-25 is y and not x . Thus , the pressure at a given depth y is the same at any horizontal position along the wall . So , we can choose dA to be the area of a strip of length L and tiny height dy . That is ,
dA = Ldy (14−28)
Then the force on that strip is
dF=pgLdy,
or , with Eq. 14-25 ,
dF=ρgyLdy (14−29)
To find the total force on the wall , we need to add the forces on all the horizontal strips from top to bottom of the wall . Of course , because each strip has the tiny height dy , there are a huge number of strips along the wall and adding up their forces would take "for ever" (much longer than our patience) . However , we can add them via integration rather quickly . Integrating both sides of Eq. 14-29 , we find
∫dF=∫ρgyLdy (14−30)
We want to include all the strips between y = 0 (at the top of the wall) and y = 3. 0 m (at the bottom of the wall) . To do so , we use those values as the limits to the integral on the right side of Eq. 14-30 . The integration on the left side yields the total force F we seek . We find
F=ρgL∫3.0m0ydy
=ρgL12y2∣∣∣3.0m0
=(1000kg/m3)(9.8m/s2)(5.0m)12(3.0m)2
2.21×105N.
Topper's Solved these Questions
- FLUIDSBOOK - RESNICK AND HALLIDAYCHAPTER - FLUIDSEXERCISE - SAMPLE PROBLEM 14.08
1 Videos
- FLUIDSBOOK - RESNICK AND HALLIDAYCHAPTER - FLUIDSEXERCISE - SAMPLE PROBLEM 14.09
1 Videos
- FLUIDSBOOK - RESNICK AND HALLIDAYCHAPTER - FLUIDSEXERCISE - SAMPLE PROBLEM 14.06
1 Videos
- ELECTROMAGNETIC WAVES BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTROMAGNETIC WAVES EXERCISE - PRACTICE QUESTIONS (Integer Type)
3 Videos
- FORCE AND MOTION - I BOOK - RESNICK AND HALLIDAYCHAPTER - FORCE AND MOTION - I EXERCISE - PRACTICE QUESTIONS (Integer Type)
3 Videos
Similar Questions
A flotation device is in the shape of a right cylinder, with a height of 0.650 m and a face area of 4.00m2 on top and bottom, and its density is 0.300 times that of fresh water. It is initially held fully submerged in fresh water, with its top face at the water surface. Then it is allowed to ascend gradually until it begins to float. How much work does the buoyant force do on the device during the ascent?
View SolutionA glass prism has its principal section in form of an equilateral triangle of side length l. The length of the prism is L (see fig.). The prism, with its base horizontal, is supported by a vertical spring of force constant k. Half the slant surface of the prism is submerged in water. Surface tension of water is T and contact angle between water and glass is 0∘. Density of glass is d and that of water is ρ(<d). Calculate the extension in the spring in this position of equilibrium.
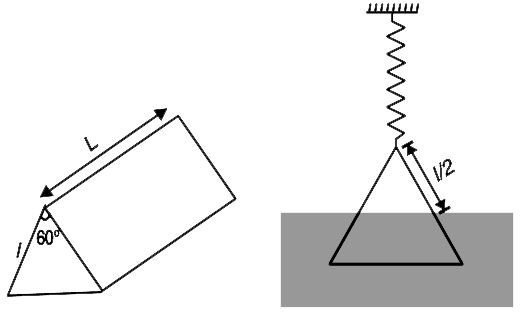
View Solution
Knowledge Check
A tank with vertical walls is mounted so that its base is at a height H above the horizontal ground. The tak is filled with water to a depth h. A hole is puched in the side wall of the tank at a depth x below the water surface. To have maximum range of the emerging stream, the value of x is
AH+h4BH+h2CH+h3D3(H+h)4A tank with length 10 m, breadth 8 m and depth 6 m is filled with water upto top . If G=10m/s2. And density of water =1000kg/m3, then the thrust on the bottom is:
A(6×1000×10×80)NB(3×1000×10×48) NC(3×1000×10×60) ND(3×100×10×80) NFigure shows a large closed cylindrical tank containing water. Initially, the air trapped above the water surface has a height h0 and pressure 2p0 where rh0 is the atmospheric pressure. There is a hole in the wall of the tank at a depth h1 below the top from which water comes out. A long vertical tube is connected as shown.
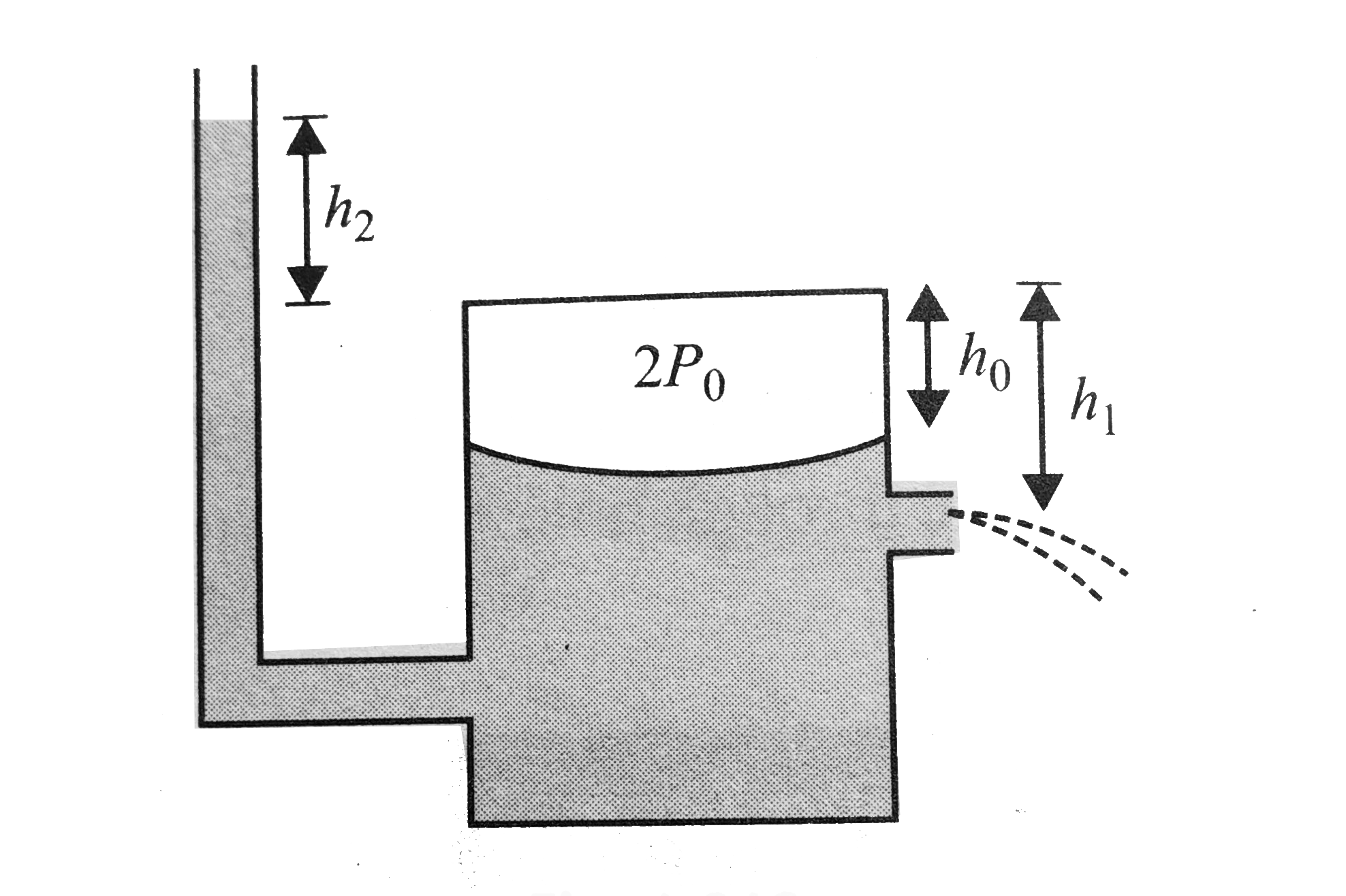
Find the height of the water in the long tube above the top when the water stops coming out of the hole.A−2h0Bh0Ch2D−h1
A ladder is resting against a vertical wall and its bottom is 2·5 m away from the wall. If it slips 0.8 m sown the wall, then its bottom will move away from the wall by 1.4 m. What is the length of the. ladder ?
View SolutionThe pressure at the bottom of a lake due to water is 4.9×106N/m2. What is the depth of the lake?
View SolutionWater stands at a depth H in a tank whose side walls are vertical. A hole is made on one of the walls at a depth h below the water surface. Find at what distance from the foot of the wall does the emerging stream of water strike the floor and for what value of h this range is maximum ? What is the maximum possible range ?
View SolutionA uniform capillary tube of length l and inner radius r with its upper end sealed is submerged vertically into water. The outside pressure is P0 and surface tension of water is T. What will be the value of c, when water levels inside and outside capillary coincide, length of capillary submerged into water is given by 2l(2+Pr0cT).
View SolutionA uniform wooden bar of length l and mass m hinged on a vertical wall of a containing water, at one end.

3/5th part of the bar is submerged in water. Find the ratio of densities of the liquid and the bar.
View Solution
