In Fig. a three-side frame is pivoted at AC and hangs vertically. Its sides are each of the same length and have a linear density of 0.10kgm−1. A current of 10.0 A is sent through the frame, which is in a uniform magnetic field of 10 mT directed upward. Through what angle will the frame be deflected?
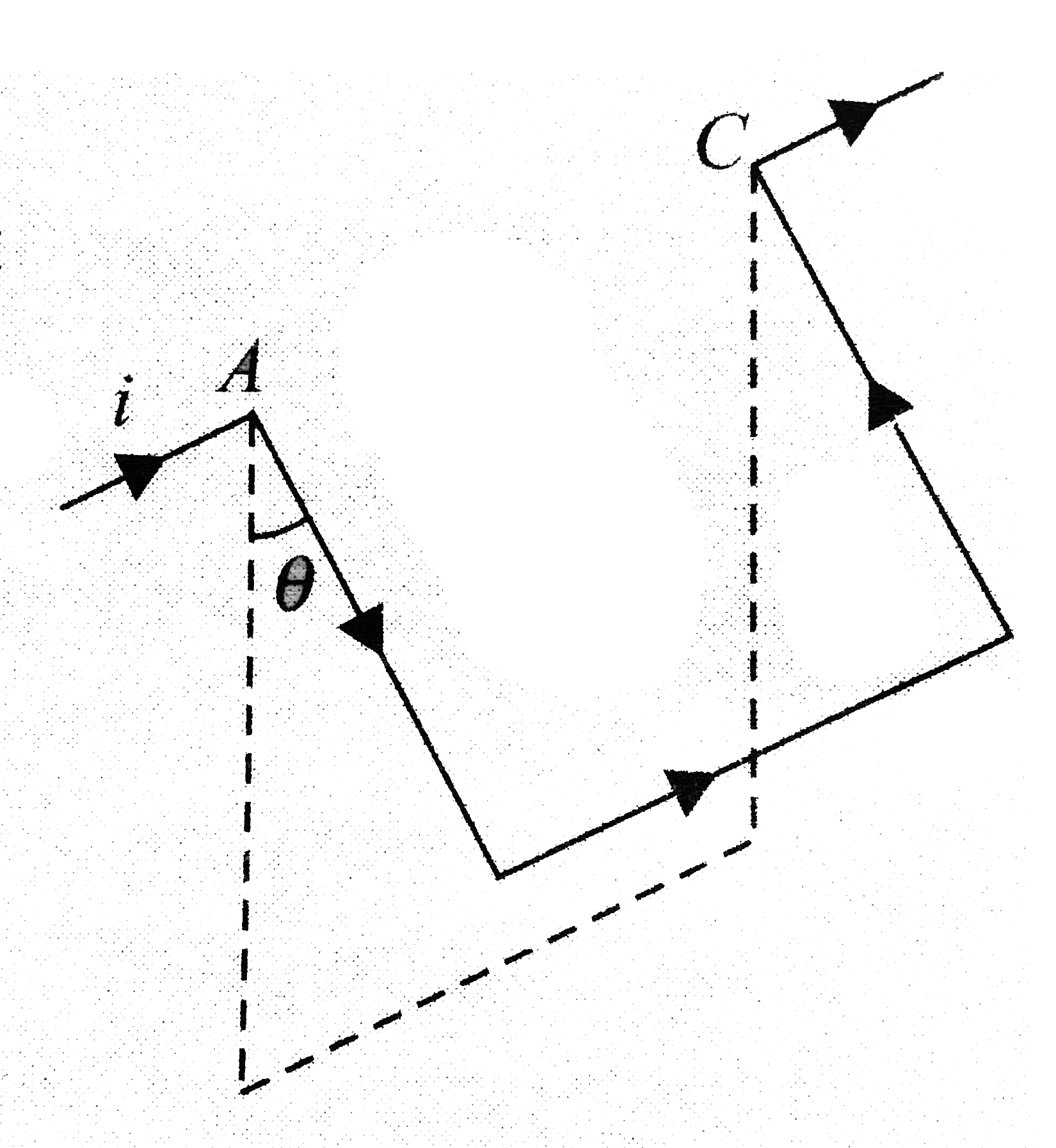
Refracting to the figure in question, we note that the magnetic forces on the slanting sides are parallel to AC and therefore, do not produce any torque about the axis AC. The magnetic force on the horizontal side of the frame is
Fmag=iL×B...(i)
This force has magnitude Fmag=iLBsin90∘=iLB and points horizontally to the right on this plane of this figure, perpendicular to L and B. The magnetic torque about AC has magnitude
Tm=iLB(Lcosθ)=iL2Bcosθ....(ii)
and tends to increase the angle θ. The gravitational torque about
the axis AC is
Tg=−[(λL)g(Lsinθ)+2(λL)]
g(12Lsinθ).....(iii)
where the minus sign indicates that gravity tends to turn the frame in the direction of decreasing θ. Let λ denote the mass per unit length of the wire. In equilibrium, the torque evaluted in equations (ii) and (iii) must cancel, which implies that tanθ=(Bi)/(2λg).

Subtituting the data, we obtain θ=tan−1(Bi2λg).
Topper's Solved these Questions
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Exercise1.3
14 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Exercises Subjective
10 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Exercise 1.1
22 Videos
- INDUCTANCEBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - INDUCTANCEEXERCISE - Concept Based
8 Videos
- MISCELLANEOUS VOLUME 3BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 3EXERCISE - True and False
3 Videos
Similar Questions
A current of 10 ampere is flowing in a wire of length 1.5m. A force of 15N acts on it when it is placed in a uniform magnetic field of 2 tesla. The angle between the magnetic field and the direction of the current is
View SolutionA coil in the shape of an equilateral triangle of side 0.01m is suspended from the vertex such that it is hanging in a vertical plane between pole pieces of a permanent magnet producing a horizontal magnetic field of 4×10−2 T. If a current of 1A is passing through it and the magnetic field is parallel the magnetic field is parallel to its plane, then the torque acting on the coil is
View Solution
Knowledge Check
The magnetic force per unit length on a wire carrying a current of 10 A and making an angle of 45∘ with the direction of a uniform magnetic field of 0.20 T is
A2√2Nm−1B2√2Nm−1C√22Nm−1D4√2Nm−1A current of 10 ampere is flowing in a wire of length 1.5m. A force of 15N acts on it when it is placed in a uniform magnetic field of 2 tesla. The angle between the magnetic field and the direction of the current is
A30∘B45∘C60∘D90∘A metallic wire having length of 2 m and weight of 4×10−3 N is found to remain at rest in a uniform and transverse magnetic field of 2×10−4T. Current flowing through the wire is :
A10AB5AC2AD1A
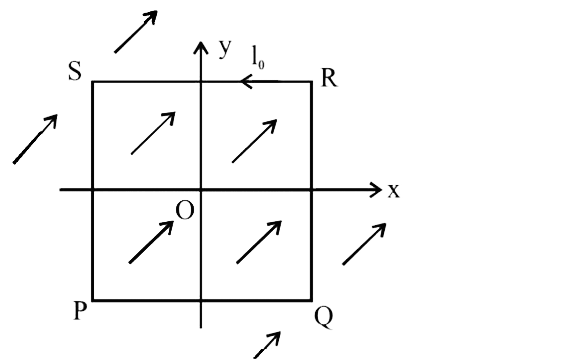
A uniform constant magnetic field B is directed at an angle of 45∘ to the xaξs in the xy- plane . PQRS is a rigid, square wire frame carrying a steady current I0, with its centre at the origin O. At time t=0, the frame is at rest in the position as shown in figure , with its sides parallel to the xandy axis. Each side of the frame is of mass M and length L.
(a) What is the torque τ about O acting on the frame due to the magnetic field?
(b) Find the angle by which the frame rotates under the action of this torque in a short interval of time Δt, and the axis about this rotation occurs .(Δtissoshorttˆanyvariation∈the→rquedur∈gthis∫ervalmaybe¬≤cted.)Given:themomentof∫eriaoftheameaboutanaξsthroughitscentreperpendica––r→itsaboutanaξsthroughitscentreperpendica––r→itspla≠is(4)/(3) ML^(2).
View SolutionA coil in the shape of an equilateral triangle of side 0.01 m is suspended from the vertex such that is hanging in a vertical plane between pole pieces of a permanent magnet producing a horizontal magnetic field of (4×10−2T).If a current of 1A is passing through it and the magnetic field is parallel to its field,then the torque acting on the coil is
View SolutionFigure shows a conducting frame having battery and a resistance on which a movable conductor of length 0.5 m can slide, The whole arrangement is placed in a uniform magnetic field of B =0.4 T directed perpendicular and into the plane of frame. Initially the circuit is open. When the key is inserted, the conductor begins to move. It is found that a force 0.5 N has to be applied on the conductor to the left to keep it moving at constant speed to the right.
Current flowing in the conductor is:

View SolutionA charge having q/m equal to 108 c/kg and with velocity 3×105 m/s enters into a uniform magnetic field B = 0.3 tesla at an angle 30∘ with direction of field. Then radius of curvature will be:
View SolutionA rectangular coil of 100 turns has length 5 cm and width 4 cm. it is placed with its plane parallel to a uniform magnetic field and a current of 2 A is sent through the coil. Find the magnitude of the magnetic field B, if the torque acting on the coil is 0.2Nm−1.
View Solution
