In Fig. initialy the system is at rest .Find out minimum value of Ffor which sliding start between the two blocks GivenmA=10kg and mB=20kg
At just sliding condition limiting friction is acting
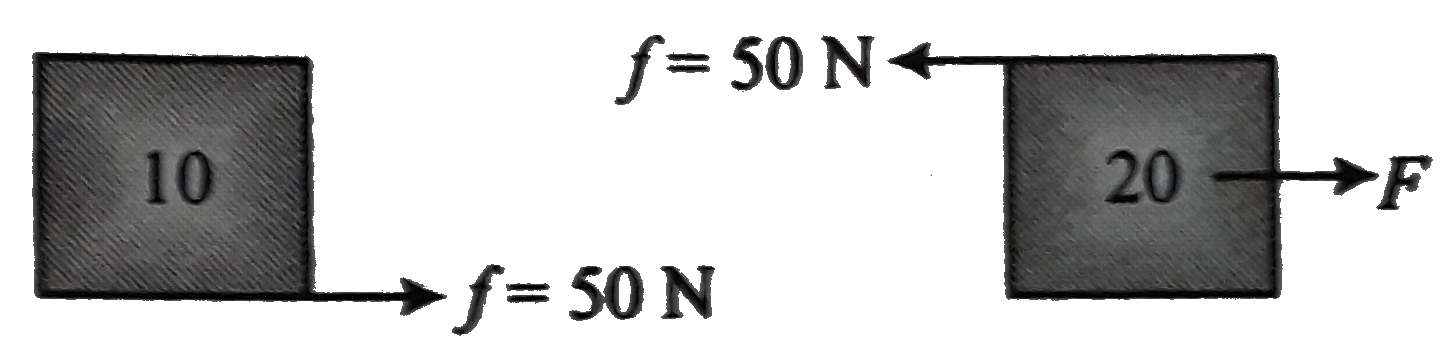
F−50=20a….(i)
f=10a ….(ii)
50=10a
∴a=5ms−2
Hence, F=50+20×5=150N
∴Fmax=150N
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Exercise 7.327videos
NEWTON'S LAWS OF MOTION 2
- BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Subjective21videos
NEWTON'S LAWS OF MOTION 2
- BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Exercise 7.125videos
NEWTON'S LAWS OF MOTION 2
- BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Integer5videos
NEWTON'S LAWS OF MOTION 1
- BOOK - CENGAGE PHYSICSCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - INTEGER_TYPE2videos
PROPERTIES OF SOLIDS AND FLUIDS
Similar Questions
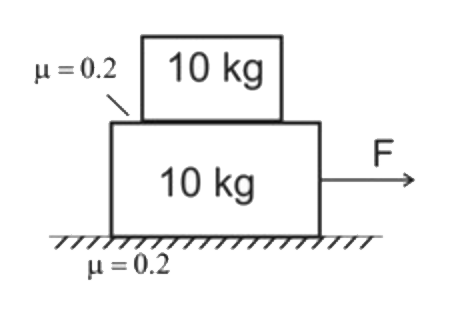
Initially the system is at rest. find out minimum value of F for which sliding starts between the two blocks.
View SolutionInitially, both the blocks are at rest on horizontal surface as shown in the figure. Find the minimum value of force F in (N) so that sliding starts between the blocks (g=10ms−2)
View Solution
Knowledge Check
- Question 1 - Select One
Find the minimum value of F for which 5 kg being to slide if the coefficient of friction between the blocks is changed to 0.5 :A10 NB30 NC45 NDno value exist - Question 2 - Select One
The coefficient of friction between block A of mass m1=5kg and block B of mass m2=10kg is μ2=0.5 . There is no friction froce between block B and fixed horizontal surface. A force of 300N acts on block B in horizontal direction and a horizontal force of magnitude F acts on block A as shown , both towards right. Initially there is no relative motion between the blocks. The minimum value of F such that relative motion starts between A and B is :
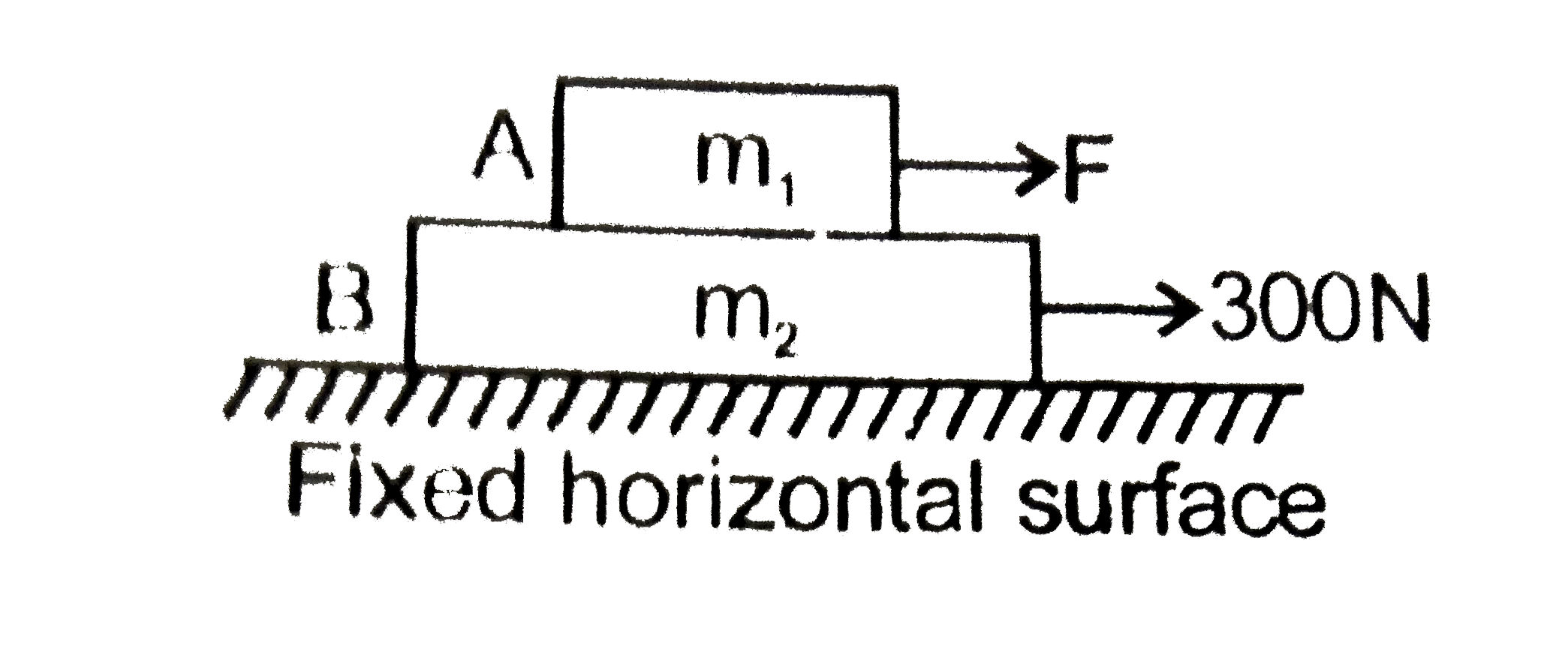A200NB187.5NC150ND0
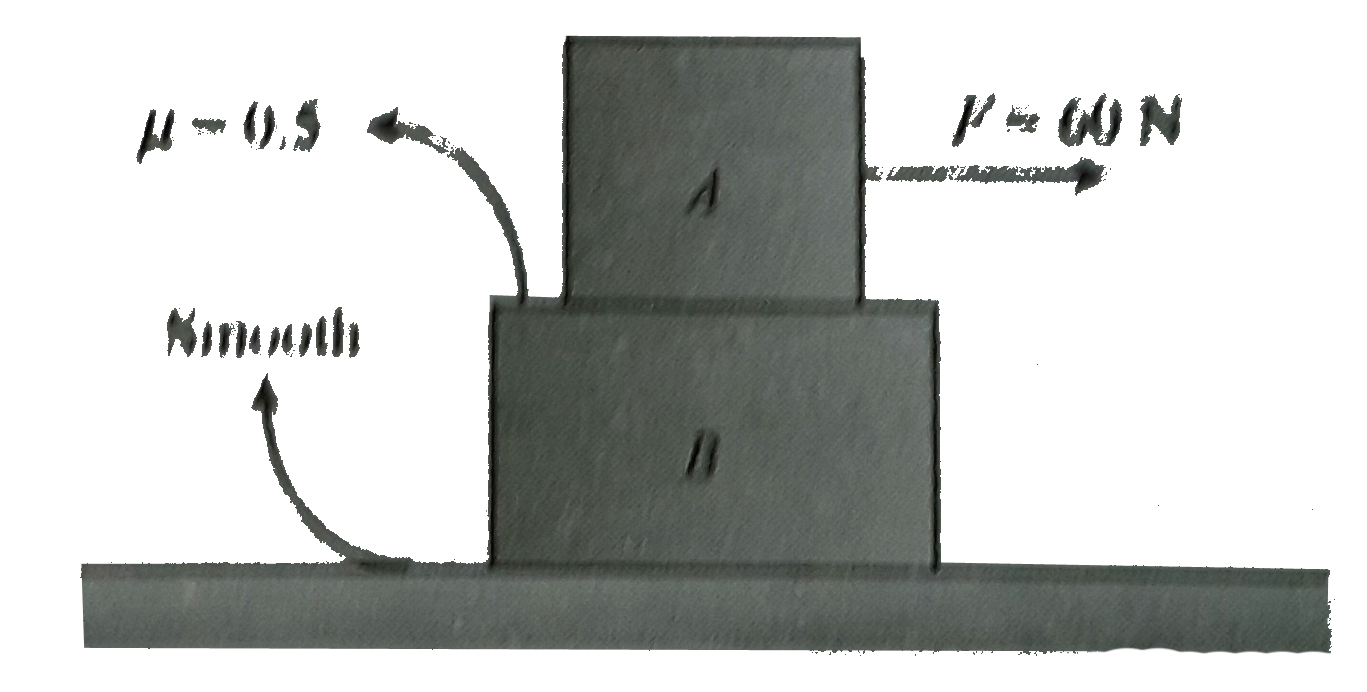
Find the acceleration of the two blocks The system initally at rest and the friction coefficient are as shown in fig 7.140? Also find maximum F for which two blocks will move togather Given mA=10kgandmB=20kg
View SolutionIn the arrangement shown in figure pulley P can move whereas other two pulleys are fixed. All of them are light. String is light and inextensible. The coefficient of friction between 2 kg and 3 kg block is μ=0.75 and that between 3 kg block and the table is μ=0.5. The system is released from rest
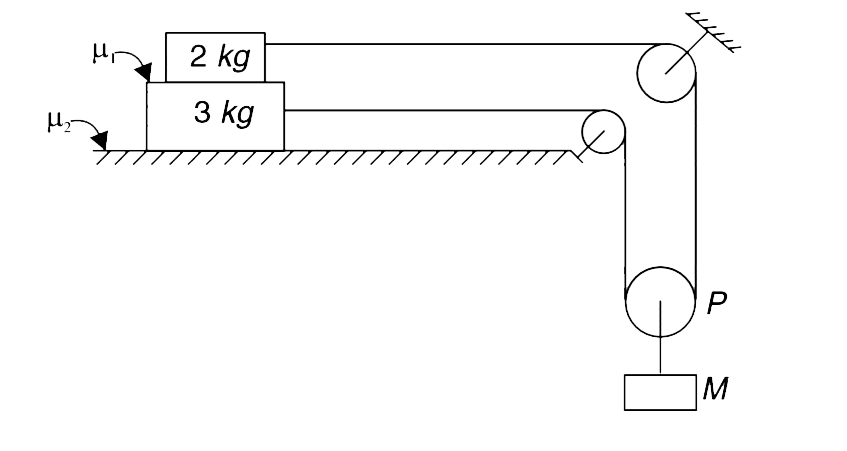
(i) Find maximum value of mass M, so that the system does not move. Find friction force between 2 kg and 3 kg blocks in this case.
(ii) If M = 4 kg, find the tension in the string attached to 2 kg block.
(iii) If M = 4 kg and μ1=0.9, find friction force between the two blocks, and acceleration of M.
(iv) Find acceleration of M if m1=0.75,m2=−0.9 and M = 4 kg.View SolutionA plank of mass 10kg rests on a smooth horizontal surface. Two blocks A and B of masses mA=2kgandmB=1kg lies at a distance of 3m on the plank as shown in the distance of 3m on the plank as shown in the figure. The friction coefficient between the blocks and plank are μA=0.3andμB=0.1. Now a force F = 15 N is applied to the plank in horizontal direction. Find the time after which block A collides with B.
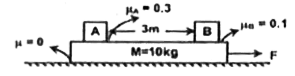View SolutionA 4 kg block ls placed on top of a long .12 kg block, which is accelerating along a smooth horizontal table at a=5.2m/s2 under application of an external constant force. Let minimum, coefficient of friction between the two blocks which will prevent the 4 kg block from sliding is μ, and coefficient of friction between blocks ls only half of this minimum value. of, (i.e., μ/2). Find the amount of heat (in joules) generated due to sliding between the two blocks during the time in which. 12 kg block moves 10 m starting from rest
View SolutionWhat should be minimum value of F so that 2 kg slides on ground but 1kg does not slide on it? [g=10msec2]
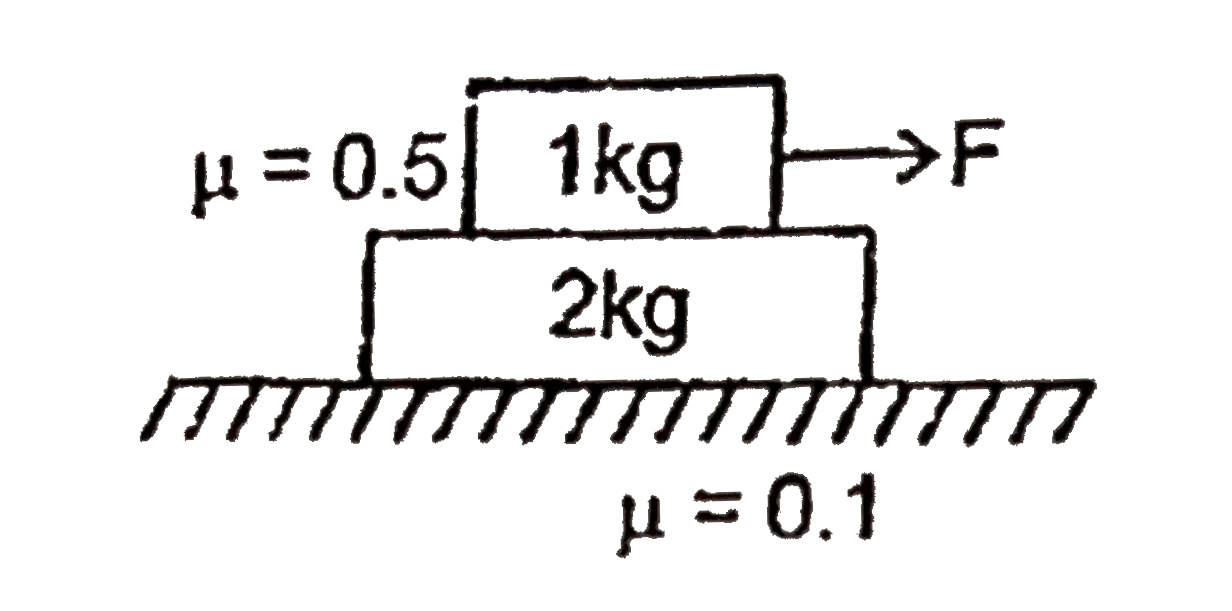View SolutionA constant force F=20N acts on a block of mass 2kg which is connected to two blocks of masses m1=1.0kg and m2=2kg as shown in Calculate the accelerations produced in ball the three blocks Assume pulleys are frictionless and weightless
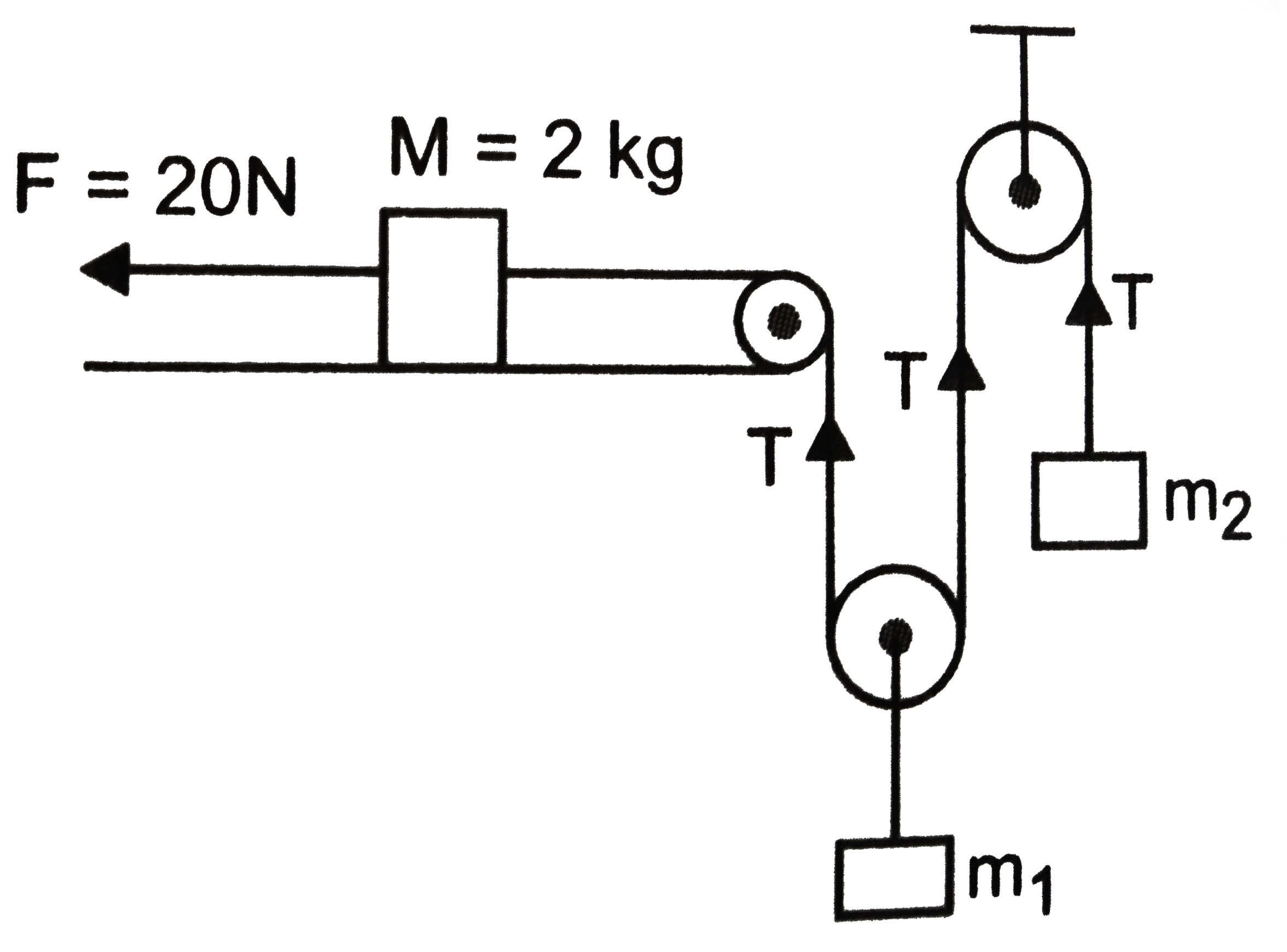. View Solution