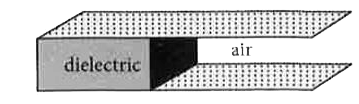
Initially the space between the plates of the capacitor is filled with air, and the field strength in the gap is equal to E0. Then half the gap is filled with uniform isotropic dielectric with permittivity ε as shown in Fig. Find the moduli of the introduction of the dielectric
(a) deos not change the voltage across the plates,
(b) leaves the charges at the plates constant.

(a) We have D1=D2, or epsilon E2=E1
Also, E1d2+E2d2=E0d or, E1+E2=2E0
Hence, E2=2E0ε+1 and E1=2εE0ε+1 and D1=D2=2εε0E0ε+1
(b) D1=D2, or εE2=E1=σε0=E0
Thus, E1=E0,E2=E0ε and D1=D2=ε0E0
Topper's Solved these Questions
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Electric Capacitance - Energy Of An Electric Field46videos
ELECTRODYNAMICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Electric Current72videos
ELECTRODYNAMICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Motion Of Charged Particle In Magnetic Field36videos
ELECTRODYNAMICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRICITY AND MAGNETISMEXERCISE - All Questions6videos
ELECTRICITY AND MAGNETISM
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTROMAGNETISMEXERCISE - All Questions24videos
ELECTROMAGNETISM
Similar Questions
Knowledge Check
- Question 1 - Select One or More
In figure half of the space between the plates of a parallel plate capacitor is filled with dielectric material of constant K. Then which of the plots are possible?
 A
A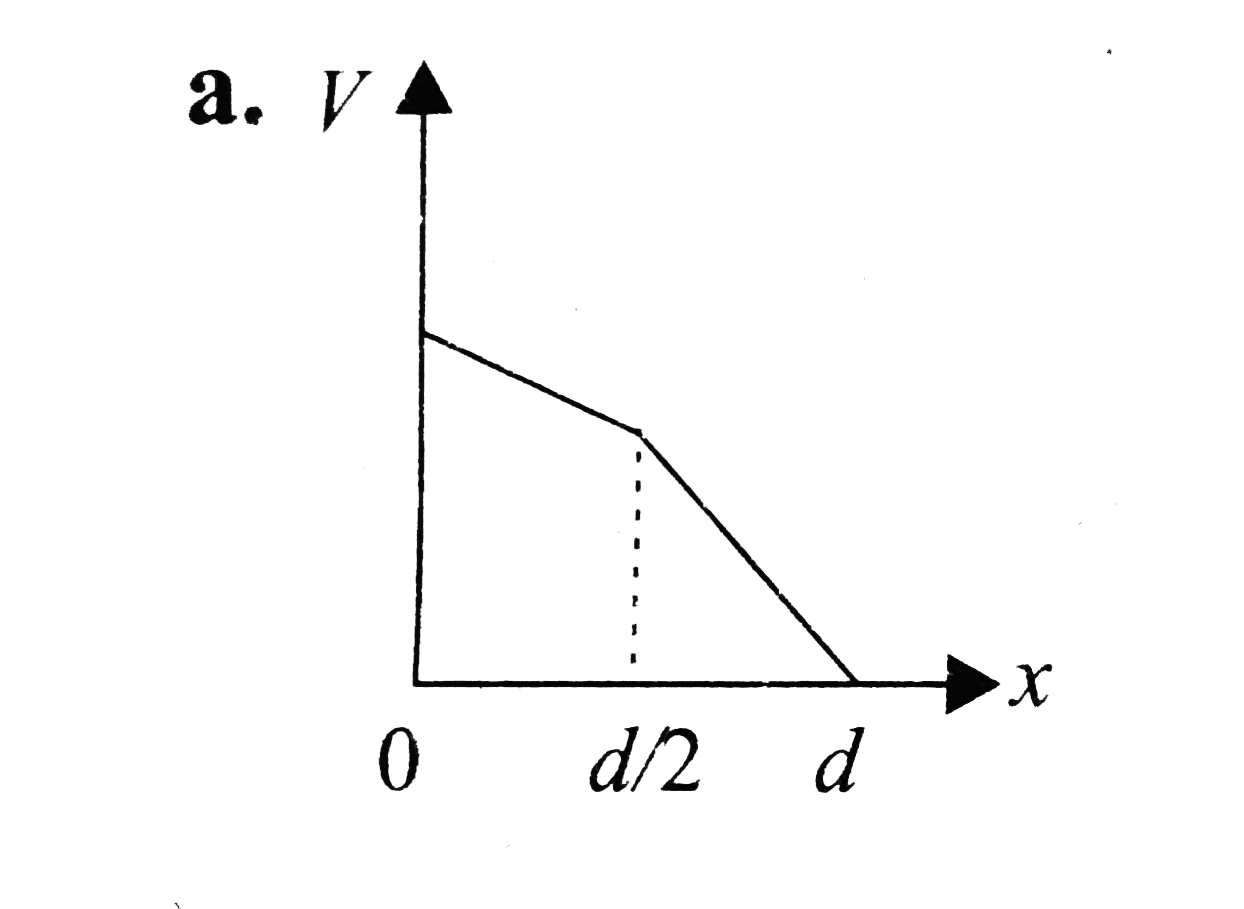 B
B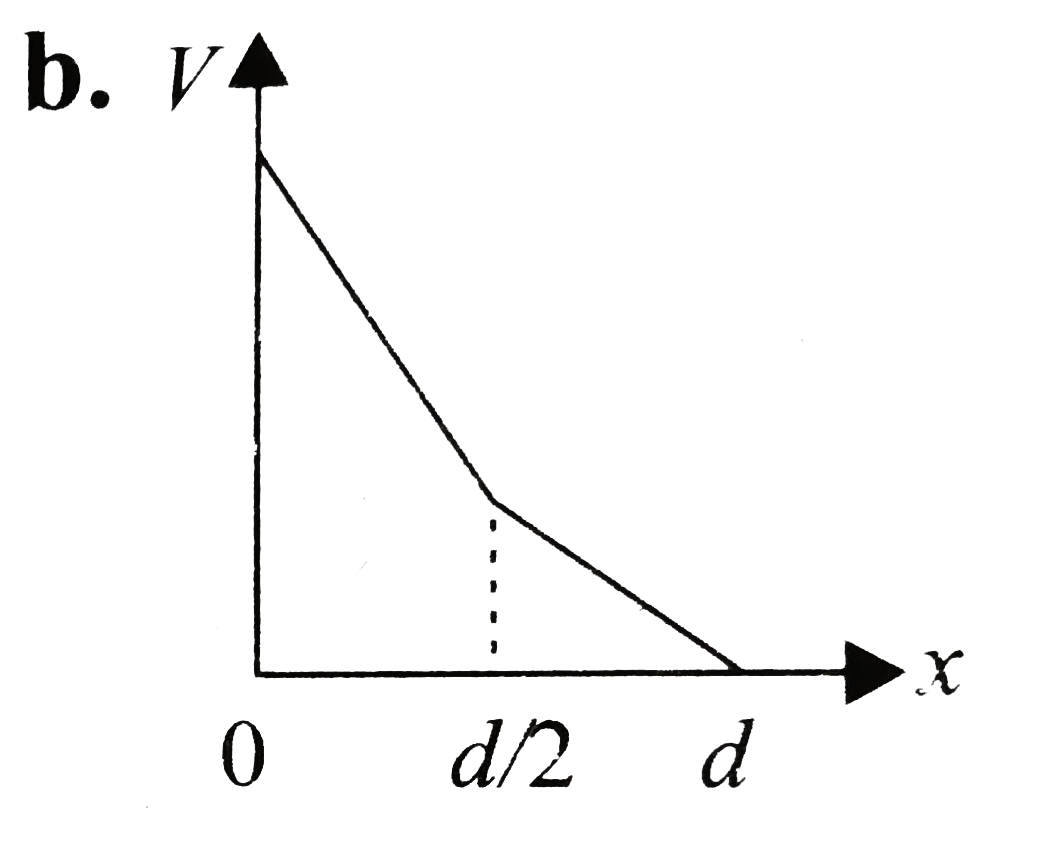 C
C D
D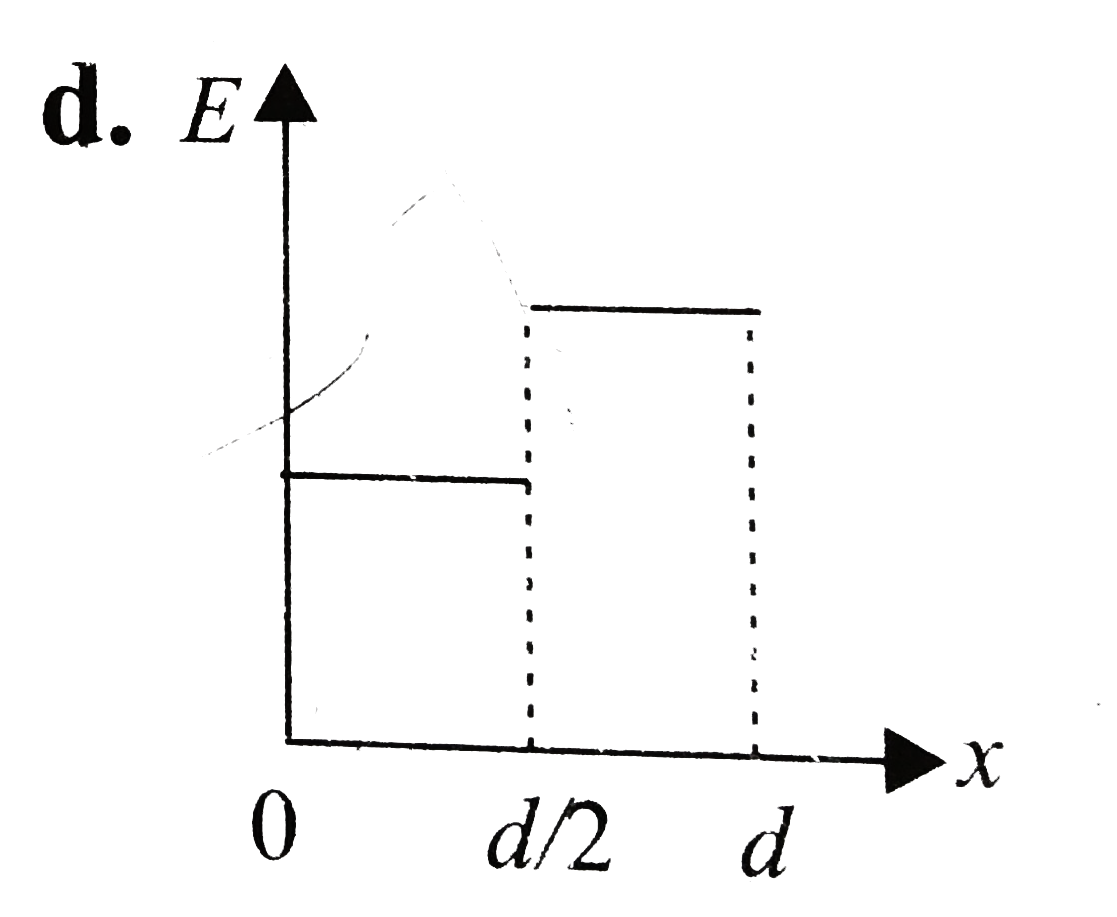
- Question 2 - Select One
If the gap between the plates of a parallel plate capacitor is filled with medium of dielectric constant k=2 , then the field between them
AIncreases by a factor 2BIncreases by a factor √2CDecreased by a factor √2DDecreased by a factor 1/2 - Question 3 - Select One
Half of the space between parallel plate capacitor is filled with a medium of dielectric constant K parallel to the plates . if initially the capacity is C, then the new capacity will be :
A2KC(1+K)BC(K+1)/2CCK/(1+K)DKC
A parallel-plate capacitor is filled with a dielectric up to one-half of the distance between the plates.The manner in which the potential between the plates varies is illustrated in the figure. Which half (1or2) of the space between the plates is filled with the dielectric and what will be the distribution of the potential after the dielectric is taken out of the capacitor provided that (a) the charges on the plates are conserved or (b) the potential difference across the capacitor is conserved?
View SolutionConsider a disconnected plate capacitor of capacity 10μF with air filled in the gap between the plates. Now one-half of the space between the plates is filled with a dielectric of dielectric constant 4 as shown in .The capacity of the capacitor changes to
 .View Solution
.View SolutionConsider a parallel plate capacitor of 10μF (micro-farad) with air filled in the gap between the plates. Now one half of the space between the plates is filled with a dielectric of dielectric constant 4, as shown in the figure. The capacity of the capacitor charges to
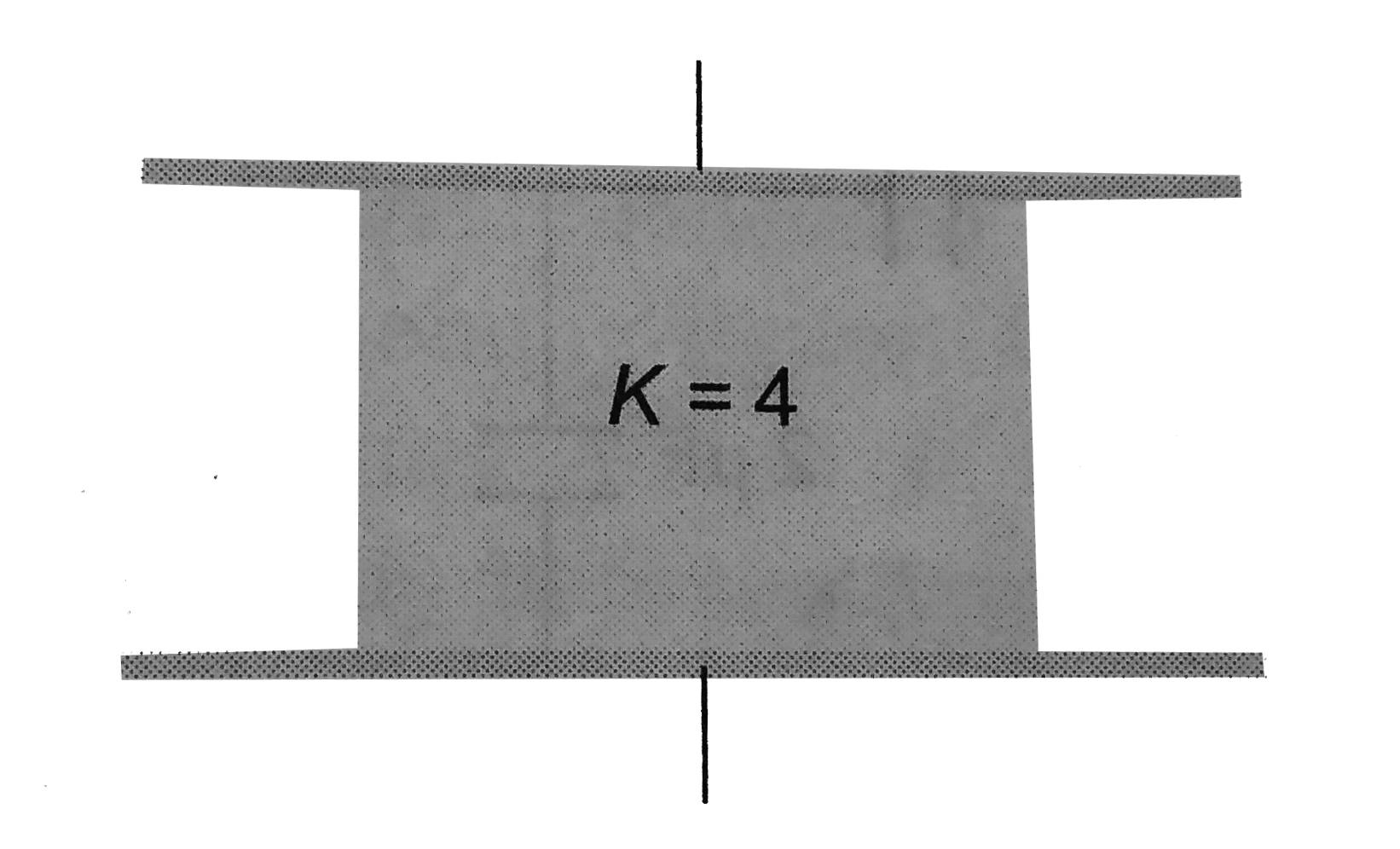 View Solution
View SolutionHalf of the space between the plates of a parallel plate capacitor is filled with a dielectric material of dielectric constant K . The ramaining half contains air as shown in the figure . The capacitor is now given a charge Q . Then
 View Solution
View SolutionConsider a disconnected. Plate capacitor of capacity 10μ with air filled in the gap between the plates. Now one-half of the space between the plates is filled with a dielectric of dielectric constant 4 as shown in .The capacity of the capacitor changes to
 .View Solution
.View Solution


